In a series LCR circuit resistance R=10Ω and the impedance Z=10Ω The phase difference between the current and the voltage is
0∘
30∘
45∘
60∘
The correct Answer is:A
To find the phase difference between the current and the voltage in a series LCR circuit where the resistance R=10Ω and the impedance Z=10Ω, we can follow these steps:
1. Understand the relationship between resistance, impedance, and phase difference:
In an LCR circuit, the phase difference ϕ between the current and voltage can be determined using the power factor, which is defined as:
Power Factor=cosϕ=RZ
2. Substitute the given values:
Here, we know:
R=10ΩandZ=10Ω
Therefore, substituting these values into the power factor equation gives:
cosϕ=1010=1
3. Determine the angle ϕ:
Since the power factor is equal to 1, we can find the phase difference ϕ by taking the inverse cosine:
ϕ=cos−1(1)
The angle whose cosine is 1 is:
ϕ=0∘
4. Conclusion:
The phase difference between the current and the voltage in the circuit is:
ϕ=0∘
Final Answer:
The phase difference between the current and the voltage is 0∘.
In an LCR circuit, the phase difference ϕ between the current and voltage can be determined using the power factor, which is defined as:
Power Factor=cosϕ=RZ
Here, we know:
R=10ΩandZ=10Ω
Therefore, substituting these values into the power factor equation gives:
cosϕ=1010=1
Since the power factor is equal to 1, we can find the phase difference ϕ by taking the inverse cosine:
ϕ=cos−1(1)
The angle whose cosine is 1 is:
ϕ=0∘
The phase difference between the current and the voltage in the circuit is:
ϕ=0∘
The phase difference between the current and the voltage is 0∘.
Similar Questions
Knowledge Check
In the series LCR circuit shown the impedance is
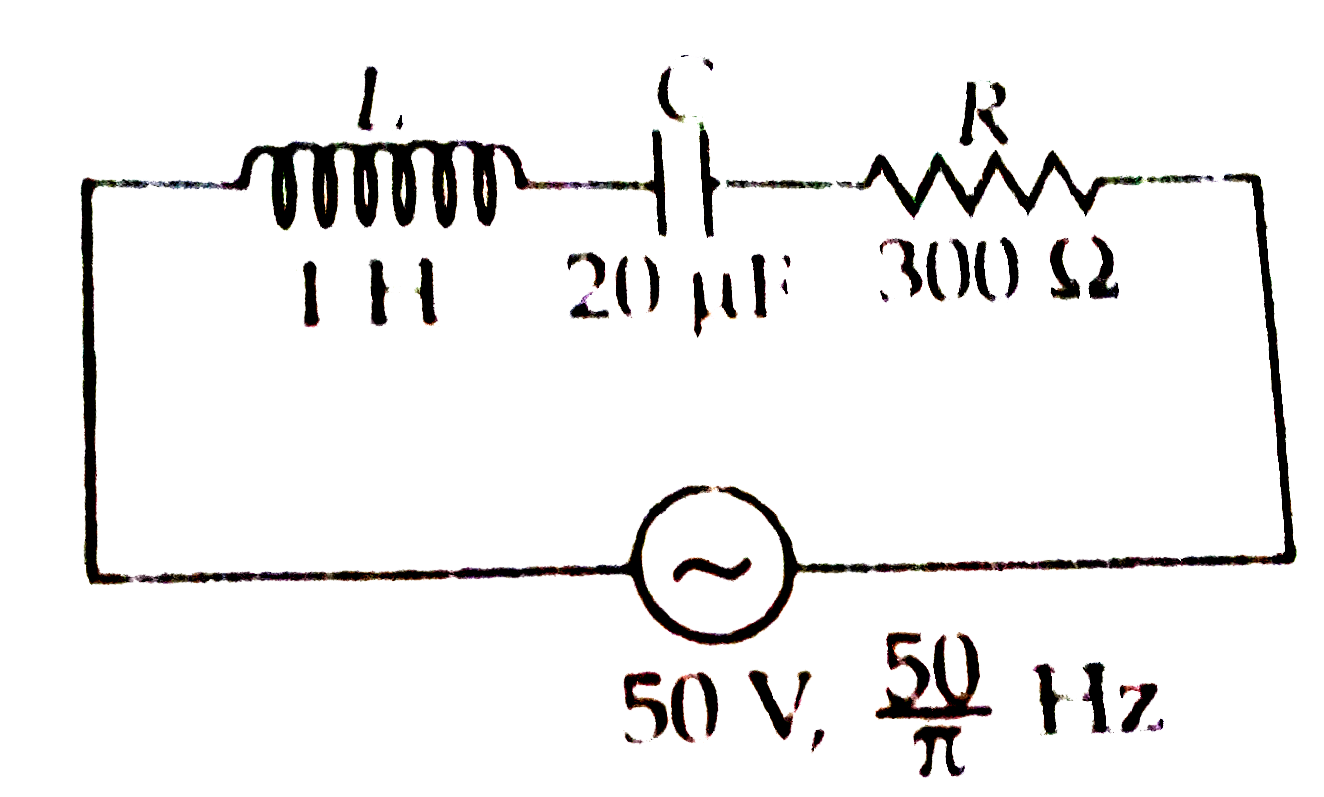 A200ΩB100ΩC300ΩD500Ω
A200ΩB100ΩC300ΩD500ΩAn alternating supply of 220 V is applied across a circuit with resistance 22Ω and impedance 44Ω. The power dissipated in the circuit is
A1100 WB550 WC2200 WD(2200/3) W
A series AC circuit has a resistance of 4Ω and a reactance of 3Ω. The impedance of the circuit is
View SolutionIn a series LCR circuit, the total reactance is 4Ω and resistance is 3Ω . Its power factor is
View SolutionIn L-R circuit, resistance is 8Ω and inductive reactance is 6Ω then impedance is:
View SolutionIn the series LCR circuit shown the impedance is
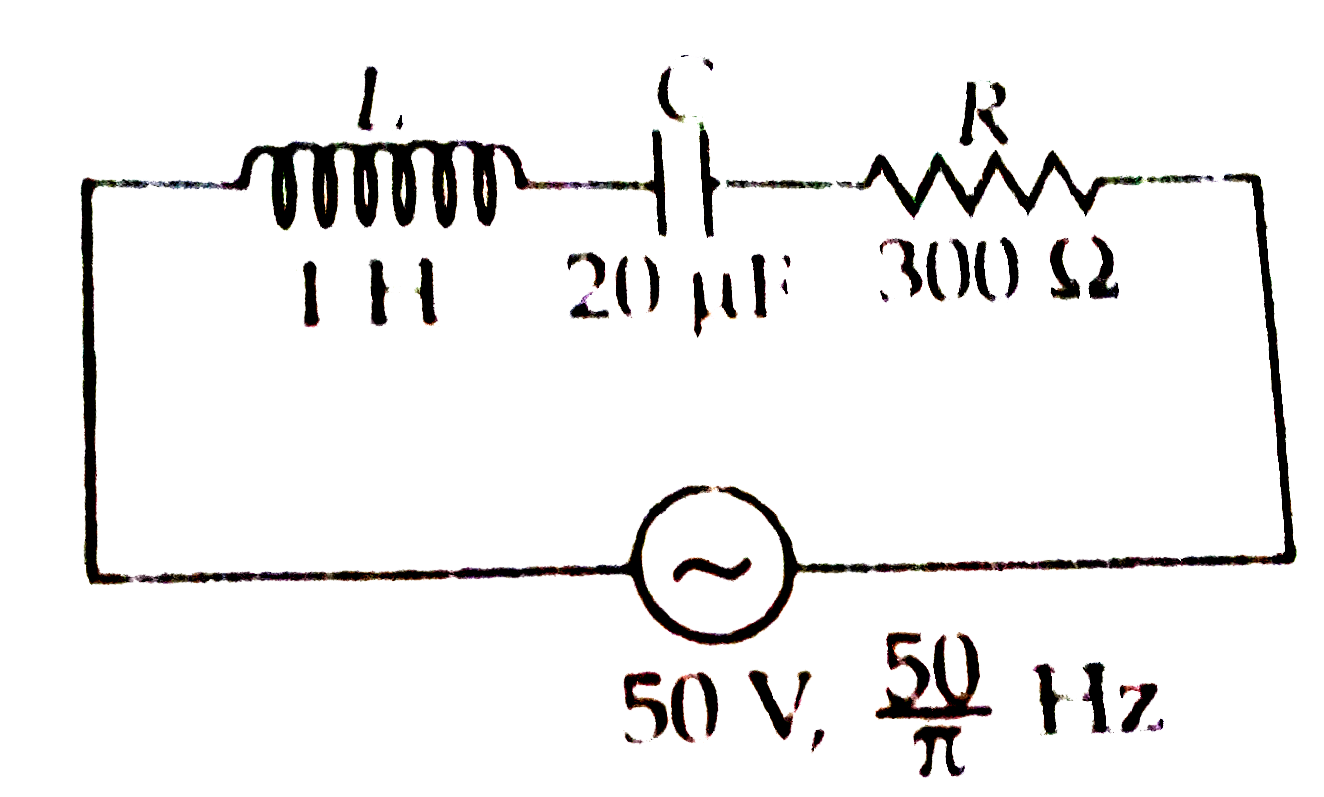
View SolutionIn series LCR circuit, the phase difference between voltage across L and voltage across C is
View SolutionAn AC voltage is applied to a resistance R and an inductance L in series. If R and the inductive reactance are both equal to 3Ω, the phase difference between the applied voltage and the current in the circuit is
View Solution
