Two solid spheres A and B made of the same material have radii rA and rB respectively . Both the spheres are cooled from the same temperature under the conditions valid for Newton's law of cooling . The ratio of the rate of change of temperature of A and B is
rArB
rBrA
r2Ar2B
r2Br2A
The correct Answer is:B
To solve the problem, we need to find the ratio of the rate of change of temperature of two solid spheres A and B, which are made of the same material and are cooled under Newton's law of cooling.
Step 1: Understand Newton's Law of Cooling
Newton's law of cooling states that the rate of change of temperature of an object is proportional to the difference between its temperature and the ambient temperature. Mathematically, it can be expressed as:
−dTdt=k(T−T0)
where T is the temperature of the object, T0 is the ambient temperature, and k is a constant that depends on the properties of the object and the medium.
Step 2: Define the Variables for Spheres A and B
Let:
- rA = radius of sphere A
- rB = radius of sphere B
- ρ = density of the material (same for both spheres)
- VA = volume of sphere A = 43πr3A
- VB = volume of sphere B = 43πr3B
- mA = mass of sphere A = ρVA=ρ43πr3A
- mB = mass of sphere B = ρVB=ρ43πr3B
Step 3: Write the Rate of Change of Temperature for Each Sphere
For sphere A:
−dTAdt=kA(TA−T0)
For sphere B:
−dTBdt=kB(TB−T0)
Step 4: Relate the Cooling Constants
The cooling constant k is proportional to the surface area and inversely proportional to the mass:
k∝Am
where A is the surface area and m is the mass.
The surface area A for a sphere is given by:
A=4πr2
Thus, for sphere A:
kA∝4πr2Aρ43πr3A=3ρrA
And for sphere B:
kB∝4πr2Bρ43πr3B=3ρrB
Step 5: Find the Ratio of the Rates of Change of Temperature
Now we can write the ratio of the rates of change of temperature:
−dTAdt−dTBdt=kA(TA−T0)kB(TB−T0)
Since both spheres are cooled from the same initial temperature and reach the same final temperature, we can simplify this to:
dTAdTB=kBkA=rBrA
Conclusion
Thus, the ratio of the rate of change of temperature of spheres A and B is:
dTAdTB=rBrA
Final Answer
The ratio of the rate of change of temperature of A and B is rBrA.
---
Newton's law of cooling states that the rate of change of temperature of an object is proportional to the difference between its temperature and the ambient temperature. Mathematically, it can be expressed as:
−dTdt=k(T−T0)
where T is the temperature of the object, T0 is the ambient temperature, and k is a constant that depends on the properties of the object and the medium.
Let:
- rA = radius of sphere A
- rB = radius of sphere B
- ρ = density of the material (same for both spheres)
- VA = volume of sphere A = 43πr3A
- VB = volume of sphere B = 43πr3B
- mA = mass of sphere A = ρVA=ρ43πr3A
- mB = mass of sphere B = ρVB=ρ43πr3B
For sphere A:
−dTAdt=kA(TA−T0)
For sphere B:
−dTBdt=kB(TB−T0)
The cooling constant k is proportional to the surface area and inversely proportional to the mass:
k∝Am
where A is the surface area and m is the mass.
A=4πr2
Thus, for sphere A:
kA∝4πr2Aρ43πr3A=3ρrA
And for sphere B:
kB∝4πr2Bρ43πr3B=3ρrB
Now we can write the ratio of the rates of change of temperature:
−dTAdt−dTBdt=kA(TA−T0)kB(TB−T0)
Since both spheres are cooled from the same initial temperature and reach the same final temperature, we can simplify this to:
dTAdTB=kBkA=rBrA
Thus, the ratio of the rate of change of temperature of spheres A and B is:
dTAdTB=rBrA
The ratio of the rate of change of temperature of A and B is rBrA.
Similar Questions
Two solid spheres of radii R1 and R2 are made of the same material and have similar surfaces. These are raised to the same temperature and then allowed to cool under identical conditions. The ratio of their initial rates of loss of heat are
View SolutionA solid sphere and a hollow sphere of the same material and of equal radii are heated to the same temperature.
View Solution
Knowledge Check
Two spheres A and B are made of the same material and have the same radius. Sphere A is hollow alnd sphere B is solid. Both the spheres are heated to the same temperature. Which of the following is correct ? a) A expands more than B. b) A expands less than B. c) Both the spheres expand equally. d) Data is insufficient
AA expands more than B.BA expands less than B.CBoth the spheres expand equally.DData is insufficientTwo rods A and B of the same material and length have radii r1 and r2respective. When they are rigidly fixed at one end and twisted by the same torque applied at the other end, the ratio [the angle of twist at the end of Athe angle of twist at the end of B] equal to
Ar21r22Br31r32Cr42r41Dr41r42
A solid sphere and a hollow sphere of the same material and of equal radii are heated to the same temperature
View SolutionThe wires A and B shown in Fig. are made of the same material and have radii rA and rB, respectively. The block between them has a mass m. When the force F is mg/3, one of the wires breaks. Then
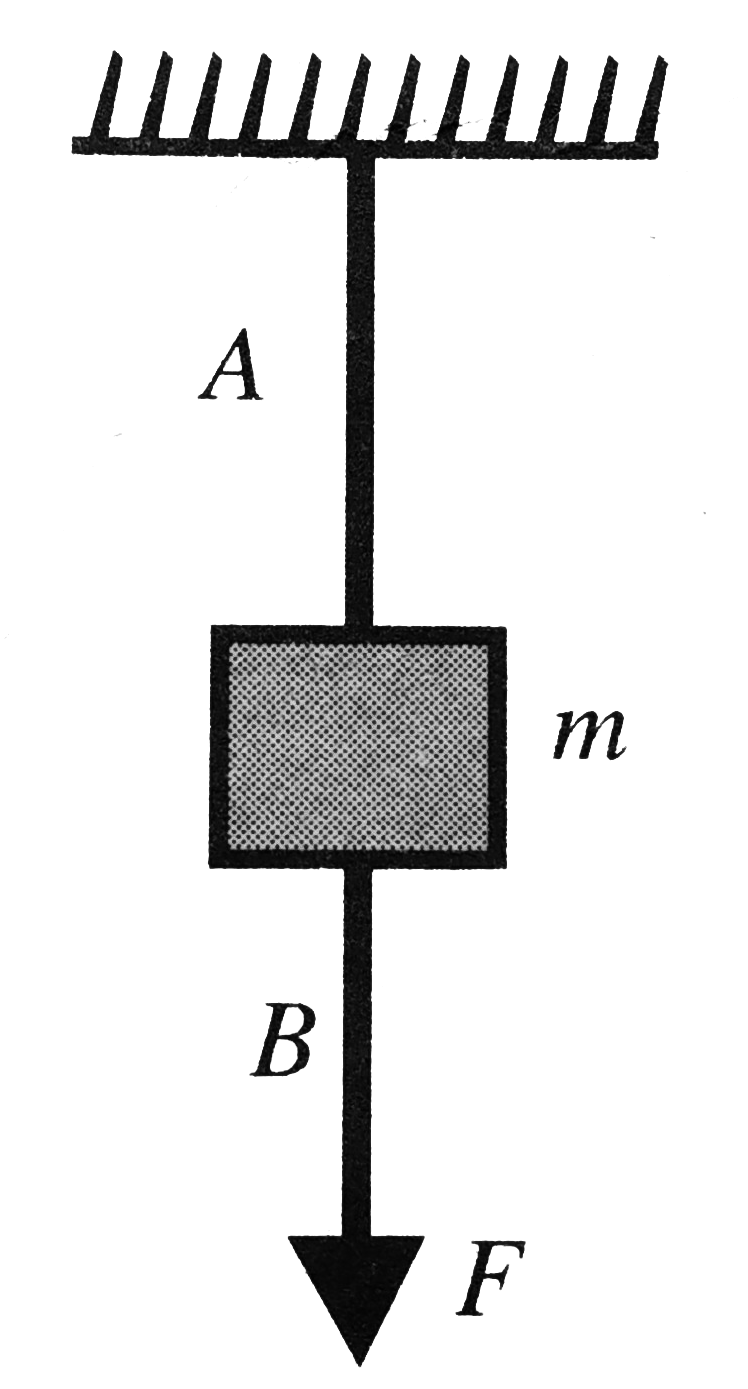
View SolutionThe wires A and B shown in Fig. are made of the same material and have radii rA and rB, respectively. The block between them has a mass m. When the force F is mg/3, one of the wires breaks. Then

View SolutionTwo metallic spheres S1andS2 are made of the same material and have got identical surface finish. The mass of S1 is thrice that of S2. Both the spheres are heated to the same high temperature and placed in the same room having lower temperature but are thermally insulated from each other. the ratio of the initial rate of cooling of S1 to that of S2 is
(a)13(b)1√3(c)√31(d)(13)13
View SolutionIf two circular discs A and B are of same mass but of radii r and 2r respectively, then the moment of inertia of A is
View SolutionTwo uniform solid spheres made of copper have radii 15 cm and 20 cm respectively. Both of them are heated to a temperature of 70∘C and then placed in a region of ambient temperature equal to 45∘C . What will be the ratio of the initial rates of cooling of both the spheres?
View Solution
