If the points A:(0,a),B:(−2,0)andC:(1,1) form an obtuse angle triangle (obtuse angled at angle A), then sum of all the possible integral values of a is
0
3
2
1
The correct Answer is:D
To solve the problem, we need to determine the conditions under which the triangle formed by the points A(0, a), B(-2, 0), and C(1, 1) has an obtuse angle at point A.
Step 1: Determine the coordinates of points B and C
The coordinates of points B and C are given as:
- B: (-2, 0)
- C: (1, 1)
Step 2: Find the lengths of the sides of the triangle
We will calculate the lengths of the sides of the triangle using the distance formula. The length of a side between two points (x1, y1) and (x2, y2) is given by:
d=√(x2−x1)2+(y2−y1)2
1. Length AB:
AB=√(0−(−2))2+(a−0)2=√(2)2+a2=√4+a2
2. Length AC:
AC=√(0−1)2+(a−1)2=√(−1)2+(a−1)2=√1+(a−1)2=√1+a2−2a+1=√a2−2a+2
3. Length BC:
BC=√(−2−1)2+(0−1)2=√(−3)2+(−1)2=√9+1=√10
Step 3: Apply the condition for an obtuse triangle
For triangle ABC to have an obtuse angle at A, the following condition must hold:
AB2+AC2<BC2
Substituting the lengths we found:
(4+a2)+(a2−2a+2)<10
Simplifying this:
4+a2+a2−2a+2<10
2a2−2a+6<10
2a2−2a−4<0
Dividing the entire inequality by 2:
a2−a−2<0
Step 4: Factor the quadratic inequality
Factoring the quadratic:
(a−2)(a+1)<0
Step 5: Determine the intervals
To find the intervals where the product is negative, we find the roots:
- a−2=0⇒a=2
- a+1=0⇒a=−1
The critical points divide the number line into intervals:
1. (−∞,−1)
2. (−1,2)
3. (2,∞)
Testing intervals:
- For a<−1 (e.g., a=−2): (−)(−)>0 (not valid)
- For −1<a<2 (e.g., a=0): (+)(−)<0 (valid)
- For a>2 (e.g., a=3): (+)(+)>0 (not valid)
Thus, the solution to the inequality is:
−1<a<2
Step 6: Identify integral values of a
The integral values of a in the interval (−1,2) are:
- 0
- 1
Step 7: Calculate the sum of integral values
The sum of all possible integral values of a is:
0+1=1
Final Answer
The sum of all possible integral values of a is 1.
---
The coordinates of points B and C are given as:
- B: (-2, 0)
- C: (1, 1)
We will calculate the lengths of the sides of the triangle using the distance formula. The length of a side between two points (x1, y1) and (x2, y2) is given by:
d=√(x2−x1)2+(y2−y1)2
AB=√(0−(−2))2+(a−0)2=√(2)2+a2=√4+a2
AC=√(0−1)2+(a−1)2=√(−1)2+(a−1)2=√1+(a−1)2=√1+a2−2a+1=√a2−2a+2
BC=√(−2−1)2+(0−1)2=√(−3)2+(−1)2=√9+1=√10
For triangle ABC to have an obtuse angle at A, the following condition must hold:
AB2+AC2<BC2
(4+a2)+(a2−2a+2)<10
Simplifying this:
4+a2+a2−2a+2<10
2a2−2a+6<10
2a2−2a−4<0
Dividing the entire inequality by 2:
a2−a−2<0
Factoring the quadratic:
(a−2)(a+1)<0
To find the intervals where the product is negative, we find the roots:
- a−2=0⇒a=2
- a+1=0⇒a=−1
1. (−∞,−1)
2. (−1,2)
3. (2,∞)
- For a<−1 (e.g., a=−2): (−)(−)>0 (not valid)
- For −1<a<2 (e.g., a=0): (+)(−)<0 (valid)
- For a>2 (e.g., a=3): (+)(+)>0 (not valid)
−1<a<2
The integral values of a in the interval (−1,2) are:
- 0
- 1
The sum of all possible integral values of a is:
0+1=1
The sum of all possible integral values of a is 1.
Similar Questions
Let the pointsA:(0,a),B:(−2,0)andC:(1,1) form an obtuse angled triangle (obtuse angled at angle A), then the complete set of values of a is
View SolutionThe points A(3,0) , B (a, -2) and C(4, -1) are the vertices of triangle ABC right angled at vertex A. Find the value of a.
View SolutionFind the ara of the given obtuse-angled triangle ABC.
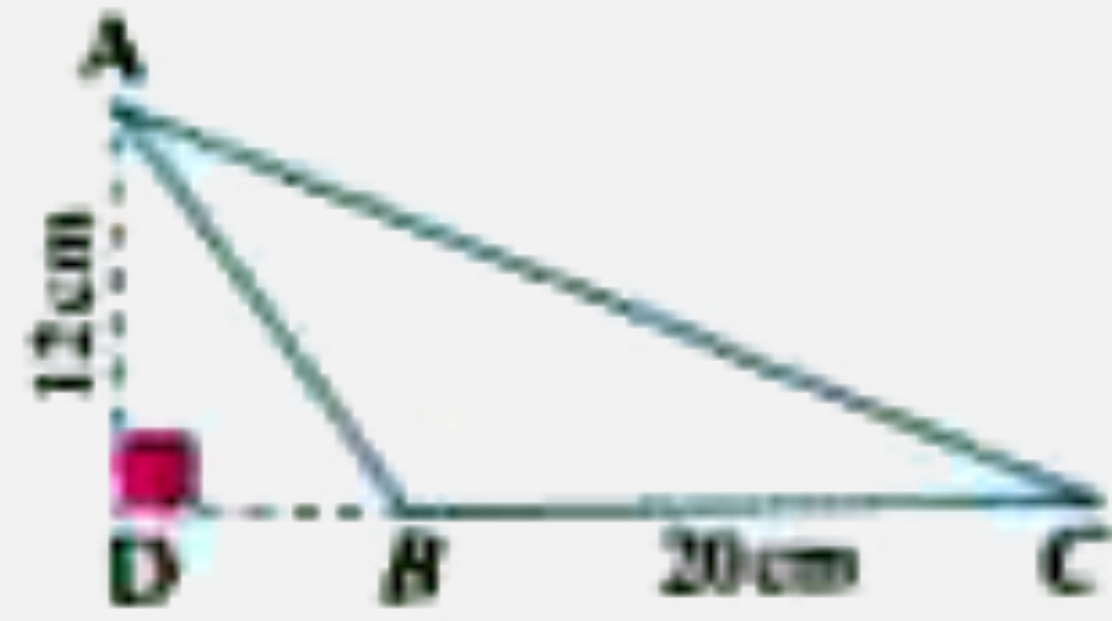
View SolutionProve that the points A(1,−3),B(−3,0)andC(4,1)are the vertices of a right angled Isosceles triangle
View SolutionProve that the points A(1, -3), B (-3, 0) and C(4, 1) are the vertices of an isosceles right- angled triangle. Find the area of the triangle.
View SolutionLet A(0,β),B(−2,0) and C(1,1) be the vertices of a triangle. Then
Angle A of the triangle ABC will be obtuse if β lies in
View SolutionWrite the sum of the angles of an obtuse triangle.
View SolutionProve that the points A (1,-3) B (-3,0) and C(4,1) are the vertices of an isosceles right angle triangle. Find the area of the triangle.
View SolutionStatement-1 : The points A(-2,2), B(2,-2) and C(1,1) are the vertices of an obtuse angled isoscles triangle.
Statement-2: Every obtuse angle triangle is isosceles.
View SolutionA median and an altitude of an obtuse-angled triangle lie outside the triangle. It is true ?
View Solution
