What is the activation energy for the decomposition of N2O5 as
N2O5⇔2NO2+12O2
If the values of the rate constants are 3.45×10−5 and 6.9×10−3 at 27∘C and 67∘C respectively
112.3 kJ mol−1
200.55 kJ mol−1
149.5 kJ mol−1
11.25 kJ mol−1
The correct Answer is:A
To find the activation energy (Ea) for the decomposition of N2O5 using the Arrhenius equation, we can follow these steps:
Step 1: Write the Arrhenius equation in logarithmic form
The Arrhenius equation relates the rate constants (k) at two different temperatures (T) to the activation energy (Ea):
ln(k2k1)=−EaR(1T2−1T1)
However, we can also use the logarithmic form:
log(k2k1)=Ea2.303R(T2−T1)1T1T2
Step 2: Identify the given values
From the problem statement, we have:
- k1=3.45×10−5 at T1=27∘C=300K
- k2=6.9×10−3 at T2=67∘C=340K
- The gas constant R=8.314J/mol⋅K
Step 3: Substitute the values into the equation
We can rearrange the equation to solve for Ea:
Ea=2.303R(T2−T1)log(k2k1)⋅1T1T2
Step 4: Calculate log(k2k1)
First, calculate k2k1:
k2k1=6.9×10−33.45×10−5=200
Now calculate the logarithm:
log(200)≈2.301
Step 5: Calculate T2−T1
T2−T1=340K−300K=40K
Step 6: Substitute everything into the equation
Now substituting all the values into the equation for Ea:
Ea=2.303×8.314×402.301
Calculating the numerator:
2.303×8.314×40≈766.8
Now divide by 2.301:
Ea≈766.82.301≈333.5J/mol
Step 7: Convert to kJ/mol
To convert to kJ/mol:
Ea≈0.3335kJ/mol≈1.13kJ/mol
Final Answer
Thus, the activation energy for the decomposition of N2O5 is approximately:
Ea≈112.63kJ/mol
The Arrhenius equation relates the rate constants (k) at two different temperatures (T) to the activation energy (Ea):
ln(k2k1)=−EaR(1T2−1T1)
However, we can also use the logarithmic form:
log(k2k1)=Ea2.303R(T2−T1)1T1T2
From the problem statement, we have:
- k1=3.45×10−5 at T1=27∘C=300K
- k2=6.9×10−3 at T2=67∘C=340K
- The gas constant R=8.314J/mol⋅K
We can rearrange the equation to solve for Ea:
Ea=2.303R(T2−T1)log(k2k1)⋅1T1T2
First, calculate k2k1:
k2k1=6.9×10−33.45×10−5=200
Now calculate the logarithm:
log(200)≈2.301
T2−T1=340K−300K=40K
Now substituting all the values into the equation for Ea:
Ea=2.303×8.314×402.301
Calculating the numerator:
2.303×8.314×40≈766.8
Now divide by 2.301:
Ea≈766.82.301≈333.5J/mol
To convert to kJ/mol:
Ea≈0.3335kJ/mol≈1.13kJ/mol
Thus, the activation energy for the decomposition of N2O5 is approximately:
Ea≈112.63kJ/mol
Similar Questions
The rate constant of a reactant is 1.5×10−3 at 25∘C and 2.1×10−2 at 60∘C. The activation energy is
View SolutionThe first order rate constant for the decomposition of N2O5 is 6.2×10−4sec−1. The t1/2 of decomposition is
View SolutionWrite the oxyacids of the following oxides: N2O3,N2O5,N2O4
View SolutionFor the reaction N2O5→2NO2+12O2, the rate of disappearance of N2O5 is 6.25×10−3mol L−1s−1. The rate of formation of NO2 and O2 will be respectively.
View SolutionThe rate law for the decomposition of N2O5 is: rate =[N2O5]. What is the significance of k in this equation?
View SolutionThe rate law for the decomposition of N2O5 is: rate =[N2O5]. What is the significance of k in this equation?
View SolutionThe rate law for the decomposition of N2O5 is: rate =[N2O5]. What is the significance of k in this equation?
View SolutionAt 250∘C , the half life for the decomposition of N2O5 is 5.7 hour and is independent of initial pressure of N2O5. The specific rate constant is
View SolutionThe following data were obtained during the first order thermal decomposition of N2O5 (g) at constant volume :
2N2O5(g)→2N2O4(g)+O2(g)
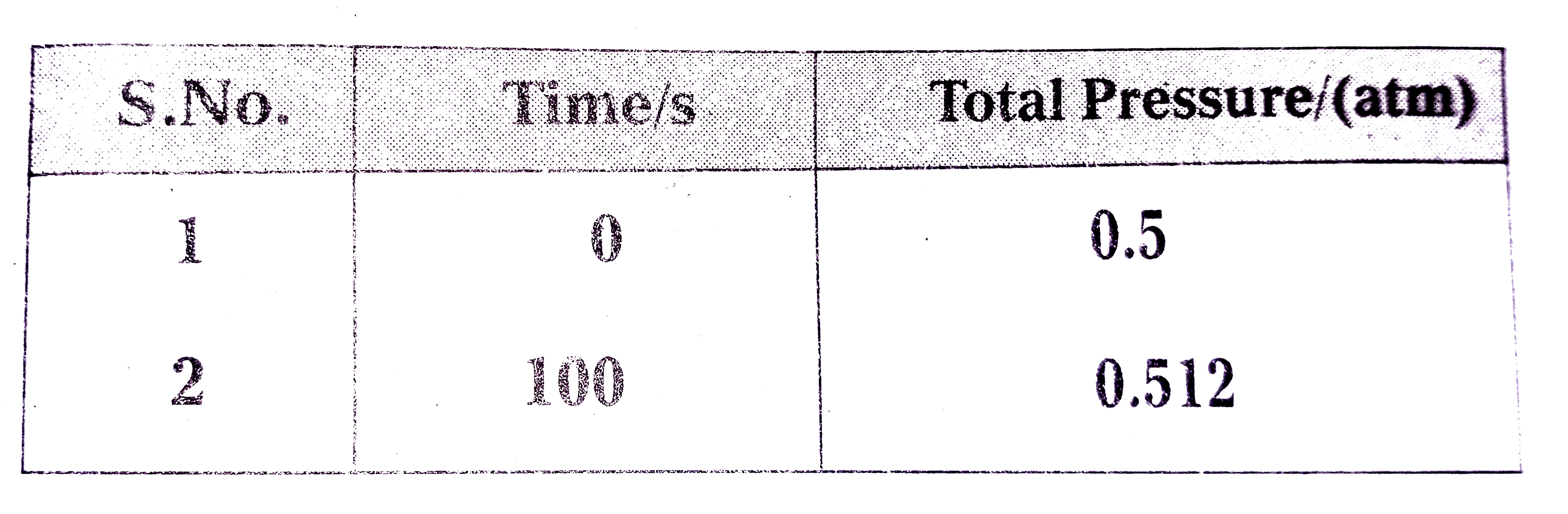
Calculate the rate constant.
View SolutionFor the reaction 2N2O5→4NO2+O2 rate of reaction and rate constant are 1.04×10−4and3.4×10−5sec−1 respectively. The concentration of N2O5 at that time will be :
View Solution
