In Young's double-slit experiment, the intensity at a point P on the screen is half the maximum intensity in the interference pattern. If the wavelength of light used is λ and d is the distance between the slits, the angular separation between point P and the center of the screen is
sin−1(λd)
sin−1(λ2d)
sin−1(λ3d)
sin−1(λ4d)
The correct Answer is:D
To solve the problem step by step, we will analyze the situation in Young's double-slit experiment where the intensity at point P on the screen is half the maximum intensity.
Step 1: Understand the relationship between intensity and phase difference
In Young's double-slit experiment, the intensity I at a point on the screen can be expressed in terms of the maximum intensity I0 and the phase difference ϕ as follows:
I=I0cos2(ϕ2)
Given that the intensity at point P is half the maximum intensity:
I=I02
Step 2: Set up the equation
Substituting the expression for intensity into the equation gives:
I02=I0cos2(ϕ2)
Dividing both sides by I0 (assuming I0≠0):
12=cos2(ϕ2)
Step 3: Solve for the phase difference ϕ
Taking the square root of both sides:
cos(ϕ2)=1√2
This implies:
ϕ2=π4orϕ2=3π4
Thus, we can find ϕ:
ϕ=π2orϕ=3π2
Since we are interested in the first order maximum, we take:
ϕ=π2
Step 4: Relate phase difference to path difference
The phase difference ϕ can also be expressed in terms of the path difference Δx as:
ϕ=2πλΔx
Setting this equal to our earlier result:
π2=2πλΔx
Step 5: Solve for the path difference Δx
Rearranging gives:
Δx=λ4
Step 6: Relate path difference to angular separation
In the double-slit experiment, the path difference Δx can also be expressed in terms of the angle θ and the slit separation d:
Δx=dsinθ
Setting this equal to our expression for Δx:
dsinθ=λ4
Step 7: Solve for sinθ
Rearranging gives:
sinθ=λ4d
Step 8: Find the angle θ
Finally, we can find the angle θ:
θ=sin−1(λ4d)
Final Answer
The angular separation between point P and the center of the screen is:
θ=sin−1(λ4d)
In Young's double-slit experiment, the intensity I at a point on the screen can be expressed in terms of the maximum intensity I0 and the phase difference ϕ as follows:
I=I0cos2(ϕ2)
Given that the intensity at point P is half the maximum intensity:
I=I02
Substituting the expression for intensity into the equation gives:
I02=I0cos2(ϕ2)
Dividing both sides by I0 (assuming I0≠0):
12=cos2(ϕ2)
Taking the square root of both sides:
cos(ϕ2)=1√2
This implies:
ϕ2=π4orϕ2=3π4
Thus, we can find ϕ:
ϕ=π2orϕ=3π2
Since we are interested in the first order maximum, we take:
ϕ=π2
The phase difference ϕ can also be expressed in terms of the path difference Δx as:
ϕ=2πλΔx
Setting this equal to our earlier result:
π2=2πλΔx
Rearranging gives:
Δx=λ4
In the double-slit experiment, the path difference Δx can also be expressed in terms of the angle θ and the slit separation d:
Δx=dsinθ
Setting this equal to our expression for Δx:
dsinθ=λ4
Rearranging gives:
sinθ=λ4d
Finally, we can find the angle θ:
θ=sin−1(λ4d)
The angular separation between point P and the center of the screen is:
θ=sin−1(λ4d)
Similar Questions
Knowledge Check
In Young's double slit experiment, the slits are horizontal. The intensity at a point P as shown in figure is 34I0,whereI0 is the maximum intensity.
Then the value of θ is,
(Given the distance between the two slits S1 and S2 is 2λ)
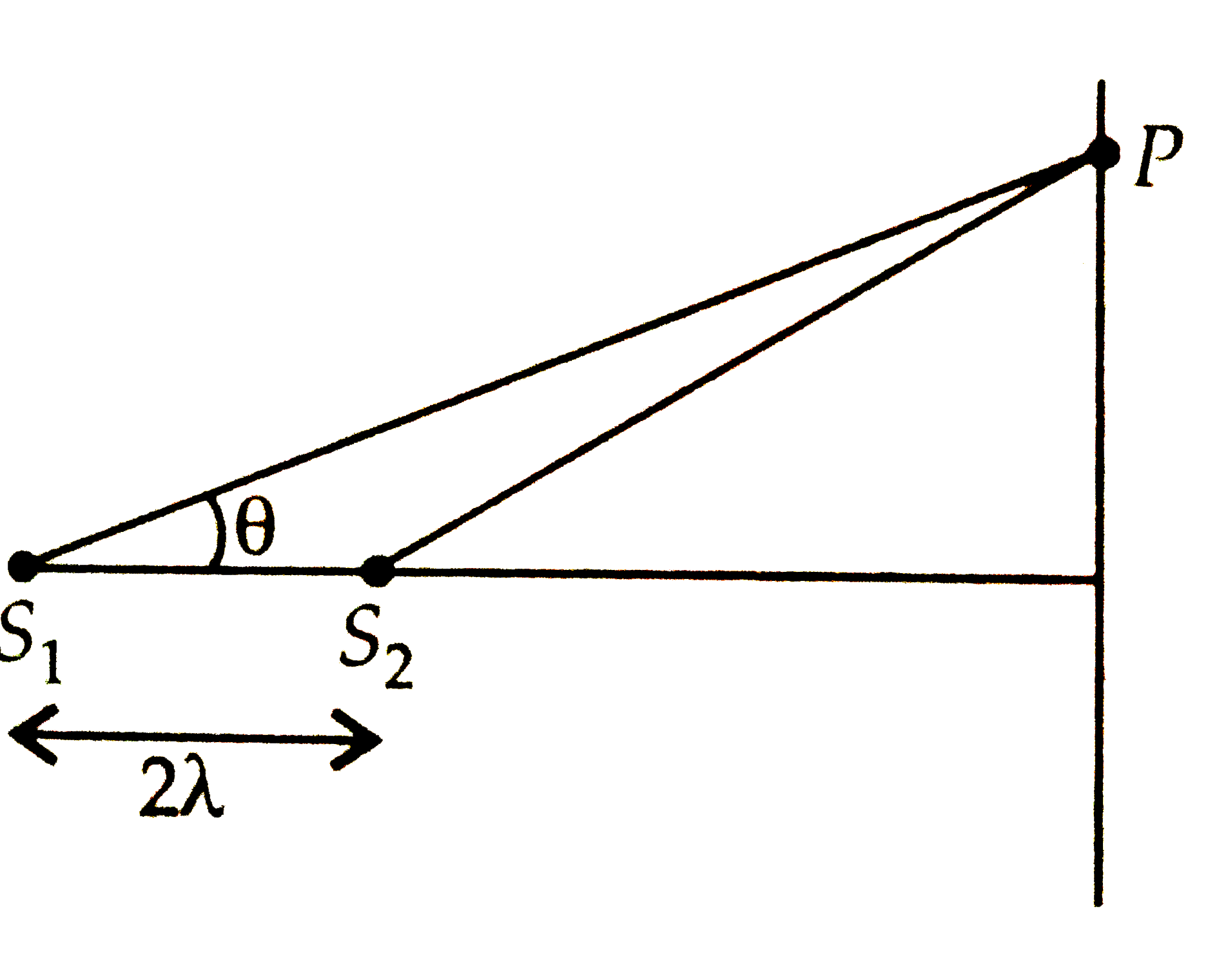 Acos−1(112)Bsin−1(112)Ctan−1(112)Dsin−1(35)
Acos−1(112)Bsin−1(112)Ctan−1(112)Dsin−1(35)
In Young's double - slit experiment intensity at a point is (1/4)th of the maximum intensity. The possible angular position of this point is
View SolutionIn the Young's double slit experiment apparatus shown in figure, the ratio of maximum to minimum intensity on the screen is 9. The wavelength of light used is λ, then the value of y is

View SolutionIn the Young's double-slit experiment, the intensity of light at a point on the screen where the path difference is λ is K, (λ being the wavelength of light used). The intensity at a point where the path difference is λ/4 will be
View SolutionIn Young's double slit experiment, the intensity on the screen at a point where path difference is λ is K. What will be the intensity at the point where path difference is λ/4?
View SolutionIn Young's double-slit experimetn, the intensity of light at a point on the screen, where the path difference is λ,is I . The intensity of light at a point where the path difference becomes λ/3 is
View SolutionIn the Young's double slit experiment, the intensity of light at a point on the screen where the path difference is λ is K, (λ being the wave length of light used). The intensity at a point where the path difference is λ/4, will be
View SolutionIn Young's double slit experiment, the intensity of light at a point on the screen where path difference is λ is I. If intensity at another point is I/4, then possible path differences at this point are
View Solution
