In the figure, AB is a chord with centre O, AOC is the diameter and AT is a tangent touching the circle at A. Prove that ∠BAT = ∠ACB.
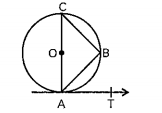
Step by step video & image solution for In the figure, AB is a chord with centre O, AOC is the diameter and AT is a tangent touching the circle at A. Prove that angle BAT = angle ACB. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
Similar Questions
If AB is chord of a circle with centre O, AOC is a diameter and AT is the tangent at A as shown in figure. Prove that ∠BAT=∠ACB.
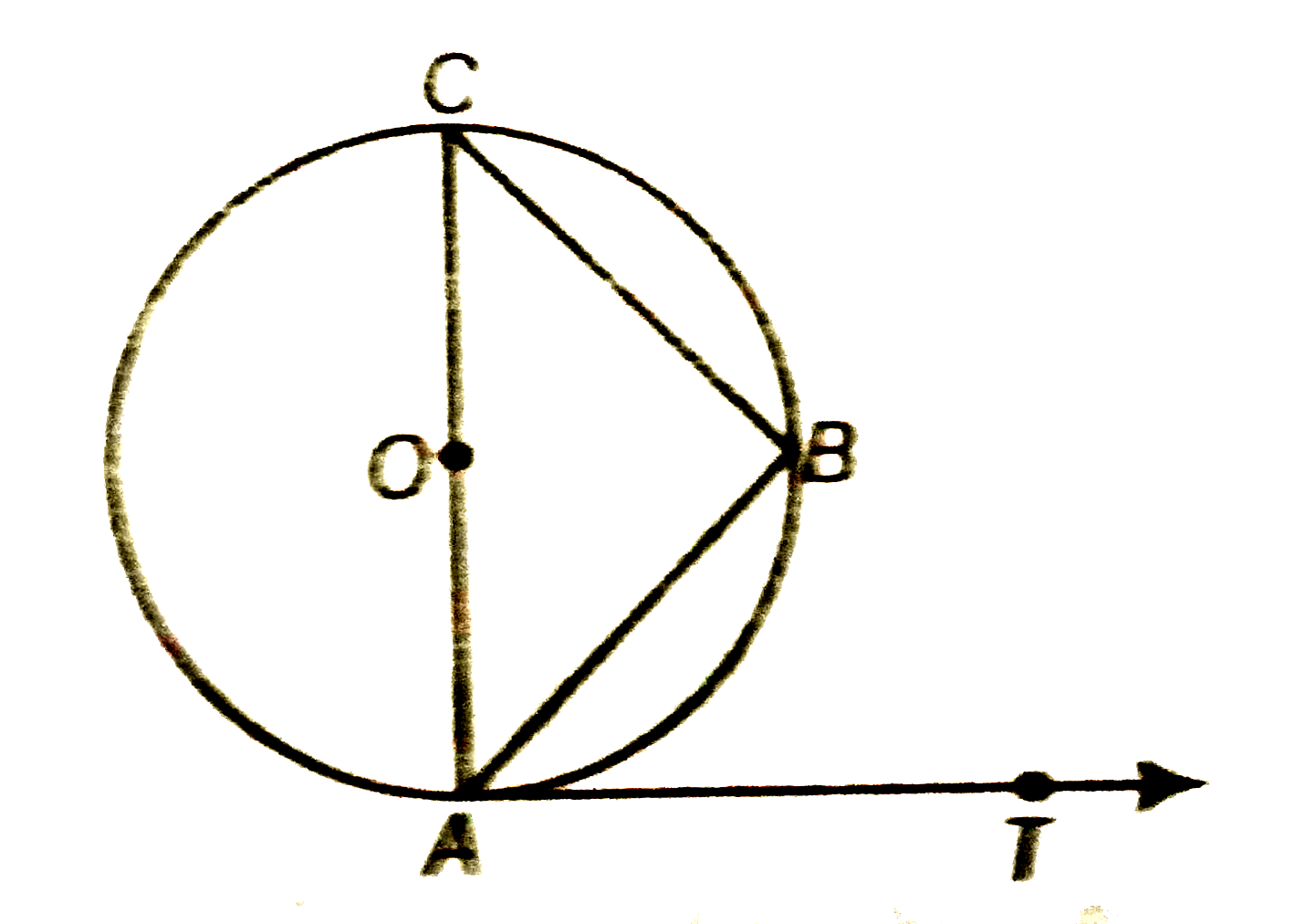 View Solution
View SolutionIn figure, AB is a chord of the circle and AOC is the diameter such that ∠ACB=50∘. If AT is the tangent to the circle at the point A, then ∠BAT is equal to : (a) 45∘ , (b) 60∘ , (c) 50∘ , (d) 55∘
View Solution
Knowledge Check
- Question 1 - Select One
In the given figure, AB is a chord of the circle with centre O and PQ is a tangent at point B of the circle. If ∠AOB=110∘, then ∠ ABQ is
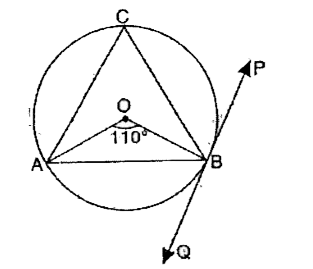 A45∘B70∘C55∘D35∘
A45∘B70∘C55∘D35∘ - Question 2 - Select One
In figure, AB is a chord of the circle and AOC is its diameter such that ∠ACB=50∘. If AT is the tangent to the circle at the point A, then ∠BAT is equal to
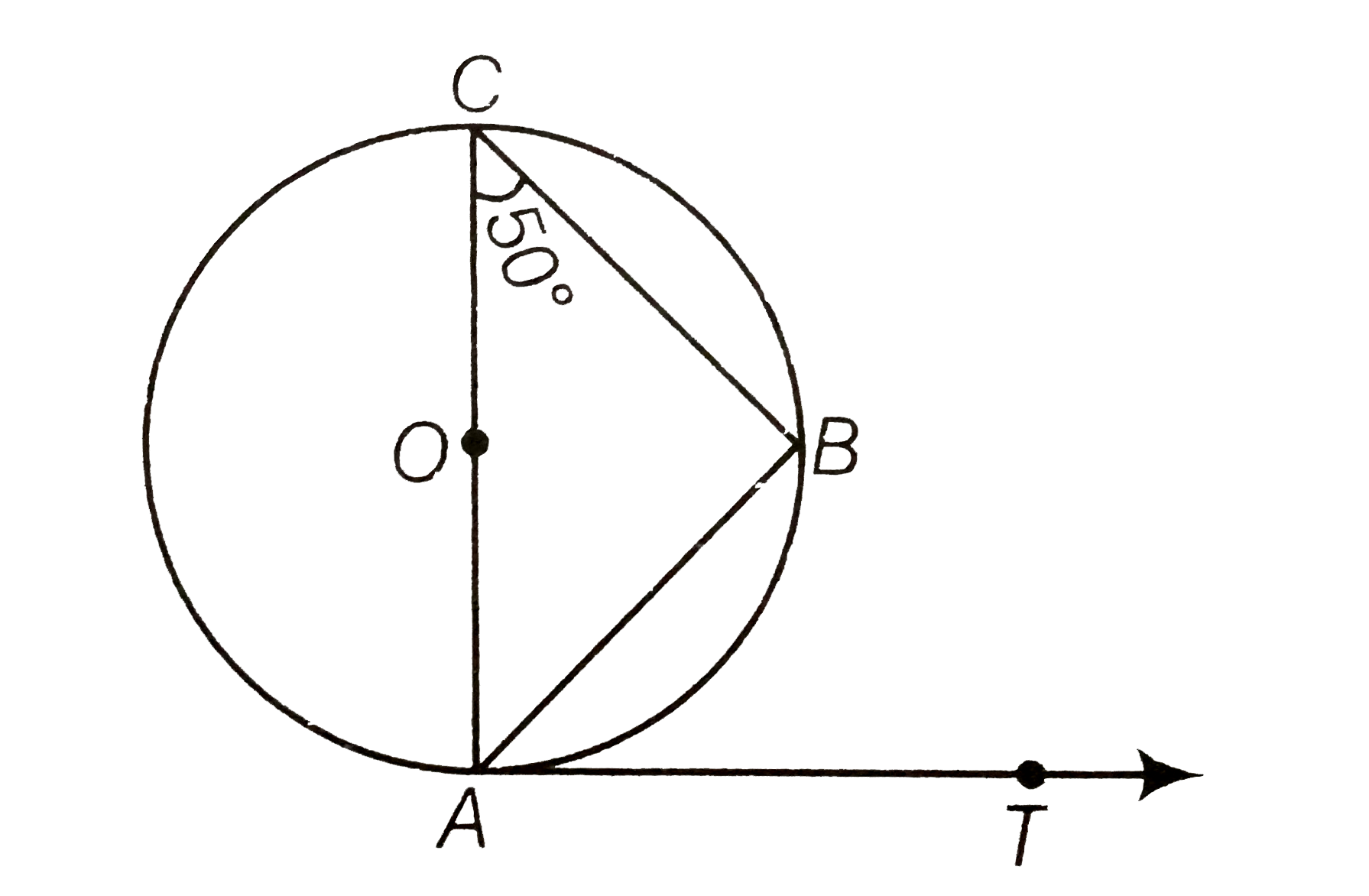 A45∘B60∘C50∘D55∘
A45∘B60∘C50∘D55∘ - Question 3 - Select One
In the figure, O is the centre of a circle and AT is a tangent at point A. The measure of ∠BAT is:
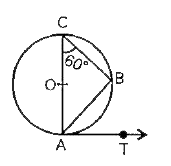 A30∘B60∘C75∘D105∘
A30∘B60∘C75∘D105∘
In the figure, O is the centre of the circle and LN is a diameter. If PQ is a tangent to the circle at K and ∠KLN=30∘, find ∠PKL.
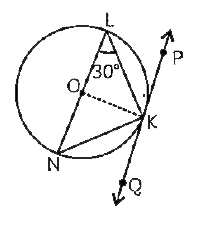 View Solution
View SolutionIn the given figure A,B is a chord of the circle and AOC is its diameter ,such that ∠ACB=50∘ .If AT is the tangent to the circle at the point A, then ∠BAT is equal to :
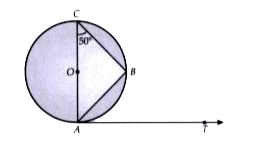 View Solution
View SolutionIn the given figure, AB is a tangent to a circle with centre O. Prove ∠BPQ=∠PRQ.
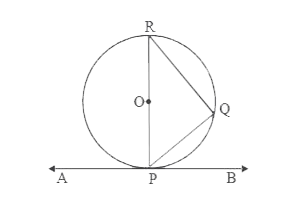 View Solution
View SolutionIn a circel with centre O. chords AB and CD interest inside the circumference at E. Prove that ∠AOC+BOD=2∠AEC.
View SolutionIn the adjoning figure O is the centre of the circle and AB is the diameter. Tangent PQ touches the circle at D. ∠BDQ=48∘. Find the ratio of angleDBA:angleDCB.
 View Solution
View Solution

