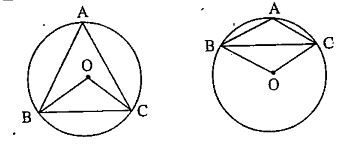
ABC ত্রিভুজের পরিকেন্দ্র O, প্রমাণ করো যে, ∠OBC+∠BAC=90∘
Step by step video & image solution for ABC ত্রিভুজের পরিকেন্দ্র O, প্রমাণ করো যে, /_OBC+/_BAC=90^@ by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
যদি বিচ্যুতি di=(ξ−22), 22= কাল্পনিক গড়, ∑f=500 এবং ∑fi=100 হয়, তাহলে যৌগিক গড ¯x এর মান—
View Solutionদুই বন্ধু যথাক্রমে 40,000 টাকা ও 50,000 টাকা দিয়ে একটি যৌথ ব্যাবসা শুরু করেন । তাদের মধ্যে চুক্তি হয় যে, লাভের 50% নিজেদের মধ্যে সমান ভাগে এবং লাভের অবশিষ্টাংশ মূলধনের অনুপাতে ভাগ হবে । প্রথম বন্ধুর লভ্যাংশের পরিমাণ যদি দ্বিতীয় বন্ধুর লভ্যাংশ অপেক্ষা 800 টাকা কম হয়, তবে প্রথম বন্ধুর লভ্যাংশের পরিমাণ নির্ণয় করো ।
View SolutionBC is a chord with centre O. A is a point on an arc BC . Prove that: ∠BAC+∠OBC=90 , if A is the point on the major arc.
View SolutionBC is a chord with centre O. A is a point on an arc BC . Prove that: ∠BAC−∠OBC=90∘ , if A is the point on the minor arc
View SolutionIf O is the circumcentre of ABC, then find the value of ∠OBC+ ∠BAC
View SolutionLet O be a point inside a triangle ABC such that /_OAB=/_OBC=/_OCA=omega, then Show that:
View SolutionIf lengths of the sides of a triangle ABC are 4cm,5cm and 6cm,O is point inside the triangle ABC such that /_OBC=/_OCA=/_OAB=theta then value of cot theta is
View Solutionযদি |z1|=|z2|এবংarg z1 + arg z2 = 0হয়,তবেপ্রমাণকরোz1 = 1/(z2)।
View Solutionযদি |z1|=|z2|এবংarg z1 ~ arg z2 = piহয়,তবেপ্রমাণকরোz1 + z2 = 0।
View SolutionIn the given figure, ABC is a right angled triangle with ∠BAC=90∘.
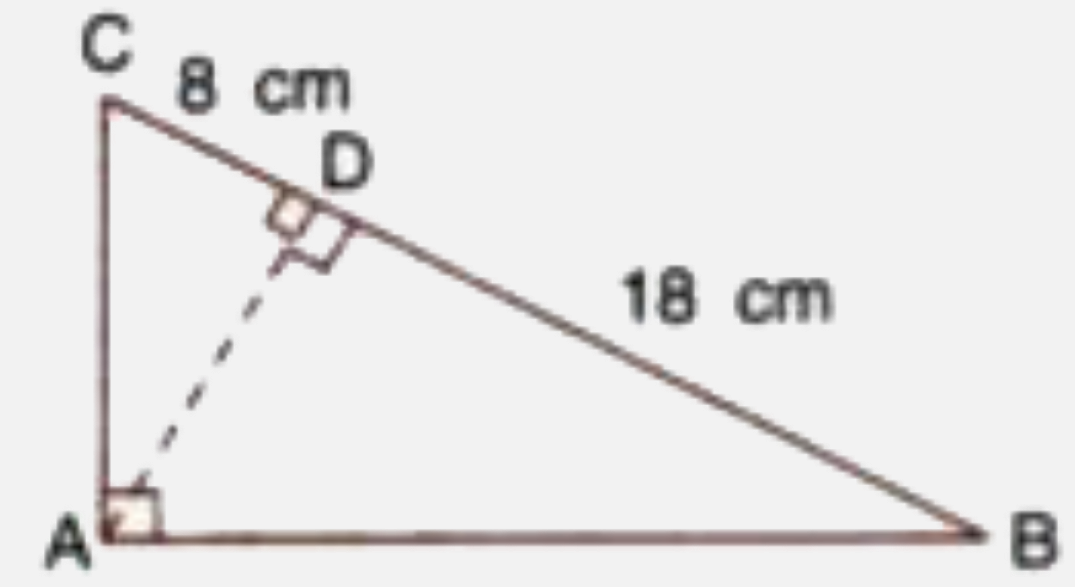
Prove that : ΔADB~ΔCDA.
View SolutionIn the given figure, ABC is a right angled triangle with ∠BAC=90∘.

Find the ratio of the area of DetlaADB is to area of ΔCDA.
View Solution/_\ABC Of AB = 1/2BC, /_BAC = 90^@ If, /_ACB What is its value?
View Solutionযদি '(logx)/(b-c)=logy/(c-a)=logz/(a-b) হয়, তবে প্রমাণ করো যে, x^(b^2+bc+c^2)*y^(c^2+ac+a^2)*z^(a^2+ab+b^2)=1'
View Solution△ABC এর অন্তর্বৃত্তের কেন্দ্র O, ∠OBC=25∘ও ∠OCB=35∘ হলে, ∠BACএ্রর মান কত?
View SolutionO কেন্দ্রীয় বৃত্তের BC হলো জ্যা।A বৃত্তের পরিধির উপরিস্থিত বিন্দু। প্রমাণ করো,∠BAC+∠OBC=90∘এবং ∠BAC–∠OBC=90∘ |
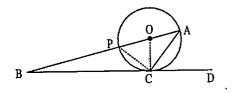
View SolutionO কেন্দ্রীয় বৃত্তের BCD হলো স্পর্শক। প্রমাণ করো, ∠BAC+ACD=90∘
View Solution
