In two triangles, if the angles are equal, then the sides opposite to the equal angles are in the same ratio (or proportional) and hence the two triangles are similar.Solution in Telugu
Step by step video & image solution for In two triangles, if the angles are equal, then the sides opposite to the equal angles are in the same ratio (or proportional) and hence the two triangles are similar. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In △ABC, DE∣∣BC and AD=13BD.
If BC = 4.5cm, Find DE.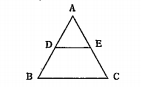
View SolutionWhich of the following are sets? Justify your answer.
The collection of all even integers.
View SolutionThe median of the data x/5, x, x/4, x/2 and x/8 is 8. Find the value of x.
View SolutionAngles opposite to two equal sides of a triangle are equal.
View SolutionSAS Similarity Criterion : If in two triangle; one pair of corresponding sides are proportional and the included angles are equal then two triangles are similar.
View SolutionIf one angle of a triangle is equal to one angle of another triangle and bisector of these equal angles divide the opposite side in the same ratio; prove that the triangles are similar.
View SolutionFill in the blanks using the correct word given in brackets: Two triangles are similar, if their corresponding angles are ...... (proportional, equal) Two triangles are similar, if their corresponding sides are ....... (proportional, equal) (iii) Two polygons of the same number of sides are similar, if (a) their corresponding angles are and (b) their corresponding sides are ........ (equal, proportional).
View SolutionOne angle of a triangle is equal to one angle of another triangle and the bisectors of these two equal angles divide the opposite sides in the same ratio, prove that the triangles are similar.
View SolutionTheorem 6.3 : If in two triangles, corresponding angles are equal, then their corresponding sides are in the same ratio (or proportion) and hence the two triangles are similar.
View SolutionTheorem 6.4 : If in two triangles, sides of one triangle are proportional to (i.e., in the same ratio of ) the sides of the other triangle, then their corresponding angles are equal and hence the two triangles are similiar
View SolutionIF one angle of a triangle is equal to one angle of another triangle and the sides including these angles are proportional, the two triangles are similar. This property is……….
View Solutionयदि किसी त्रिभुज के दो कोण समान हों तो उनकी सम्मुख भुजाएँ समान होती हैं।
View SolutionIf the corresponding angles in the two triangles are equal, then their corresponding sides are in the same proportion (proportional) and hence they are congruent. Prove it
View SolutionTheorem 6.3 : If in two triangles, corresponding angles are equal, then their corresponding sides are in the same ratio (or proportion) and hence the two triangles are similar.
View SolutionTheorem 6.4 : If in two triangles, sides of one triangle are proportional to (i.e., in the same ratio of ) the sides of the other triangle, then their corresponding angles are equal and hence the two triangles are similiar
View SolutionFill in the blanks using the correct word given in brackets: Two triangles are similar, if their corresponding angles are ...... (proportional, equal) Two triangles are similar, if their corresponding sides are ....... (proportional, equal) (iii) Two polygons of the same number of sides are similar, if (a) their corresponding angles are and (b) their corresponding sides are ........ (equal, proportional).
View Solution
