The radius of a cylinder and a hemisphere is equal and their volumes are equal. The height of the hemisphere is greater than the height of the cylinder by.Solution in Bengali
0.75
0.5
0.3
0.25
Step by step video & image solution for The radius of a cylinder and a hemisphere is equal and their volumes are equal. The height of the hemisphere is greater than the height of the cylinder by. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Multiple Choice Questions (MCQ) If r, 8, 16, q are in continued proportion, then the value of (p + q) is
View SolutionMultiple Choice Questions(MCQ) The ratio of the volume of two cubes is 8 : 125, the ratio of total surface areas of two cubes is
View SolutionThe radii of the bases as well as the heights of a cone and a cylinder are each equal to h and the radius of a hemisphere is also equal to h. Find the ratio of the volume of cylinder, hemisphere and cone.
View SolutionThe radius and height of a cylinder are equal. If the radius of the sphere is equal to the height of the cylinder, then the ratio of the rates of increase of the volume of the sphere and the volume of the cylinder, is
View Solutionसमान ऊँचाई के एक बेलन और एक अर्धगोले के आधार समान हैं । उनके आयतनों का अनुपात है
View SolutionA cylinder, a cone and a hemisphere are of equal base and have the same height, then the ratio of their volumes is
View SolutionRatio of volumes of a cone, a cylinder and a hemisphere of same base, radius and equal heights is ………..
View SolutionA cone, a hemisphere and a cylinder have equal bases. The heights of the cone and cylinder are equal and are same as the common radius. Are they equal in volume?
View SolutionRatio of volumes of a cylinder and a hemisphere of equal radius and heights is ………..
View SolutionA cylinder, a cone and a hemisphere have equal base radius and height. Find the ratio of their volumes
View SolutionRadius of a cylinder is equal to its height. If the radius is taken as r, volume of the cylinder is πr2×r=πr3. Like this find the volume of the solids, with the following measures.What is the ratio of the volume of cone, hemisphere, cylinder and the sphere?
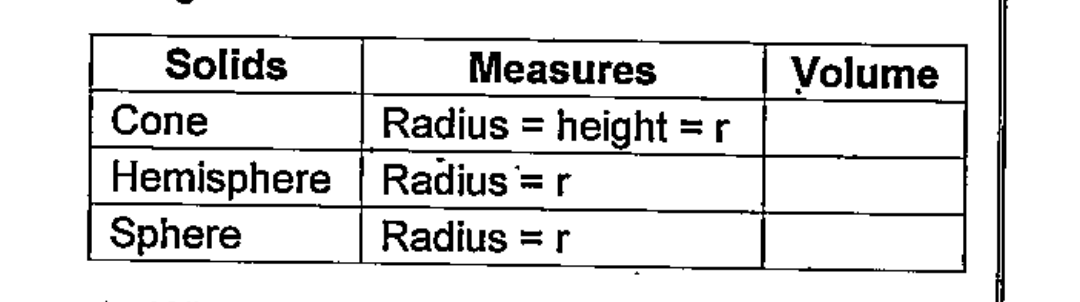 .
.
View SolutionThe radius and height of a cylinder are equal. If the radius of the sphere is equal to the height of the cylinder, then the ratio of the rates of increase of the volume of the sphere and the volume of the cylinder, is
View SolutionIf the radius of a cylinder and hemisphere is same and their height is also equal then the ratio of their volume is
View SolutionIf the base radius and height of solid hemisphere and a solid right circular cylinder are equal, then the ratio of their volume is
View SolutionThe radii of the base as well as the heights of a cone and a cylinder are each equal to h and the radius of a hemisphere is also equal to h . Find the ratio of the volume of cylinder , hemisphere , and cone .
View Solution
