A conical cap is filled with ice cream. The ice cream forms a hemi-spherical shape on its open top. The height of the hemispherical part is 7 cm, The radius of the hemispherical part equals the height of the come. Then the volume of ice-cream is____Solution in Bengali
1078 cu cm
1708 cu. Cm
7108 cu. Cm
7180 cu. Cm
Step by step video & image solution for A conical cap is filled with ice cream. The ice cream forms a hemi-spherical shape on its open top. The height of the hemispherical part is 7 cm, The radius of the hemispherical part equals the height of the come. Then the volume of ice-cream is____ by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Multiple Choice Questions (MCQ) If r, 8, 16, q are in continued proportion, then the value of (p + q) is
View SolutionMultiple Choice Questions(MCQ) The ratio of the volume of two cubes is 8 : 125, the ratio of total surface areas of two cubes is
View SolutionA conical cup when filled with ice-cream forms a hemispherical shape on its open end. Findthe volume of the ice-cream,if the radius of the base of the cone is 3.5cm and the verticalheight of the cone is 7cm.
View SolutionA cylindrical container of radius 6 cm and height 15 cm is filled with ice-cream. The whole ice-cream has to be distributed to 10 children in equal cones with hemispherical tops. If the height of the conical portion is four times the radius of its base, find the radius of the ice-cream cone.
View SolutionTake π=227
एक शंकु आईसक्रीम से भरा है । शंकु के ऊपर आईसक्रीम एक अर्द्धगोले का आकार ले लेती है। अर्द्धगोले की ऊंचाई 7 सेमी. हैं अर्द्धगोले की त्रिज्या तथा शंकु की ऊंचाई समान है। आईसक्रीम का आयतन ज्ञात करें?
View SolutionAn ice-ceram cone full of ice-cream having radius 5 cm height 10 cm as shown if figure.
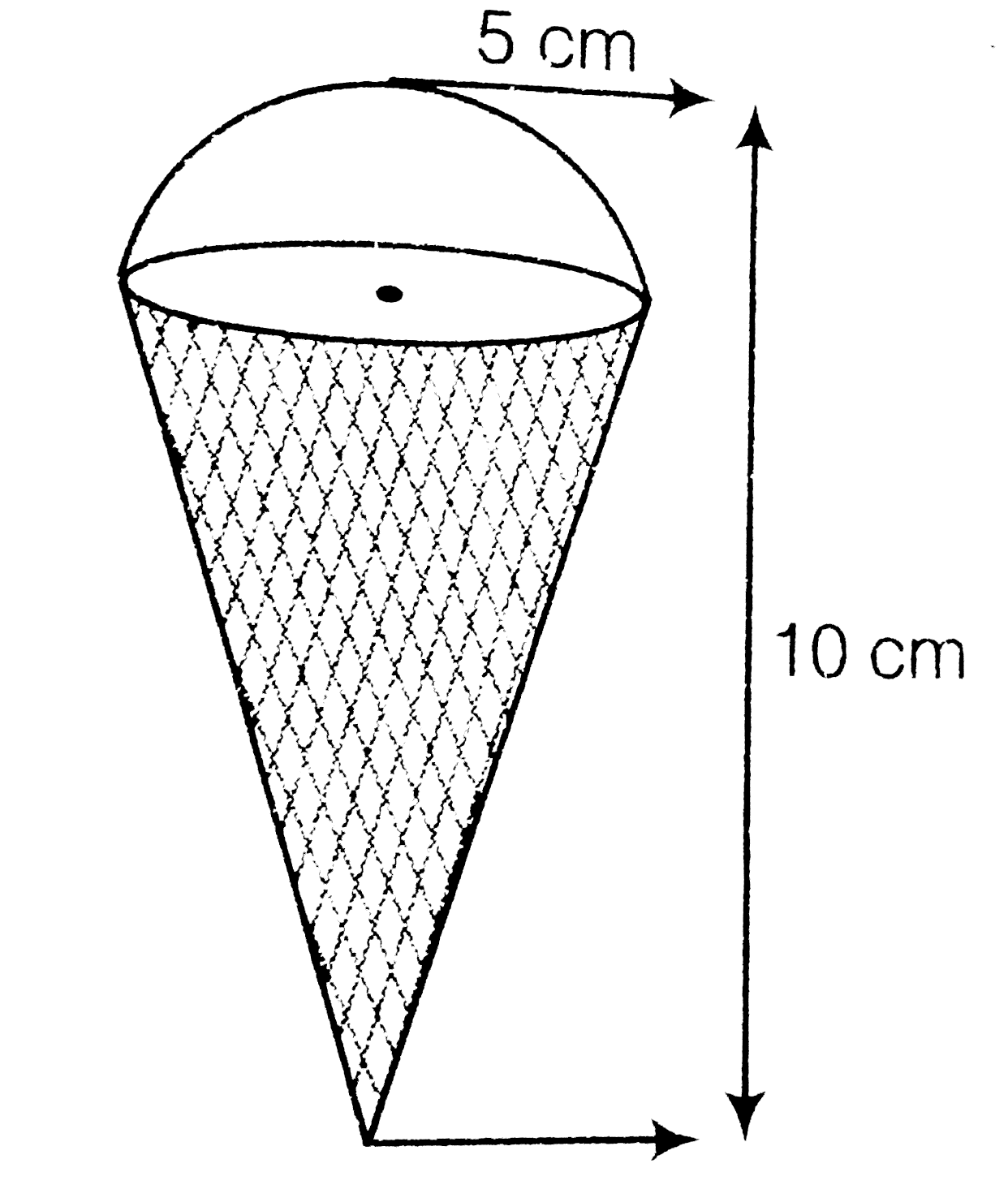
Calculate the volume of ice-cream, provided that its 16 part is left unfilled with ice-cream.
View SolutionA cylindrical ice-cream pot of radius 6 cm and height 21 cm full of ice cream. The ice cream is to be filled in cones of height 12 cm and radius 3 cm. Ice cream on the top of the cone has hemispherical shape. How many such cones can be filled with ice cream?
View SolutionA cylindrical container of radius 6 cm and height 15 cm is filled with ice cream. The whole ice cream has to be distributed to 10 children in equal cones with hemispherical tops .If the height of the conical portion is 4 times the radius of its base, find the radius of the ice cream cone.
View SolutionIf ice cream in hemisphere shape of radius 6 cm is melted to form cylinder of same radius then height of melted ice cream in cylinder form is
View SolutionIce-cream cone is of shape hemisphere mounted on cone, if radius of hemisphere is 3 cm and total height of ice-cream is 12 cm then volume of ice-cream is ( in cm3)
View SolutionA cylindrical container is filled with ice-cream whose diameter is 12 cm and height is 15 cm . The whole ice cream is distributed to 10 children by filling in equal cones and forming hemispherical tops. If the height of the conical portion is twice the diameter of its base. Find the diameter of the ice cream cone.
View SolutionA cylindrical container is filled with ice-cream whose diameter is 12 cm and height is 15 cm . The whole ice cream is distributed to 10 children by filling in equal cones and forming hemispherical tops. If the height of the conical portion is twice the diameter of its base. Find the diameter of the ice cream cone.
View SolutionA cylindrical container is filled with ice-cream whose diameter is 12 cm and height is 15 cm . The whole ice cream is distributed to 10 children by filling in equal cones and forming hemispherical tops. If the height of the conical portion is twice the diameter of its base. Find the diameter of the ice cream cone.
View SolutionA cylindrical container is filled with ice-cream whose diameter is 12 cm and height is 15 cm . The whole ice cream is distributed to 10 children by filling in equal cones and forming hemispherical tops. If the height of the conical portion is twice the diameter of its base. Find the diameter of the ice cream cone.
View SolutionA cylinderical container of diameter 12 cm and height 15 cm is filled with ice cream. The whole ice cream has to be distributed to 10 children in equal cones with hemispherical tops. If the height of the conical portion is four times the radius of its base, find the radius of the ice cream cone.
View SolutionAn ice-ceram cone full of ice-cream having radius 5 cm height 10 cm as shown if figure.

Calculate the volume of ice-cream, provided that its 16 part is left unfilled with ice-cream.
View Solution
