The probability density function of a random variable X is f(x) = k(x−1)2, 1≤x≤2. The value of the constant K isSolution in Bengali
3
4
5
6
Step by step video & image solution for The probability density function of a random variable X is f(x) = k(x-1)^2, 1 le x le 2. The value of the constant K is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
If A = {1,2,3,4} and IA be the identity relation on A, then
View SolutionLet Z be the set of integers and the mapping f:Z→Z be defined by, f(x):x2. State which of the following is equal to f−1(−4)?
View SolutionLet X is a continuous random variable with probability density function
f(x)={x6+k0≤x≤30 otherwise
The value of k is equal to
View SolutionX is continuous random variable with probability density function f(x)=x28,0≤x≤1. Then, the value of P(0.2≤X≤0.5) is
View SolutionShow that the function f(x) defined by .f(x)=17, for 1≤x≤8 =0, otherwise, is a probability density function for a random variable. Hence find P(3<X<10)
View SolutionIf the probability function of a random variable X is defined by P(X=k)=a(k+12k) for k=0,1,2,3,4,5 then the probability that X takes a prime value is
View SolutionIf the probability density function of a random variable X is f(x)=x2 in 0≤x≤2, then P(X>1.5∣X>1) is equal to
View SolutionIf X is the random variable with probability density function f(x) is given by
f(x)=⎧⎪⎨⎪⎩x+1−1≤x<0−x+10≤x<10otherwise
then find (i) the distribution function F(x) (ii) P(−0.5≤X≤0.5)
View SolutionIf f(x)={2x0≤x≤a0otherwise is a probability density function of a random variable, then the value of a is
View SolutionA continuous random varibale X has probability density function f(x)=kx20≤x<1 find k find
distrubution function
View SolutionA continuous random varibale X has probability density function f(x)=kx20≤x<1 find k find
P(X<5)
View SolutionA continuous random varibale X has probability density function f(x)=kx20≤x<1 find k
P(1<x<5)
View SolutionIf f(x)=k(2x−x2),0≤x≤1 is probability density function of a continous random variable x then k is
View SolutionIf the probability function of a random variable X is defined by P(X=k)=a(k+12k) for k=0,1,2,3,4,5, then the probability that X takes a prime value is
View SolutionProbability distribution of a discrete random variable X is given in the following table.
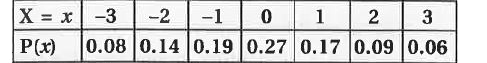
1) Find the probability of random variable X assuming negative values.
2) Find the value of P(0≤x≤3)
View SolutionX is continuous random variable with probability density function f(x)=x28,0≤x≤1. Then, the value of P(0.2≤X≤0.5) is
View Solution
