If the parabola y=ax2+bx+c,wherea,b,c∈{1,2,3,4,5,6} pass through (1, 8), then number of such parabola is k the k−12
The correct Answer is:9
To solve the problem of finding the number of parabolas of the form y=ax2+bx+c that pass through the point (1, 8), where a,b,c are integers from the set \{1, 2, 3, 4, 5, 6\}, we can follow these steps:
Step 1: Set up the equation
Since the parabola passes through the point (1, 8), we can substitute x=1 and y=8 into the equation:
8=a(1)2+b(1)+c
This simplifies to:
8=a+b+c
Step 2: Determine the range of values for a,b,c
We know that a,b,c can take values from the set \{1, 2, 3, 4, 5, 6\}. Therefore, we need to find combinations of a,b,c such that their sum equals 8.
Step 3: Analyze possible values for a
We will analyze each possible value of a from 1 to 6 and find corresponding values of b and c that satisfy the equation b+c=8−a.
1. If a=1:
b+c=8−1=7
Possible pairs (b,c): (1, 6), (2, 5), (3, 4), (4, 3), (5, 2), (6, 1) → 6 combinations.
2. If a=2:
b+c=8−2=6
Possible pairs (b,c): (1, 5), (2, 4), (3, 3), (4, 2), (5, 1) → 5 combinations.
3. If a=3:
b+c=8−3=5
Possible pairs (b,c): (1, 4), (2, 3), (3, 2), (4, 1) → 4 combinations.
4. If a=4:
b+c=8−4=4
Possible pairs (b,c): (1, 3), (2, 2), (3, 1) → 3 combinations.
5. If a=5:
b+c=8−5=3
Possible pairs (b,c): (1, 2), (2, 1) → 2 combinations.
6. If a=6:
b+c=8−6=2
Possible pairs (b,c): (1, 1) → 1 combination.
Step 4: Calculate total combinations
Now we sum all the combinations found:
6+5+4+3+2+1=21
Thus, the total number of parabolas k=21.
Step 5: Find k−12
Finally, we compute:
k−12=21−12=9
Final Answer:
The value of k−12 is 9.
Since the parabola passes through the point (1, 8), we can substitute x=1 and y=8 into the equation:
8=a(1)2+b(1)+c
This simplifies to:
8=a+b+c
We know that a,b,c can take values from the set \{1, 2, 3, 4, 5, 6\}. Therefore, we need to find combinations of a,b,c such that their sum equals 8.
We will analyze each possible value of a from 1 to 6 and find corresponding values of b and c that satisfy the equation b+c=8−a.
b+c=8−1=7
Possible pairs (b,c): (1, 6), (2, 5), (3, 4), (4, 3), (5, 2), (6, 1) → 6 combinations.
b+c=8−2=6
Possible pairs (b,c): (1, 5), (2, 4), (3, 3), (4, 2), (5, 1) → 5 combinations.
b+c=8−3=5
Possible pairs (b,c): (1, 4), (2, 3), (3, 2), (4, 1) → 4 combinations.
b+c=8−4=4
Possible pairs (b,c): (1, 3), (2, 2), (3, 1) → 3 combinations.
b+c=8−5=3
Possible pairs (b,c): (1, 2), (2, 1) → 2 combinations.
b+c=8−6=2
Possible pairs (b,c): (1, 1) → 1 combination.
Now we sum all the combinations found:
6+5+4+3+2+1=21
Thus, the total number of parabolas k=21.
Finally, we compute:
k−12=21−12=9
The value of k−12 is 9.
Topper's Solved these Questions
Similar Questions
Knowledge Check
A parabola passes through the points (0, 0) and (6, 0). If the turning point of the parabola is T(h,4), which statement must be true?
I. h=2
II. If the parabola passes through (1, 2), then it must also pass through (5, 2).
III. Point T is the highest point of the parabolaAII onlyBIII onlyCI and II onlyDII and III only
If (a,b) is the midpoint of a chord passing through the vertex of the parabola y2=4(x+1), then prove that 2(a+1)=b2.
View SolutionStatement 1: Normal chord drawn at the point (8, 8) of the parabola y2=8x subtends a right angle at the vertex of the parabola. Statement 2: Every chord of the parabola y2=4ax passing through the point (4a,0) subtends a right angle at the vertex of the parabola.
View SolutionIf a line x+y=1 cut the parabola y2=4ax in points A and B and normals drawn at A and B meet at C. The normals to the parabola from C other than above two meets the parabola in D, then point D is : (A) (a,a) (B) (2a,2a) (C) (3a,3a) (D) (4a,4a)
View SolutionThe vertex of a parabola is the point (a,b) and the latus rectum is of length l. If the axis of the parabola is parallel to the y-axis and the parabola is concave upward, then its equation is a. (x+a)2=12(2y−2b) b. (x−a)2=12(2y−2b) c. (x+a)2=14(2y−2b) d. (x−a)2=18(2y−2b)
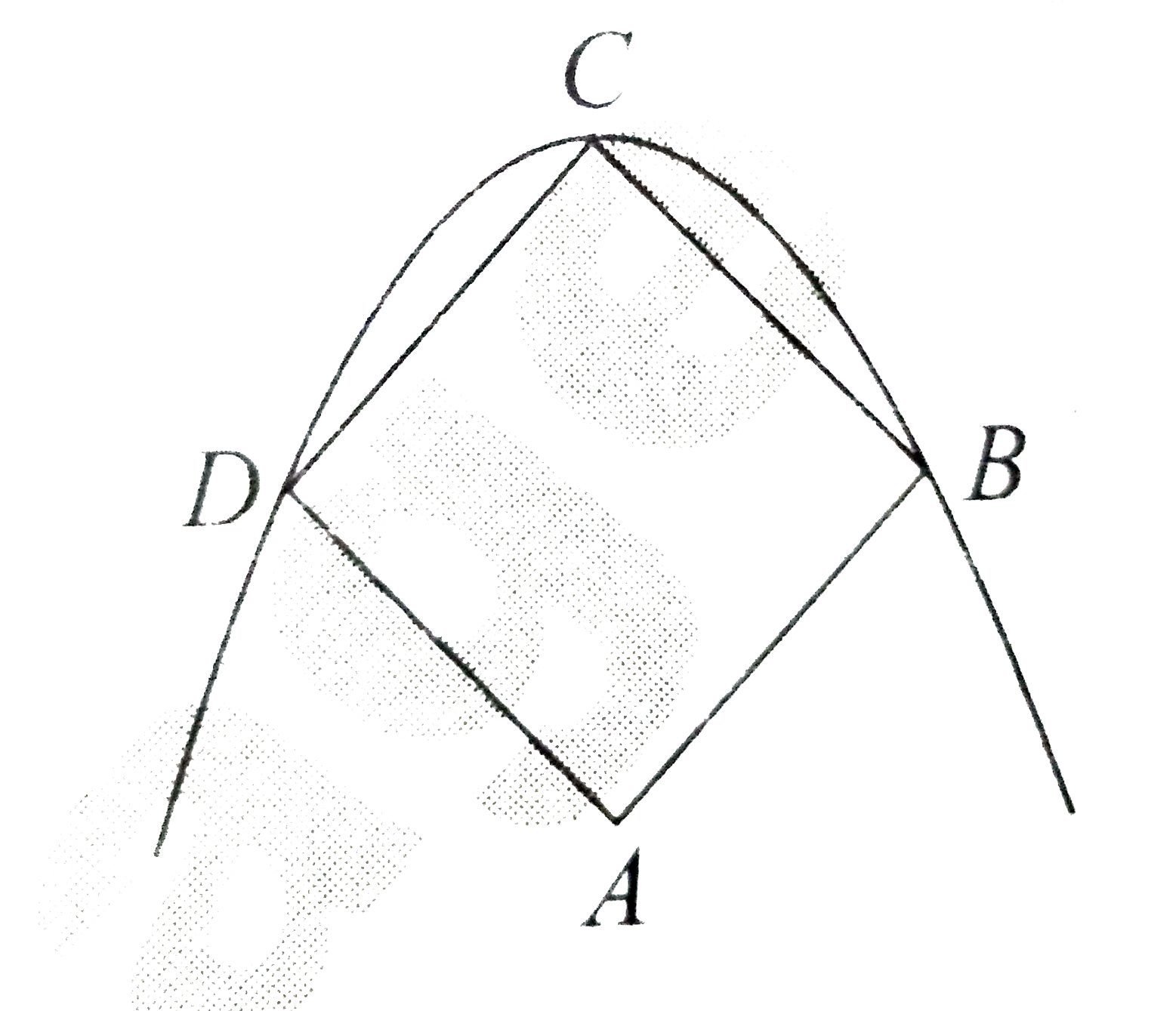
View SolutionIn the adjacent figure a parabola is drawn to pass through the vertices B,C and D of the square ABCD. If A(2,1),C(2,3), then focus of this parabola is
View SolutionIf the line passing through the focus S of the parabola y=ax2+bx+c meets the parabola at PandQ and if SP=4 and SQ=6 , then find the value of a.
View SolutionIf the line passing through the focus S of the parabola y=ax2+bx+c meets the parabola at PandQ and if SP=4 and SQ=6 , then find the value of a.
View Solution
