There are 5 routes ferom A to B and 3 routes from place B to place C. Find how many different routes are there from A to C via B.
To solve the problem of finding the number of different routes from A to C via B, we can follow these steps:
Step 1: Identify the number of routes from A to B
We are given that there are 5 routes from A to B.
Step 2: Identify the number of routes from B to C
We are also given that there are 3 routes from B to C.
Step 3: Use the multiplication principle
To find the total number of different routes from A to C via B, we can use the multiplication principle (also known as the universal law of multiplication). According to this principle, if there are m ways to do one thing and n ways to do another, then there are m×n ways to do both.
Step 4: Calculate the total routes
In our case, we have:
- m=5 (routes from A to B)
- n=3 (routes from B to C)
Thus, the total number of routes from A to C via B is:
Total routes=5×3=15
Conclusion
Therefore, there are a total of 15 different routes from A to C via B.
---
We are given that there are 5 routes from A to B.
We are also given that there are 3 routes from B to C.
To find the total number of different routes from A to C via B, we can use the multiplication principle (also known as the universal law of multiplication). According to this principle, if there are m ways to do one thing and n ways to do another, then there are m×n ways to do both.
In our case, we have:
- m=5 (routes from A to B)
- n=3 (routes from B to C)
Total routes=5×3=15
Therefore, there are a total of 15 different routes from A to C via B.
Similar Questions
A person wants to go from station A to station C via station b. There are three routes from A to B four routes from B to C. In how many ways can he travel from A to C?
View SolutionThere are five routes for a journey from stations . A to B . In how many different ways can a man go from A to B and return , if for returning.
any of the routes is taken
View Solution
Knowledge Check
There are 4 bus routes between A and B and 3 bus routes between B and C. A man can travel round trip in number of ways by bus from A to C via B. If he does not want to use a bus route more than once, in how many ways can he make round trip?
A72B142C14D19
There are five routes for a journey from stations . A to B . In how many different ways can a man go from A to B and return , if for returning.
the same route is taken
View SolutionThere are five routes for a journey from stations . A to B . In how many different ways can a man go from A to B and return , if for returning.
the same route is not taken?
View SolutionA person wants to leave station B. There are three routes from station B to A and four routes from B to c. In how many ways can he leave the station B.
View SolutionThere are three stations A,B and C five routes for going from station A to station B and four routes for going from station B to station C. find number of different ways through which a person can go from A to C via B.
View SolutionFrom Goa to Bombay there are two roots; air, and sea. From Bombay to Delhi there are three routes air, rail and road. From Goa to Delhi via Bombay, how many kinds of routes are there?
View SolutionDinesh went from place A to place B and from there to place C. A is 7.5 km from B and B is 12.7 km from C. Ayub went from place A to placeD and from there to place C. D is 9.3 km from A and C is 11.8 km from D. Who travelled more and by how much?
View SolutionFind the solution A,B and C in which different plant cells are placed
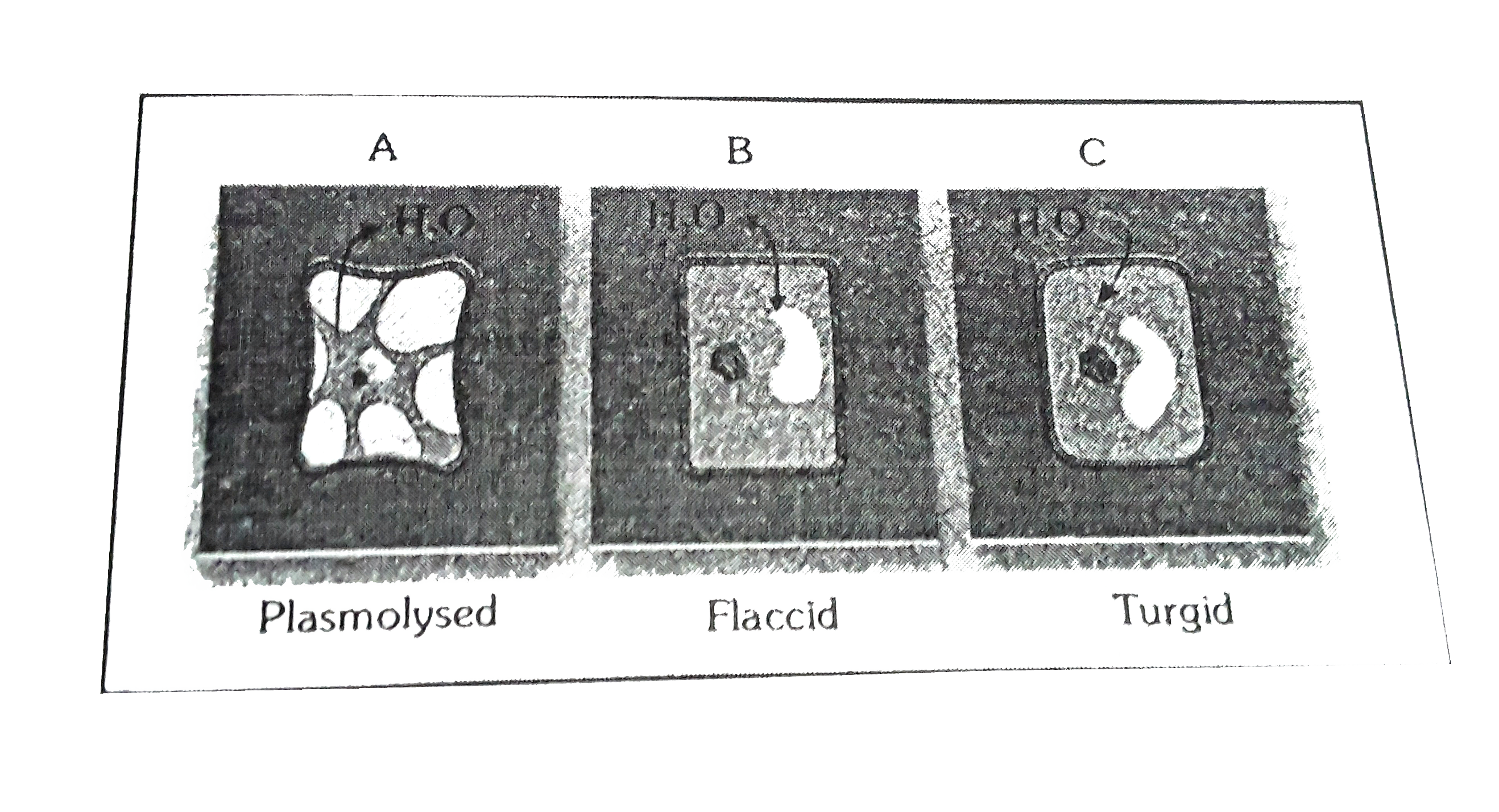
View Solution
