The focus of a parabolic mirror as shown in the figure alongside is at a distance of 6cm from its vertex. If the mirror is 20cm deep, find the distance BC.
To solve the problem, we need to find the distance BC in the parabolic mirror setup described. Here’s the step-by-step solution:
Step 1: Understand the given information
- The focus (A) of the parabolic mirror is 6 cm from the vertex (O).
- The depth of the mirror (OD) is 20 cm.
- We need to find the distance BC.
Step 2: Set up the equation of the parabola
For a parabola that opens to the right, the standard equation is given by:
y2=4ax
where a is the distance from the vertex to the focus. Here, a=6 cm.
Thus, the equation of the parabola becomes:
y2=24x
Step 3: Determine the x-coordinate of point B
Since the depth of the mirror is 20 cm, the x-coordinate of point B (which is at the depth of the mirror) is:
x=20
Step 4: Substitute x into the parabola equation to find y
We substitute x=20 into the equation of the parabola to find the y-coordinate (h) of point B:
y2=24⋅20
y2=480
Step 5: Solve for y
Taking the square root of both sides gives:
y=√480
Calculating this, we find:
y≈21.9 cm
Step 6: Calculate distance BC
The distance BC is twice the value of h (since BC spans from point B to point C, which are symmetric about the x-axis):
BC=2h=2⋅21.9
BC≈43.8 cm
Final Answer
The distance BC is approximately 43.8 cm.
---
- The focus (A) of the parabolic mirror is 6 cm from the vertex (O).
- The depth of the mirror (OD) is 20 cm.
- We need to find the distance BC.
For a parabola that opens to the right, the standard equation is given by:
y2=4ax
where a is the distance from the vertex to the focus. Here, a=6 cm.
y2=24x
Since the depth of the mirror is 20 cm, the x-coordinate of point B (which is at the depth of the mirror) is:
x=20
We substitute x=20 into the equation of the parabola to find the y-coordinate (h) of point B:
y2=24⋅20
y2=480
Taking the square root of both sides gives:
y=√480
Calculating this, we find:
y≈21.9 cm
The distance BC is twice the value of h (since BC spans from point B to point C, which are symmetric about the x-axis):
BC=2h=2⋅21.9
BC≈43.8 cm
The distance BC is approximately 43.8 cm.
Similar Questions
The focus of a parabolic mirror as shown in figure is at a distance of 5 cm from its vertex. If the mirror is 45 cm deep, find the distance AB
View SolutionThe focus of a parabolic mirror is at a distance of 6 cm from its vertex. If the mirror is 20 cm deep, find the distance LM.
View SolutionA concave mirror forms an image of the sun at a distance of 12 cm from it.
View SolutionA parabola reflector is 15 cm deep and its focus is at a distance of 5 cm from its vertex. Find the diameter of the reflector.
View SolutionA slab of glass, of thickness 6 cm and refractive index μ=1.5 is placed in front of a concave mirror as shown in the figure. If the radius of curvature of the mirror is 40 cm and the reflected image coincides with the object, then the distance of the object from the mirror is

View SolutionAn object is placed in front of a concave mirror at a distance of 7.5 cm from it. If the real image is formed at a distance of 30 cm from the mirror, find the focal length of the mirror. What would be the focal length if the image is virtual.
View SolutionConcave mirror forms the real image of a point source lying on the optical axis at a distance of 50 cm from the mirror. The focal length of the mirror is 25 cm. The mirror is cut into and its halves are drawn a distance of 1 cm apart in a direction perpendicular to the optical axis. How will the image formed by the halves of the mirror be arranged?
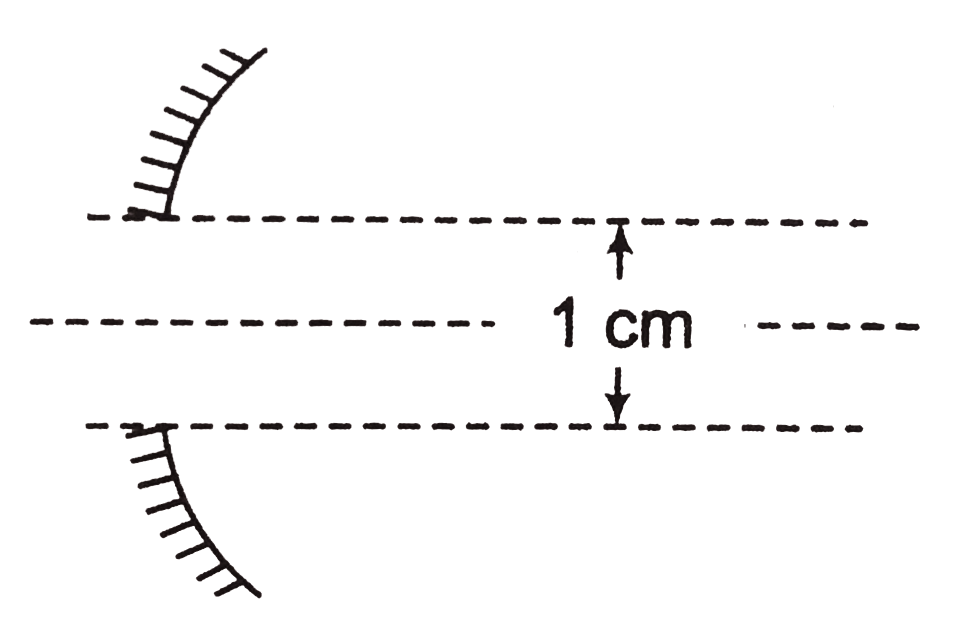
View SolutionA point light source is kept in front of a convex mirror at a distance of 40 cm. The focal length of the mirror is 40 cm. Find the position of image.
View SolutionThe focal length of a convex mirror is 40 cm. A point source of light is kept at distance 40 cm from the mirror. Find the distance of image from the mirror.
View SolutionAn object is placed at a distance of 30 cm from a concave mirror and its real image is formed at a distance of 30 cm from the mirror. The focal length of the mirror is
View Solution
