A straight line l passes through a fixed point (6, 8). If locus of the foot of perpendicular on line l from origin is a circle, then radius of this circle is … .
Step by step video & image solution for A straight line l passes through a fixed point (6, 8). If locus of the foot of perpendicular on line l from origin is a circle, then radius of this circle is … . by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
A given line L1 cut x and y-axes at PandQ respectively and has intercepts aandb2 on x and y-axes respectively. Let another line L2 perpendicular to L1 cut x and y-axes at RandS respectively. Let T be the point of intersection of PSandQR. A straight line passes through the centre of locus of T. Then locus of the foot of perpendicular to it from origin is : (A) a straight line (B) a circle (C) a parabola (D) none of these
View SolutionA plane passes through a fixed point (a,b,c). Show that the locus of the foot of the perpendicular to it from the origin is the sphere x2+y2+z2−ax−by−cz=0.
View SolutionA plane passes through a fixed point (a,b,c). Show that the locus of the foot of the perpendicular to it from the origin is the sphere x2+y2+z2−ax−by−cz=0.
View SolutionThe locus of the foot of perpendicular drawn from origin to a variable line passing through fixed points (2,3) is a circle whose diameter is?
View SolutionThe equation of the straight line passing through the point (3,2) and perpendicular to the line y=x is
View SolutionFind the locus of the foot of the perpendicular from the origin to the line which always passes through a fixed point(h, k).
View SolutionA variable line intersects the co-ordinate axes at A and B and passes through a fixed point (a,b).then the locus of the vertex C of the rectangle OACB where O is the origin is
View SolutionA straight line moves so that the product of the length of the perpendiculars on it from two fixed points is constant. Prove that the locus of the feet of the perpendiculars from each of these points upon the straight line is a unique circle.
View Solution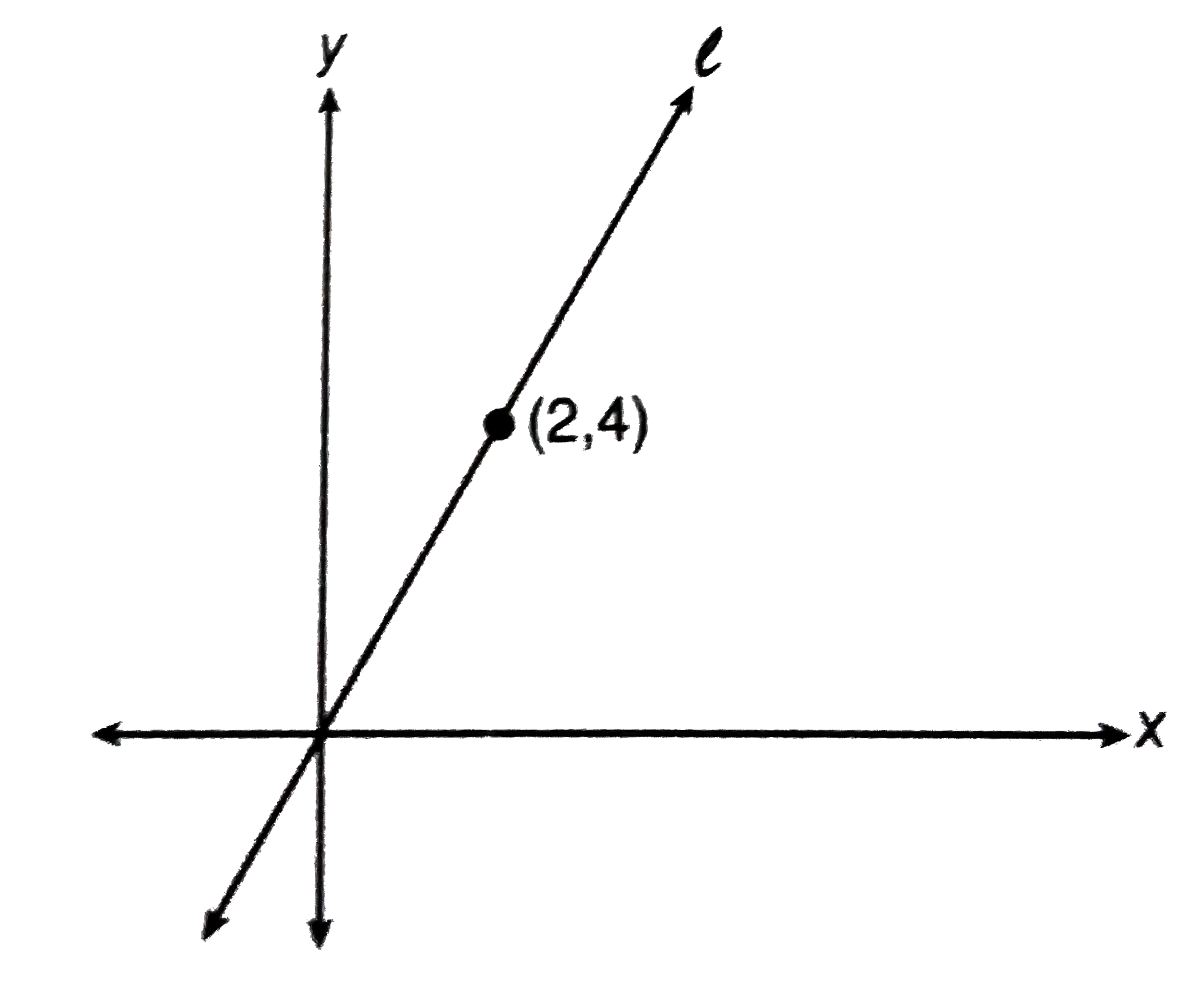
In the accompanying figure. Line l passes through the origin and the point (2, 4). Line m (not shown) is perpendicular to line l at (2, 4). Line m intersects the x-axis at which point?
View SolutionA variable circle passes through the fixed point (2, 0) and touches y-axis Then, the locus of its centre, is
View Solution
