If in a △ABC,∑cos3A=1, then (A) △ABC is a rilght angled triangle (B) △ABC is an obtuse angled triangle (C) △ABC is an acute angled triangle (D) In radius r of △ABC is either √3(s+a)or√3(s+b)or√3(s+c)
To solve the problem, we need to analyze the given condition that the sum of cosines of three times the angles of triangle ABC is equal to 1, i.e.,
cos3A+cos3B+cos3C=1
Step 1: Use the cosine sum identity
We can express the sum of cosines using the identity for the sum of cosines:
cosA+cosB=2cos(A+B2)cos(A−B2)
Applying this identity to our equation:
cos3A+cos3B=2cos(3A+3B2)cos(3A−3B2)
Thus, we can rewrite our equation as:
2cos(3(A+B)2)cos(3(A−B)2)+cos3C=1
Step 2: Substitute A+B
Since A+B+C=180∘, we have A+B=180∘−C. Therefore, we can substitute:
cos(3(180∘−C)2)=cos(270∘−3C2)=−sin(3C2)
So the equation becomes:
−2sin(3C2)cos(3(A−B)2)+cos3C=1
Step 3: Analyze the equation
We know that:
cos3C=4cos3C−3cosC
Setting this equal to the rest of the equation, we can analyze the values of C.
Step 4: Solve for C
To satisfy the equation, we can set:
sin(3C2)=0
This occurs when:
3C2=nπfor n∈Z
Thus, we can find C:
C=2nπ3
For C to be an angle in a triangle, we can take n=1:
C=2⋅180∘3=120∘
Step 5: Determine the type of triangle
Since C=120∘ is greater than 90∘, triangle ABC is an obtuse triangle.
Conclusion
Thus, the correct option is:
(B) Triangle ABC is an obtuse angled triangle.
We can express the sum of cosines using the identity for the sum of cosines:
Since A+B+C=180∘, we have A+B=180∘−C. Therefore, we can substitute:
We know that:
To satisfy the equation, we can set:
Since C=120∘ is greater than 90∘, triangle ABC is an obtuse triangle.
Thus, the correct option is:
Similar Questions
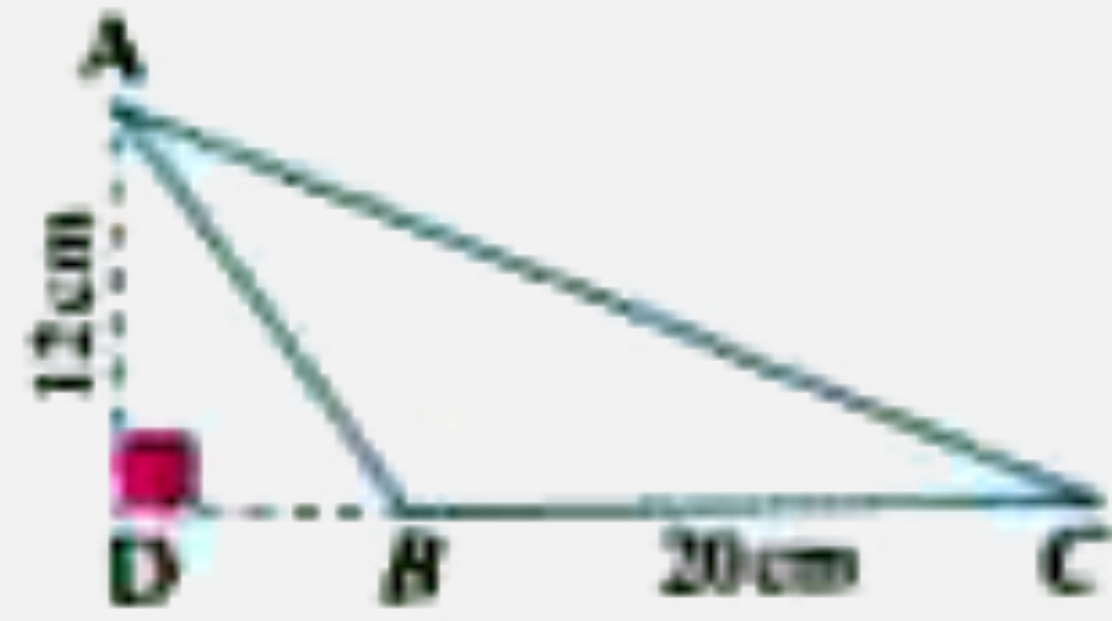
Find the ara of the given obtuse-angled triangle ABC.
View SolutionIn an acute-angled triangle ABC, tanA+tanB+tanC
View SolutionIf in a triangle ABC,tanA+tanB+tanC>0 the triangle is (A) acute angled triangle (B) obtuse angled (C) right angled (D) none of these
View SolutionIn a triangle ABC,sinA−cosB=CosC, then angle B is
View SolutionIn a triangle ABC,vertex angles A,B,C and side BC are given .The area of △ABC is
View SolutionIf triangle ABC is right angled at C, then the value of sec (A+B) is
View SolutionIn an acute angled triangle ABC, r+r1=r2+r3and∠B>π3, then
View SolutionIf in a triangle ABC, θ is the angle determined by cosθ=(a−b)/c, then
View SolutionIf in a triangle ABC,a2+b2+c2=ca+ab√3, then the triangle is
View SolutionThe angles of a triangle ABC are in A.P and b:c = √3:√2 find ∠A
View Solution
