If P is the point (2,1,6) find the point Q such that PQ is perpendicular to the plane x+y−2z=3 and the mid point of PQ lies on it.
To solve the problem, we need to find the point Q such that the line segment PQ is perpendicular to the plane given by the equation x+y−2z=3, and the midpoint of PQ lies on this plane.
Step 1: Identify the given point P and the plane equation
The point P is given as P(2,1,6) and the equation of the plane is x+y−2z=3.
Step 2: Determine the normal vector of the plane
The normal vector →n of the plane can be derived from the coefficients of x, y, and z in the plane equation. Therefore, the normal vector is:
→n=(1,1,−2)
Step 3: Write the parametric equations of the line PQ
Since PQ is perpendicular to the plane, the direction ratios of the line PQ are the same as the normal vector. The parametric equations of the line can be expressed as:
x−21=y−11=z−6−2=λ
From this, we can express x, y, and z in terms of λ:
x=λ+2,y=λ+1,z=−2λ+6
Step 4: Find the coordinates of the foot of the perpendicular (point R)
The point R is the foot of the perpendicular from P to the plane. Since R lies on the line PQ, it must satisfy the plane equation. Substituting the coordinates of R into the plane equation:
(λ+2)+(λ+1)−2(−2λ+6)=3
Simplifying this equation:
λ+2+λ+1+4λ−12=3
6λ−9=3
6λ=12⟹λ=2
Step 5: Substitute λ back to find coordinates of R
Now substituting λ=2 back into the equations for x, y, and z:
x=2+2=4,y=2+1=3,z=−2(2)+6=2
Thus, the coordinates of point R are R(4,3,2).
Step 6: Use the midpoint formula to find Q
The midpoint M of segment PQ is given by:
M=(xP+xQ2,yP+yQ2,zP+zQ2)
Since M lies on the plane, we have:
2+xQ2=4,1+yQ2=3,6+zQ2=2
Step 7: Solve for the coordinates of Q
1. For xQ:
2+xQ2=4⟹2+xQ=8⟹xQ=6
2. For yQ:
1+yQ2=3⟹1+yQ=6⟹yQ=5
3. For zQ:
6+zQ2=2⟹6+zQ=4⟹zQ=−2
Final Answer
Thus, the coordinates of point Q are:
Q(6,5,−2)
The point P is given as P(2,1,6) and the equation of the plane is x+y−2z=3.
The normal vector →n of the plane can be derived from the coefficients of x, y, and z in the plane equation. Therefore, the normal vector is:
→n=(1,1,−2)
Since PQ is perpendicular to the plane, the direction ratios of the line PQ are the same as the normal vector. The parametric equations of the line can be expressed as:
x−21=y−11=z−6−2=λ
From this, we can express x, y, and z in terms of λ:
x=λ+2,y=λ+1,z=−2λ+6
The point R is the foot of the perpendicular from P to the plane. Since R lies on the line PQ, it must satisfy the plane equation. Substituting the coordinates of R into the plane equation:
(λ+2)+(λ+1)−2(−2λ+6)=3
Simplifying this equation:
λ+2+λ+1+4λ−12=3
6λ−9=3
6λ=12⟹λ=2
Now substituting λ=2 back into the equations for x, y, and z:
x=2+2=4,y=2+1=3,z=−2(2)+6=2
Thus, the coordinates of point R are R(4,3,2).
The midpoint M of segment PQ is given by:
M=(xP+xQ2,yP+yQ2,zP+zQ2)
Since M lies on the plane, we have:
2+xQ2=4,1+yQ2=3,6+zQ2=2
1. For xQ:
2+xQ2=4⟹2+xQ=8⟹xQ=6
2. For yQ:
1+yQ2=3⟹1+yQ=6⟹yQ=5
3. For zQ:
6+zQ2=2⟹6+zQ=4⟹zQ=−2
Thus, the coordinates of point Q are:
Q(6,5,−2)
Similar Questions
If P is the point (2,1,6) find the point Q such that PQ is perpendiculr to the plane in x+y-2z =3 and the mid point of PQ lies on it.
View SolutionFind the equation of the plane passing through the points(2,1,0),(5,0,1) and (4,1,1) If P is the point (2,1,6) then find point Q such that PQ is perpendicular to the above plane and the mid point of PQ lies on it.
View Solution
Knowledge Check
If P is the point (1,0) and Q is any point on the parabola y2=8x then the locus of mid - point of PQ is
AP1 lies on C2andQ1 lies on C1BPQ≥min{PP1,QQ1}Cpoint P0 on C1 such that P0Q0≤PQ for all pairs of points (P,Q) is (13,109)Dpoint Q0 on C2 such that P0Q0≤PQ for all pairs of points (P,Q) is (109,13)
Let P be a point in the first octant, whose image Q in the plane x+y=3 (that is, the line segment PQ is perpendicular to the plane x+y=3 and the mid-point of PQ lies in the plane x+y=3) lies on the z-axis. Let the distance of P from the x-axis be 5. If R is the image of P in the xy-plane, then the length of PR is _______.
View SolutionConsider the line L≡x−12=y+23=z−76. Point P(2,−5,0) and Q are such that PQ is perpendicular to the line L and the midpoint of PQ lies on line L, then coordinates of Q are
View SolutionFind the equation of the plane that contains the point (1,−1,2)and is perpendicular to each of the planes 2x+3y−2z=5and x+2y−3z=8.
View SolutionA plane P = 0 passing through the point (1, 1, 1) is perpendicular to the planes 2x−y+2z=5 and 3x+6y−2z=7. If the distance of the point (1, 2, 3) from the plane P = 0 is k units, then the value of 34k2 is equal to
View SolutionThe distance of the point (1,−2,4) from the plane passing through the point (1,2,2) perpendicular to the planes x−y+2z=3 and 2x−2y+z+12=0 is
View SolutionFind the equation of plane perpendicular to the plane 2x + 3y - 5z - 6 = 0 and passing through the point P(2,-1,-1) and Q(1,2,3)
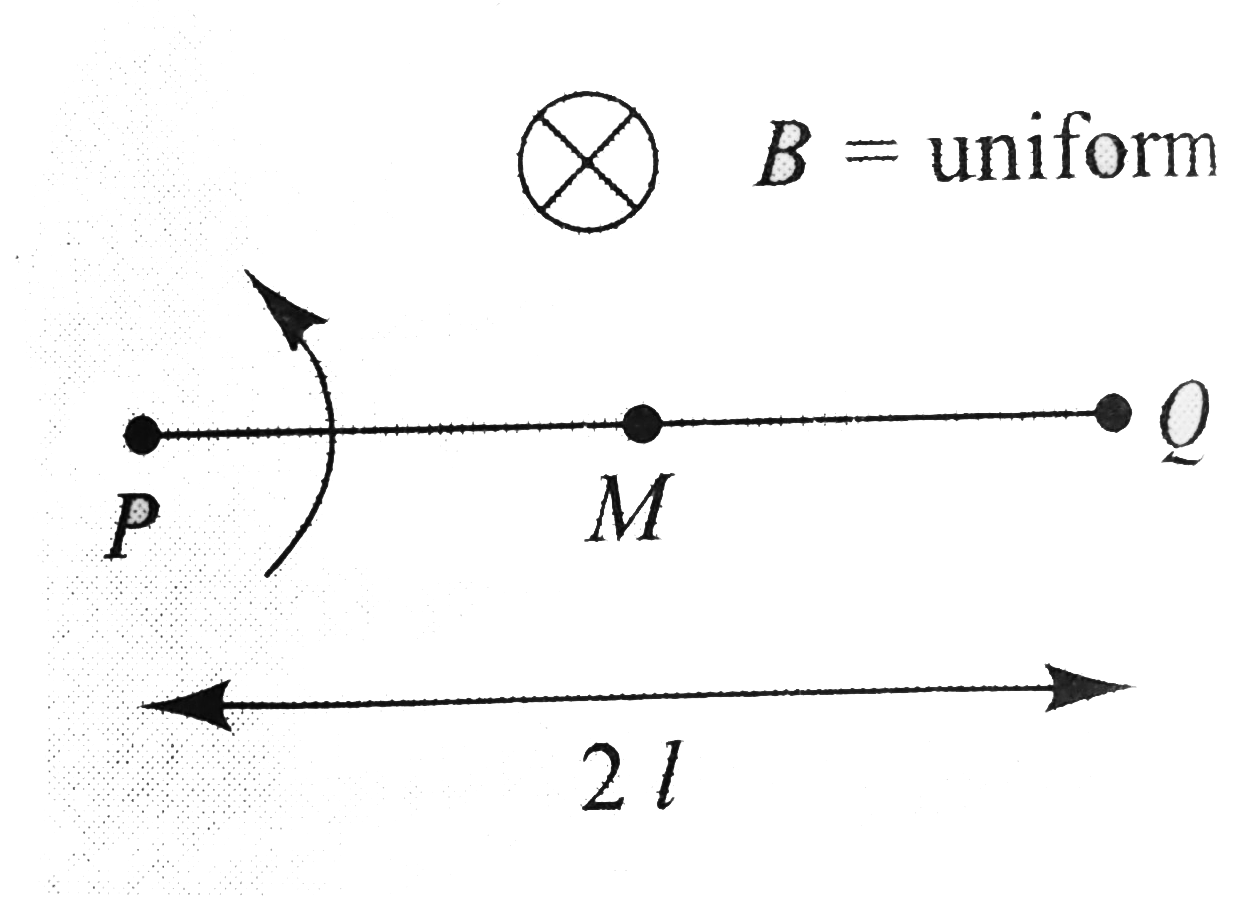
View SolutionRod PQ of length 2l is rotating about one end P in a uniform magnetic field B which is perpendicular to the plane of rotation of the rod . Point M is the mid- point of the rod. Find the induced emf between M and Q if the potential between P and Q is 100V.
View Solution
