Each question has four choices a, b, c and d, out of which only one is correct. Each question contains STATEMENT 1 and STATEMENT 2. Both the statements are TRUE and STATEMENT 2 is the correct explanation of STATEMENT1. Both the statements are TRUE but STATEMENT 2 is NOT the correct explanation of STATEMENT 1. STATEMENT 1 is TRUE and STATEMENT 2 is FALSE. STATEMENT 1 is FALSE and STATEMENT 2 is TRUE. Statement 1: if are the angles of a triangles and , then triangle may not be equilateral Statement 2: if any two rows of a determinant are the same, then the value of that determinant is zero.Solution in Tamil
Step by step video & image solution for Each question has four choices a, b, c and d, out of which only one is correct. Each question contains STATEMENT 1 and STATEMENT 2. Both the statements are TRUE and STATEMENT 2 is the correct explanation of STATEMENT1. Both the statements are TRUE but STATEMENT 2 is NOT the correct explanation of STATEMENT 1. STATEMENT 1 is TRUE and STATEMENT 2 is FALSE. STATEMENT 1 is FALSE and STATEMENT 2 is TRUE. Statement 1: if A ,B ,C are the angles of a triangles and |1 1 1 1+s in A1+sinB1+s in C s in A+sin^2A s in B+sin^2B s in C+sin^2C|=0 , then triangle may not be equilateral Statement 2: if any two rows of a determinant are the same, then the value of that determinant is zero. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
consider the fourth -degree polynomial equation
∣∣ ∣ ∣∣a1+b1xa1x2+b1c1a2+b2x2a2x2+b2c2a3+b3x2a3x2+b3c3∣∣ ∣ ∣∣=0
Without expanding the determinant find all the roots of the equation.
View SolutionFind |→a|and∣∣→b∣∣, if (→a+→b)⋅(→a−→b)=8and|→a|=8∣∣→b∣∣.
View SolutionEach question has four choices a,b,c and d out of which only one is correct. Each question contains Statement 1 and Statement 2. Make your answer as: If both the statements are true and Statement 2 is the correct explanation of statement 1. If both the statements are True but Statement 2 is not the correct explanation of Statement 1. If Statement 1 is True and Statement 2 is False. If Statement 1 is False and Statement 2 is True. It is given that a≻,b>0,c>0anda+b+c=abc Statement 1: All of the three numbers cannot be less than √3. Statement 2: In ABC. tanA+tanB+tanC=tanAtanBtanC
View SolutionEach question has four choices a,b,c and d out of which only one is correct. Each question contains Statement 1 and Statement 2. Make your answer as: a) If both the statements are true and Statement 2 is the correct explanation of statement 1. b) If both the statements are True but Statement 2 is not the correct explanation of Statement 1. c) If Statement 1 is True and Statement 2 is False. d) If Statement 1 is False and Statement 2 is True. Statement 1: If A,B,C are the angles of a triangle such that angle A is obtuse, then tanBtanC>1. Statement 2: In any triangle, tanA=tanB+tanCtanBtanC−1
View SolutionEach question has four choices a, b, c and d, out of which only one is correct. Each question contains STATEMENT 1 and STATEMENT 2. Both the statements are TRUE and STATEMENT 2 is the correct explanation of STATEMENT1. Both the statements are TRUE but STATEMENT 2 is NOT the correct explanation of STATEMENT 1. STATEMENT 1 is TRUE and STATEMENT 2 is FALSE. STATEMENT 1 is FALSE and STATEMENT 2 is TRUE. Statement 1: |adj(adj(adjA))|−|A|n−1^3 , where n is order of matrix A. Statement 2: |adjA|=|A|n.
View SolutionEach question has four choice: a, b, c and d, out of which only one is correct. Each question contains Statement 1 and Statement 2. Find the correct answer. Both the Statements are true but Statement 2 is the correct explanation of Statement 1. Both the Statement are True but Statement 2 is not the correct explanation of Statement 1. Statement 1 is True and Statement 2 is False. Statement 1 is False and Statement 2 is True Statement 1: The lines (a+b)x+(a−2b)y=a are con-current at the point (23,13). Statement 2: The lines x+y−1=0 and x−2y=0 intersect at the point (23,13).
View SolutionEach question has 5 choices (a) ,(b) ,(c ) (d) and (e ) out of which ONLY ONE is correct. (a) Statement -1 is True, Statement -2 is True , Statement -2 is a correct explanation for Statement -1. (b) Statement -1 is True, Statement -2 is True, Statement -2 is NOT a correct explanation for Statement -1. (C ) Statement -1 is True, Statement -2 is False. (d) Statement -1 is False, Statement -2 is True. (e) Statement -1 and Statement -2 both are False. Statement -1: Yield of R-Br follows the order 1^(@) gt 2^(@) gt 3^(@) in Hundsdicker reaction Statement -2: Hundsdicker reaction follows free redical mechanism.
View SolutionEach question has 5 choices (a) ,(b) ,(c ) (d) and (e ) out of which ONLY ONE is correct.
(a) Statement -1 is True, Statement -2 is True , Statement -2 is a correct explanation for Statement -1.
(b) Statement -1 is True, Statement -2 is True, Statement -2 is NOT a correct explanation for Statement -1.
(C ) Statement -1 is True, Statement -2 is False.
(d) Statement -1 is False, Statement -2 is True.
(e) Statement -1 and Statement -2 both are False.
Statement -1: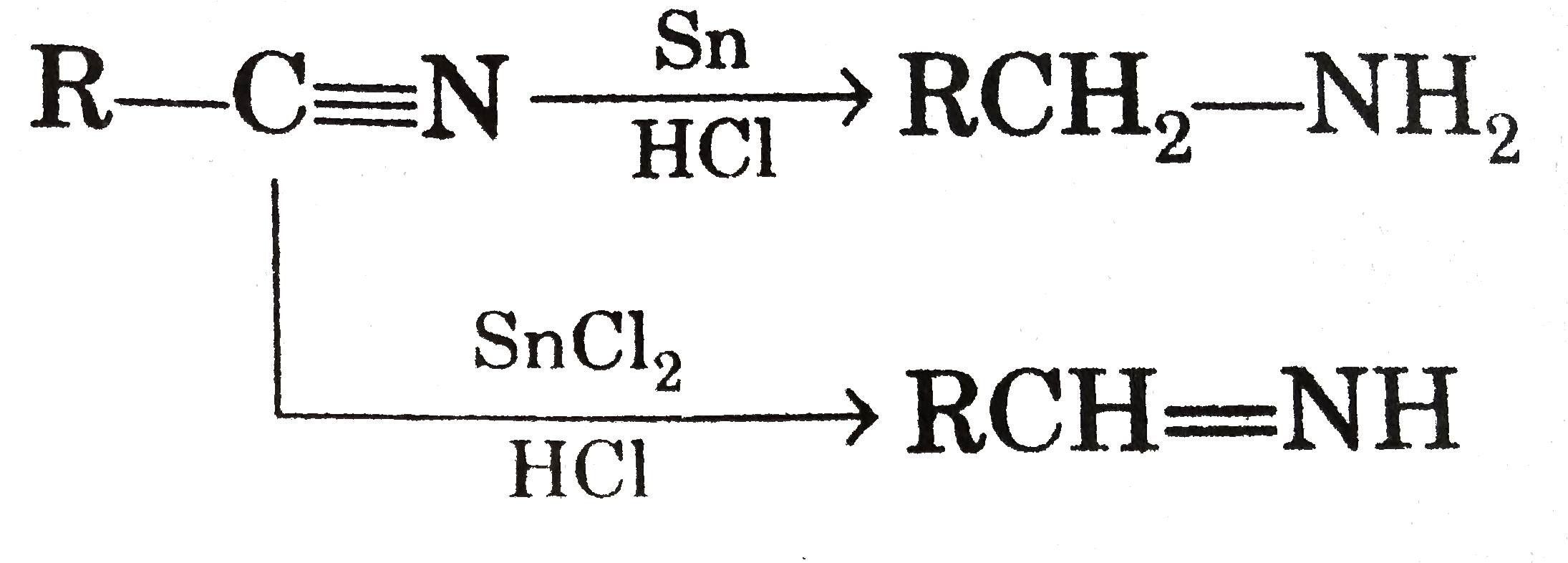
Statement-2: Sn can give maximum of 4 electron during reduction, whereas SnCl2 can give only 2.
View SolutionEach question has four choices a,b,c and d out of which only one is correct. Each question contains Statement 1 and Statement 2. Make your answer as: If both the statements are true and Statement 2 is the correct explanation of statement 1. If both the statements are True but Statement 2 is not the correct explanation of Statement 1. If Statement 1 is True and Statement 2 is False. If Statement 1 is False and Statement 2 is True. It is given that a≻,b>0,c>0anda+b+c=abc Statement 1: All of the three numbers cannot be less than √3. Statement 2: In ABC. tanA+tanB+tanC=tanAtanBtanC
View SolutionEach question has four choices a,b,c and d out of which only one is correct. Each question contains Statement 1 and Statement 2. Make your answer as: a) If both the statements are true and Statement 2 is the correct explanation of statement 1. b) If both the statements are True but Statement 2 is not the correct explanation of Statement 1. c) If Statement 1 is True and Statement 2 is False. d) If Statement 1 is False and Statement 2 is True. Statement 1: If A,B,C are the angles of a triangle such that angle A is obtuse, then tanBtanC>1. Statement 2: In any triangle, tanA=tanB+tanCtanBtanC−1
View SolutionEach question has four choices a,b,c and d out of which only one is correct. Each question contains Statement 1 and Statement 2. Make your answer as: If both the statements are true and Statement 2 is the correct explanation of statement 1. If both the statements are True but Statement 2 is not the correct explanation of Statement 1. If Statement 1 is True and Statement 2 is False. If Statement 1 is False and Statement 2 is True. If A+B+C=π, then Statement 1: cos2A+cos2B+cos2C has its minimum value 34. Statement 2: Maximum value of cosAcosBcosC is 18
View SolutionEach question has four choices, a,b,c and d, out of which only one is correct. Each question contains STATEMENT 1 and STATEMENT 2. (a) If both the statements are TRUE and STATEMENT 2 is the correct explanation of STATEMENT 1. (b) If both the statements are TRUE but STATEMENT 2 is NOT the correct explanation of STATEMENT 1. (c) If STATEMENT 1 is TRUE and STATEMENT 2 is FALSE. (d) If STATEMENT 1 is FALSE and STATEMENT 2 is TRUE. Statement 1: ∫exsinxdx=ex2(sinx−cosx)+c Statement 2: ∫ex(f(x)+f′(x))dx=exf(x)+c
View SolutionEach question has four choices a, b, c and d, out of which only one is correct. Each question contains STATEMENT 1 and STATEMENT 2. Both the statements are TRUE and STATEMENT 2 is the correct explanation of STATEMENT1. Both the statements are TRUE but STATEMENT 2 is NOT the correct explanation of STATEMENT 1. STATEMENT 1 is TRUE and STATEMENT 2 is FALSE. STATEMENT 1 is FALSE and STATEMENT 2 is TRUE. Statement 1: |adj(adj(adjA))|=|A|n−1^3 , where n is order of matrix A. Statement 2: |adjA|=|A|n.
View SolutionEach question has four choice: a, b, c and d, out of which only one is correct. Each question contains Statement 1 and Statement 2. Find the correct answer. Both the Statements are true but Statement 2 is the correct explanation of Statement 1. Both the Statement are True but Statement 2 is not the correct explanation of Statement 1. Statement 1 is True and Statement 2 is False. Statement 1 is False and Statement 2 is True Statement 1: The lines (a+b)x+(a−2b)y=a are con-current at the point (23,13). Statement 2: The lines x+y−1=0 and x−2y=0 intersect at the point (23,13).
View SolutionEach question has four choices a, b, c and d, out of which only one is correct. Each question contains STATEMENT 1 and STATEMENT 2. (a)Both the statements are TRUE and STATEMENT 2 is the correct explanation of STATEMENT1. (b)Both the statements are TRUE but STATEMENT 2 is NOT the correct explanation of STATEMENT 1. (c) STATEMENT 1 is TRUE and STATEMENT 2 is FALSE. (d) STATEMENT 1 is FALSE and STATEMENT 2 is TRUE. Statement 1: |adj(adj(adjA))|−|A|n−1^3 , where n is order of matrix A. Statement 2: |adjA|=|A|n.
View SolutionEach question has four choice: a, b, c and d, out of which only one is correct. Each question contains Statement 1 and Statement 2. Find the correct answer. Both the Statements are true but Statement 2 is the correct explanation of Statement 1. Both the Statement are True but Statement 2 is not the correct explanation of Statement 1. Statement 1 is True and Statement 2 is False. Statement 1 is False and Statement 2 is True Statement 1: The lines (a+b)x+(a−2b)y=a are con-current at the point (23,13). Statement 2: The lines x+y−1=0 and x−2y=0 intersect at the point (23,13).
View Solution
