Let two non-collinear unit vector ˆa a n d ˆb form an acute angle. A point P moves so that at any time t, the position vector OP(whereO is the origin) is given by ˆacost+ˆbsint.WhenP is farthest from origin O, let M be the length of OPandˆu be the unit vector along OP. Then (a)ˆu=ˆa+ˆb∣∣ˆa+ˆb∣∣andM=(1+ˆa.ˆb)1/2 (b) ˆu=ˆa−ˆb∣∣ˆa−ˆb∣∣andM=(1+ˆa.^)1/2 (c) ˆu=ˆa+ˆb∣∣ˆa+ˆb∣∣andM=(1+2ˆa.ˆb)1/2 (d) ˆu=ˆa−ˆb∣∣ˆa−ˆb∣∣andM=(1+2ˆa.ˆb)1/2Solution in Tamil
Step by step video & image solution for Let two non-collinear unit vector hat a a n d hat b form an acute angle. A point P moves so that at any time t , the position vector O P(w h e r eO is the origin) is given by hat acost+ hat bsintdotW h e nP is farthest from origin O , let M be the length of O Pa n d hat u be the unit vector along O Pdot Then (a) hat u=( hat a+ hat b)/(| hat a+ hat b|)a n dM=(1+ hat adot hat b)^(1//2) (b) hat u=( hat a- hat b)/(| hat a- hat b|)a n dM=(1+ hat adot hat )^(1//2) (c) hat u=( hat a+ hat b)/(| hat a+ hat b|)a n dM=(1+2 hat adot hat b)^(1//2) (d) hat u=( hat a- hat b)/(| hat a- hat b|)a n dM=(1+2 hat adot hat b)^(1//2) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Let two non-collinear unit vector ˆa a n d ˆb form an acute angle. A point P moves so that at any time t, the position vector OP(whereO is the origin) is given by ˆacost+ˆbsint.WhenP is farthest from origin O, let M be the length of OPandˆu be the unit vector along OP. Then (a)ˆu=ˆa+ˆb∣∣ˆa+ˆb∣∣andM=(1+ˆa.ˆb)1/2 (b) ˆu=ˆa−ˆb∣∣ˆa−ˆb∣∣andM=(1+ˆa.^)1/2 (c) ˆu=ˆa+ˆb∣∣ˆa+ˆb∣∣andM=(1+2ˆa.ˆb)1/2 (d) ˆu=ˆa−ˆb∣∣ˆa−ˆb∣∣andM=(1+2ˆa.ˆb)1/2
View SolutionIf →a and →b are two unit vectors and θ is the angle between them, then the unit vector along the angular bisector of →a and →b will be given by
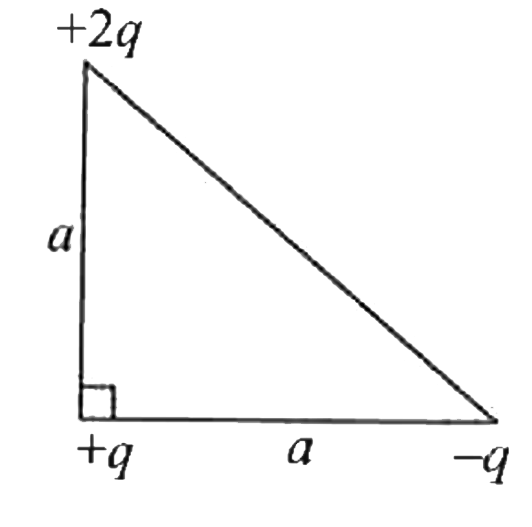
View SolutionThree charges +q,−q,and+2q are placed at the verticles of a right angled triangle (isoceles triangle) as shown. The net electrostatic energy of the configuration is :
View SolutionThe position vectors of the vertices A,B,andC of a triangle are ˆi+ˆj,ˆj+ˆkandˆi+ˆk , respectively. Find the unite vector ˆr lying in the plane of ABC and perpendicular to IA,whereI is the incentre of the triangle.
View SolutionThe position vectors of points AandB w.r.t. the origin are →a=ˆi+3ˆj−2ˆk, →b=3ˆi+ˆj−2ˆk respectively. Determine vector →OP which bisects angle AOB, where P is a point on AB.
View SolutionIf hat a , hat b ,a n d hat c are three unit vectors, such that hat a+ hat b+ hat c is also a unit vector and theta_1,theta_2a n dtheta_3 are angles between the vectors hat a , hat b ; hat b , hat ca n d hat c , hat a respectively, then among theta_1,theta_2,a n dtheta_3dot a. all are acute angles b. all are right angles c. at least one is obtuse angle d. none of these
View SolutionThe position vectors of the vertices A,B,andC of a triangle are ˆi+ˆj,ˆj+ˆkandˆi+ˆk , respectively. Find the unit vector ˆr lying in the plane of ABC and perpendicular to IA,whereI is the incentre of the triangle.
View Solution
