Find the shortest distance between the lines whose vector equations are→r=(4ˆi−ˆj)+λ(ˆi+2ˆj−3ˆk) and →r=(ˆi−ˆj+2ˆk)+μ(ˆi+4ˆj−5ˆk)Solution in Tamil
The correct Answer is:a→qs;b→p;c→s;d→r
The given lines is x=4y+5,z=3y−6
or x−54=y,z+63=y
or x−54=y1=z+63=λ (say)
Any point on the line is of the form (4λ+5,λ,3λ−6).
The distance between (4λ+5,λ,3λ−6)and(5,3,−6) is 3 units (given). Therefore
(4λ+5−5)2+(λ−3)2+(3λ−6+6)2=9
or 16λ2+λ2+9−6λ+9λ2=9
or 26λ2−6λ=0
or λ=0,3/13
The point is (5,0,−6).
b. The equation of the plane containing the lines x−23=y+35=z+57 and parallel to ˆi+4ˆj+7ˆk is
∣∣
∣
∣∣x−2y+3z+5147357∣∣
∣
∣∣=0
or x−2y+z−3=0
Point (−1,−2,0) lies on this plane.
c. The line passing through points A(2,−3,−1)andB(8,−1,2) is x−28−2=y+3−1+3=z+12+1
or x−26=y+32=z+13=λ (say).
Any point on this line is of the form P(6λ+2,2λ−3,3λ−1), whose distance from point
A(2,−3,−1) is 14 units. Therefore,
PA=14
or PA2=(14)2
⇒ (6λ)2+(2λ)2+(3λ)2=196
or 49λ2=196
or λ2=4
or λ=±2
Therefore, the required points are (14, 1, 5) and (−10,−7,−7). The point nearer to the origin is (14,1,5).
d. Any point on line AB,x2=y−23=z−34=λ is M(2λ,3λ+2,4λ+3). Therefore, the direction ratios of PM are 2λ−3,3λ+3and4λ−8.
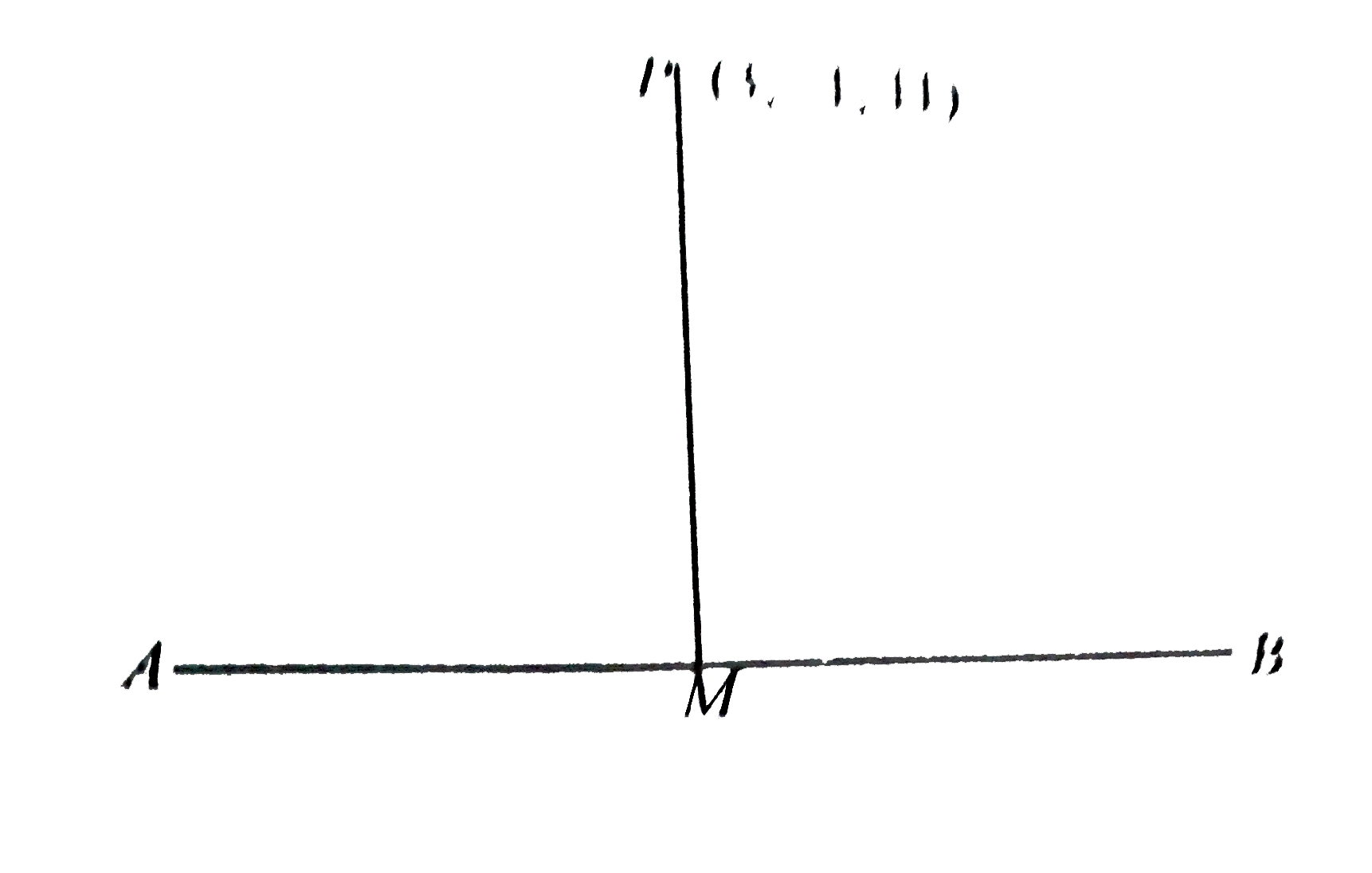
But PM⊥AB
∴ 2(2λ−3)+3(3λ+3)+4(4λ−8)=0
4λ−6+9λ+9+16λ−32=0
29λ−29=0
λ=1
Therefore, foot of the perpendicular is M(2,5,7).
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise (Numerical)10videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - JEE Previous Year26videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGECHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise (Comprehension)12videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGECHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - Question Bank12videos
THREE DIMENSIONAL GEOMETRY
- BOOK - CENGAGECHAPTER - TRIGNOMETRIC RATIOS IDENTITIES AND TRIGNOMETRIC EQUATIONSEXERCISE - Question Bank4videos
TRIGNOMETRIC RATIOS IDENTITIES AND TRIGNOMETRIC EQUATIONS
Similar Questions
Find the number of sphere of radius r touching the coordinate axes.
04:54View Solution(i) Find the equation of the plane passing through the points(2,1,0),(5,0,1)and(4,11). (ii) If P s the point (2,1,6), then the find the point Q such that PQ is perpendicular to the plane in (i) and the midpoint of PQ lies on it.
05:51View SolutionGiven four points A(2,1,0),B(1,0,1),C(3,0,1)andD(0,0,2). Point D lies on a line L orthogonal to the plane determined by the points A, B and C.
The equation of the plane ABC is03:52View SolutionThe equation of the plane which has the property that the point Q(5,4,5) is the reflection of point P(1,2,3) through that plane, is ax+by+cz=d where a,b,c,d∈N. Find the least value of (a+b+c+d).
05:21View SolutionThe number of solution (s) of the equation
√2+cos2x=(sinx+cosx)∈[−π2,π]05:53View SolutionFind the shortest distance between the lines →r=(ˆi+2ˆj+ˆk)+λ(ˆi−ˆj+ˆk)and →r=2ˆi−ˆj−ˆk+μ(2ˆi+ˆj+2ˆk)
06:49View SolutionFind the shortest distance between the lines →r=(ˆi+2ˆj+ˆk)+λ(ˆi−ˆj+ˆk) and →r=(2ˆi−ˆj−ˆk)+μ(2ˆi+ˆj+2ˆk)
06:06View SolutionDetermine whether the following pair of lines intersect or not. (1) →r=ˆi−5ˆj+λ(2ˆi+ˆk);→r=2ˆi−ˆj+μ(ˆi+ˆj−ˆk) (2) →r=ˆi+ˆj−ˆk+λ(3ˆi−ˆj);→r=4ˆi−ˆk+μ(2ˆi+3ˆk)
07:19View SolutionFind the distance between the parallel lines L1 and L2 whose vector equations are
→r=(ˆi+2ˆj+3ˆk)+λ(ˆi−ˆj+ˆk) and (2ˆi−ˆj−ˆk)+μ(ˆi−ˆj+ˆk)05:57View Solutionउन रेखाओ के बीच की न्यूनतम दूरी ज्ञात कीजिए जिनके सदिश समीकरण -
→r=ˆi+ˆj+λ(2ˆi−ˆj+ˆk) तथा →r=2ˆi+ˆj−ˆk+μ(3ˆi−5ˆj+2ˆk) है।06:21View Solutionरेखाओं →r=(ˆi−2ˆj+3ˆk)+λ(−ˆi+ˆj−2ˆk) और →r=(ˆi−ˆj+ˆk)+μ(ˆi+2ˆj−2ˆk) के मध्य न्यूनतम दूरी ज्ञात कीजिए।
05:49View SolutionFind the shortest distance between the lines →r=(ˆi+2ˆj+ˆk)+λ(ˆi−ˆj+ˆk)and →r=2ˆi−ˆj−ˆk+μ(2ˆi+ˆj+2ˆk)
06:03View SolutionFind the shortest distance between lines →r=6ˆi+2ˆj+ˆk+λ(ˆi−2ˆj+2ˆk)and →r=−4ˆi−ˆk+μ(3ˆi−2ˆj−2ˆk).
05:08View SolutionFind the shortest distance between the lines →r=(ˆi+2ˆj+ˆk)+λ(ˆi−ˆj+ˆk) and →r=(2ˆi−ˆj−ˆk)+μ(2ˆi+ˆj+2ˆk)
02:20View SolutionDetermine whether the following pair of lines intersect or not. (1) →r=ˆi−5ˆj+λ(2ˆi+ˆk);→r=2ˆi−ˆj+μ(ˆi+ˆj−ˆk) (2) →r=ˆi+ˆj−ˆk+λ(3ˆi−ˆj);→r=4ˆi−ˆk+μ(2ˆi+3ˆk)
05:43View SolutionFind the shortest distance between the lines →r=(ˆi+2ˆj+ˆk)+λ(ˆi−ˆj+ˆk)and →r=2ˆi−ˆj−ˆk+μ(2ˆi+ˆj+2ˆk)
03:55View Solution

