Question

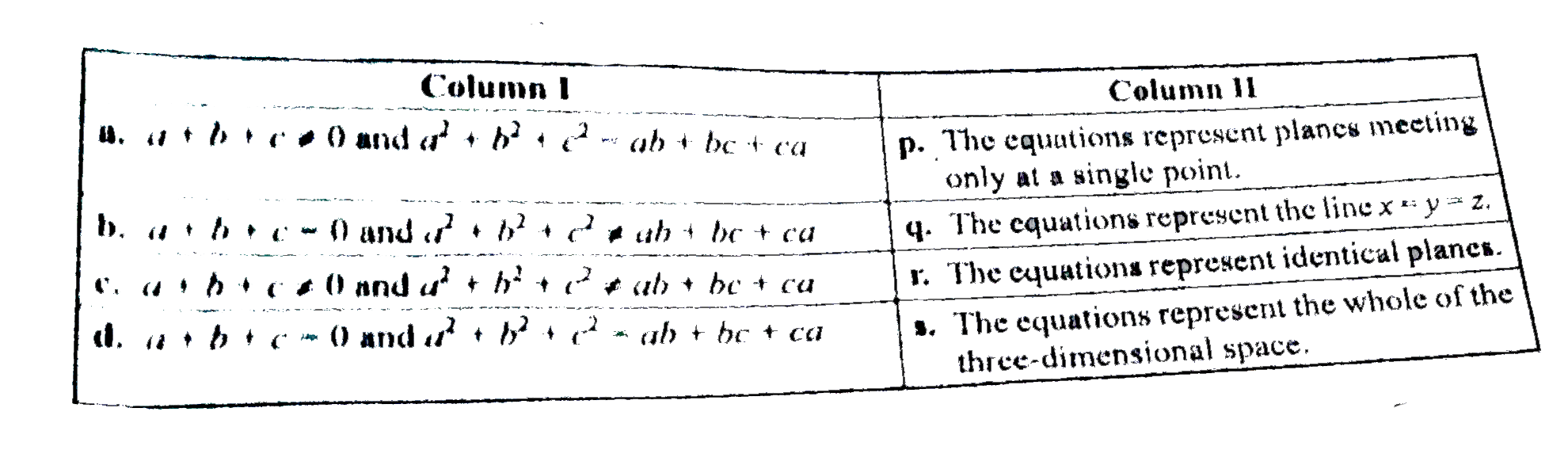
Consider the linear equations $ax+by+cz=0,bx+cy+az=0\text{and}cx+ay+bz=0$.
Match the conditions/expressions in Column I with statements in Column II.

Answer

`a to r; b to q; c to p; d to s`

Explanation

Here we have the determinant of the coefficient matrix of given equation as
`Delta = |{:(a,,b,,c),(b,,c,,a),(c,,a,,b):}|`
`" "= -(a+b+c)(a^(2)+b^(2)+c^(2)-ab-bc-ca)`
`" "= -(1)/(2)(a+b+c)[(a-b)^(2)+ (b-c)^(2)+ (c-a)^(2)]`
a. `a+b+c ne 0`
and `" "a^(2)+b^(2)+c^(2)-ab-bc-ca=0`
or `" "(a-b)^(2)+ (b-c)^(2)+ (c-a)^(2)=0`
or `" "a=b=c`
Therefore, this question represents identical planes.
b. `a+b+c=0`
and `" "a^(2)+b^(2)+c^(2)-ab-bc-ca ne 0`
This means `Delta = 0 and a, b and c` are not all equal. Therefore, all equations are not identical but have infinite solutions. Hence,
`" "ax+by= (a+b)z " "` (using `a+b+c=0`)
and `" "bx+cy= (b+c)z`
`rArr" "(b^(2)-ac)y=(b^(2)-ac)z rArr y=z`
rArr `" "ax+by +cy=0 rArr ax=ay`
`rArr" "x=y=z`
Therefore, the equations represent the line `x=y=z`.
c. `a+b+c ne 0 and a^(2)+b^(2)+c^(2)-ab-bc-ca ne 0`
`rArr" " Delta ne 0` and the equations have only trivial solution, i.e., `x=y=z=0`.
Therefore, the equations represent the planes meeting at a single point, namely origin.
d. `a+b+c=0 and a^(2)+b^(2)+c^(2)-ab-bc-ca=0`
`rArr" " a=b=c and Delta =0 rArr a=b =c =0`
`rArr" "` All equations are satisfied by all `x, y and z`.
`rArr " "` The equations represent the whole of the three-dimensional space.