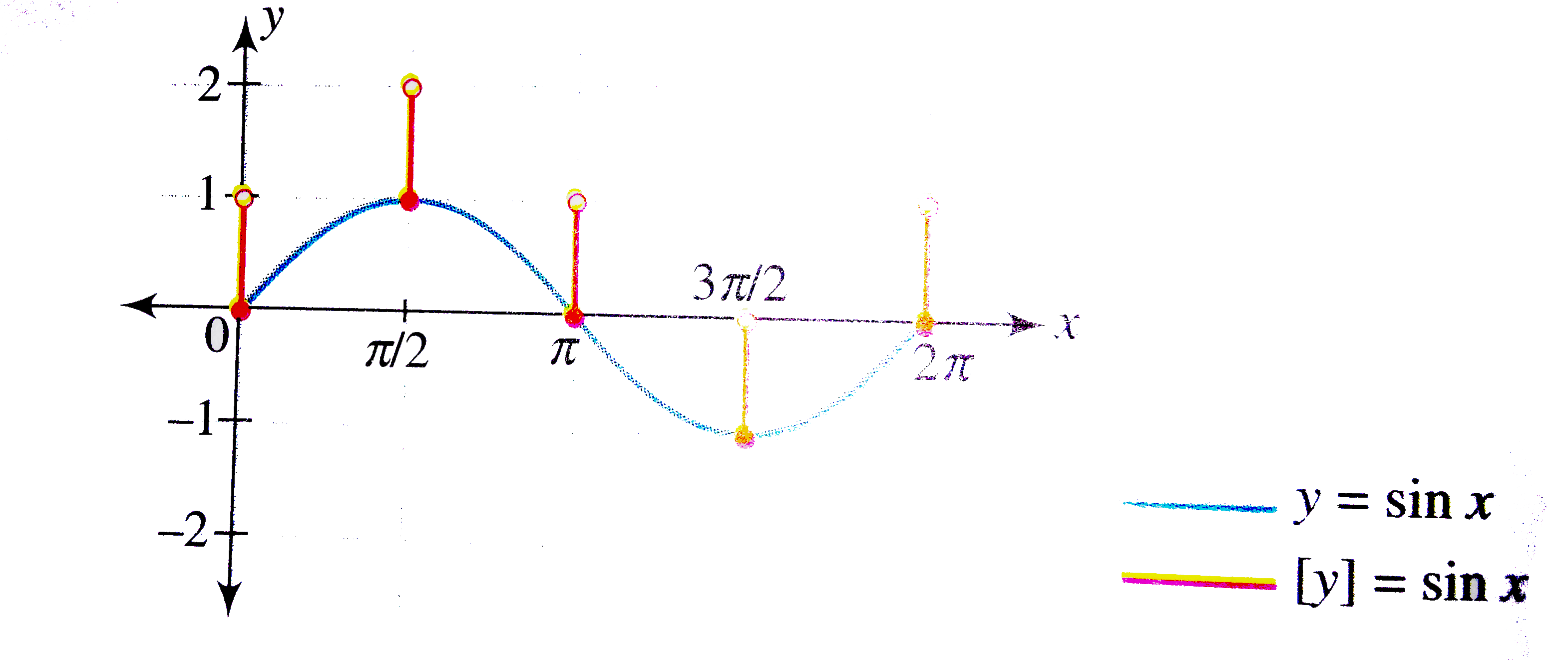
Draw the graph of [y]=sinx,x∈[0,2π] where [⋅] denotes the greatest integer function Solution in Tamil
We have [y] = sin x
This is satisfied by intergral values of sin x only. So
sin x = -1, 0, 1
When sinx=−1,x=(4n−1)π2,n∈Z
And [y]=−1∴−1≤y<0
When sinx=1,x(4n+1)π2,n∈Z
And [y]=1∴1≤y<2
When sinx=0,x=nπ,n∈Z
And [y]=0∴0≤y<1
Hence the graph of the function is as shown in the following figure.
Drawing the graph of g(x)=f(x)sinx
We have g(x)=f(x)sinx.
Since −1≤sinx≤1, we have −f(x)≤f(x)sinx≤f(x).
Thus the graph of g(x)=f(x) sin x lies between th graphs of y=f(x) and y = -f(x). So the draw the graph of y = g(x), we first draw the graph of y = f(x) and y = -f(x) and plot the points of the graph for quadrant angles, i.e. x=...−π,−π/2,0,π/2,π,3π/2,2π...
g(π/2)=f(π/2) sin (π/2)=f(π/2), thus the point (π/2,f(π/2)) lies on the graph of y = f(x)
g(3π/2)=f(3π/2) sin (3π/2)=−f(3π/2), thus the point (3π/2,−f(3π/2)) lies on the graph of y = -f(x).
Similarly, for x=5π/2,9π/2,... points of y = g(x) lie on the graph of y = f(x), and for x=3π/2,7π/2,... points of y = g(x) lie on the graph of y = -f(x).
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise22videos
GRAPHS OF TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise34videos
GRAPHS OF ELEMENTARY FUNCTIONS
- BOOK - CENGAGECHAPTER - HIGHT AND DISTANCE EXERCISE - JEE Previous Year3videos
HIGHT AND DISTANCE
Similar Questions
Draw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
02:52View SolutionDraw the graph of y=1(1/x).
View SolutionA bird is sitting on the top of a vertical pole 20 m high and its elevation from a point O on the ground is 45o . It flies off horizontally straight away from the point O. After one second, the elevation of the bird from O is reduced to 30o . Then the speed (in m/s) of the bird is (1) 40(√2−1) (2) 40(√3−2) (3) 20√2 (4) 20(√3−1)
01:45View SolutionDraw the graph of y=[|x|], where [.] denotes the greatest integer function.
03:50View SolutionDraw the graph of [y]=sinx,x∈[0,2π] where [⋅] denotes the greatest integer function
01:51View SolutionDraw the graph of [y]=cosx,x∈[0,2π], where [⋅] denotes the greatest integer function.
02:27View SolutionIf [sinx]+[√2cosx]=−3,x∈[0,2π], (where ,[.] denotes th greatest integer function ), then
04:33View SolutionDraw the graph of the function y = [x], where [x] denotes the greatest integer not greater than x.
04:42View SolutionDraw the graphs of the following function abs(f(x))=2+sinx x in[0,2pi] [.] denotes the greatest integer function.
07:18View SolutionDraw the graph of y=[|x|], where [.] denotes the greatest integer function.
06:39View SolutionDraw the graph of [y]=sinx,x∈[0,2π] where [⋅] denotes the greatest integer function
03:03View SolutionA point where function f(x)=[sin[x]] is not continuous in (0,2π) [.] denotes the greatest integer ≤x, is
02:43View SolutionIf [sinx]+[√2cosx]=−3,x∈[0,2π], (where ,[.] denotes th greatest integer function ), then
03:03View SolutionThe number of points where f(x) = [sin x + cosx] (where [.] denotes the greatest integer function) x in (0,2pi) is not continuous is
04:24View SolutionDraw the graph of y=[|x|] , where [.] denotes the greatest integer function.
03:28View Solution

