Discuss maxima/minima of f(x)=x1+xtanx,x∈(0,π2)Solution in Tamil
f'(x)=1−x2sec2x(1+xtanx)2
=sec2X(cosx+x)(cosx−x)(1+xtanx)2
Now the sign of the derivative depends on the factor 'cos x - x' only.
To check the sign of 'cos x - x', we draw the graphs of y = x and y = cos x.
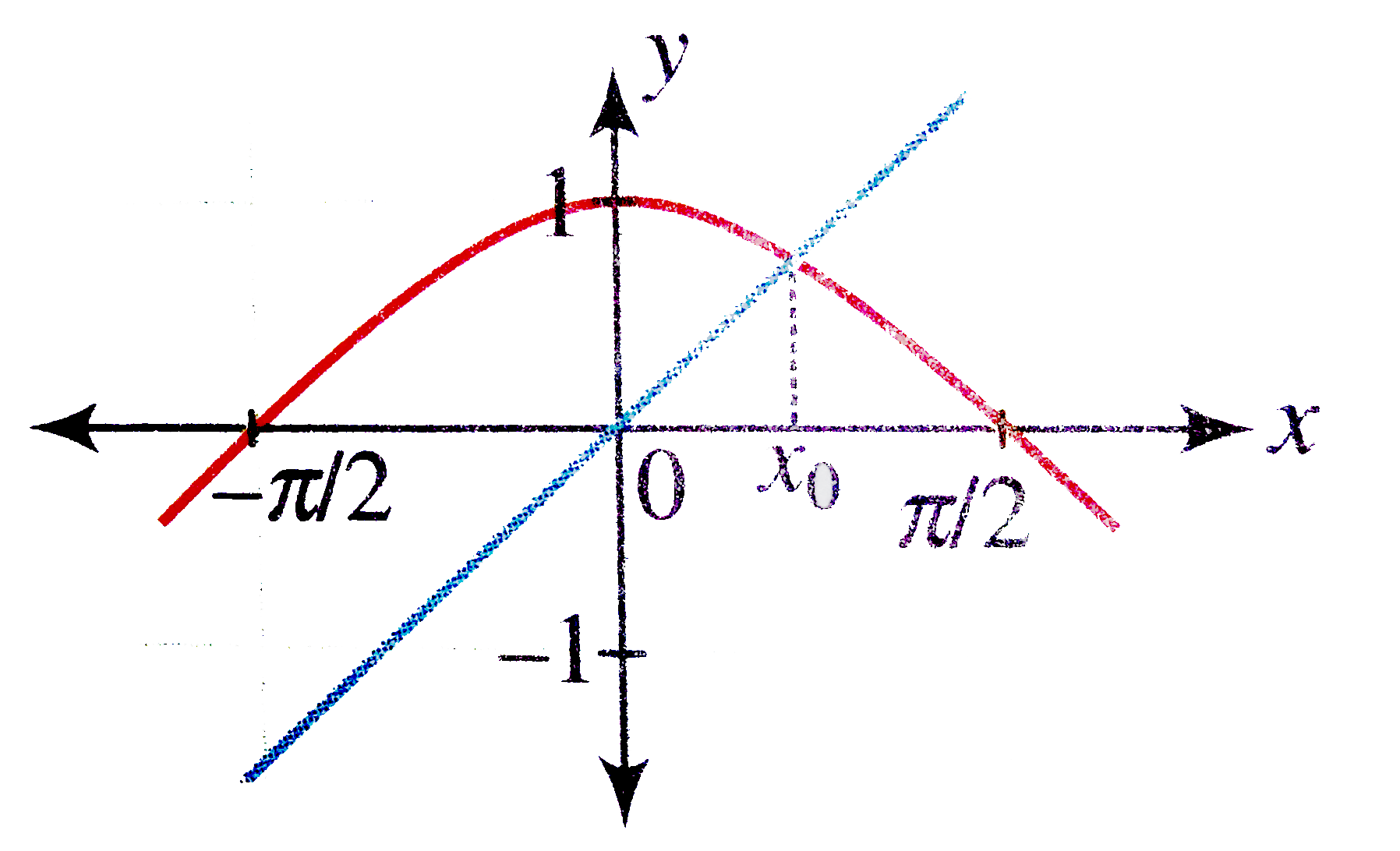
Clearly, f'(x0)=0
and f'(x)>0∀x∈(0,x0)
f'(x)<0∀x∈(x0,π/2)
Thus, x=x0 is the only point of maxima for y = f(x).
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise22videos
GRAPHS OF TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise34videos
GRAPHS OF ELEMENTARY FUNCTIONS
- BOOK - CENGAGECHAPTER - HIGHT AND DISTANCE EXERCISE - JEE Previous Year3videos
HIGHT AND DISTANCE
Similar Questions
Draw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
03:35View SolutionDraw the graph of y=1(1/x).
03:15View SolutionA bird is sitting on the top of a vertical pole 20 m high and its elevation from a point O on the ground is 45o . It flies off horizontally straight away from the point O. After one second, the elevation of the bird from O is reduced to 30o . Then the speed (in m/s) of the bird is (1) 40(√2−1) (2) 40(√3−2) (3) 20√2 (4) 20(√3−1)
03:34View SolutionDiscuss the extrema of f(x)=x1+xtanx,x∈(0,π2)
07:44View SolutionDiscuss the maxima/minima of the function f(x)=4sinx−2x−xcosx2+cosx,0x<2π
View SolutionIf f(x)=(x)/(x+tan x),x belongs to (0,pi/2)(A)f(x) has exactly one point of maxima (C) f(x) is increasing in (0,pi/2))((B)f(x) has exactly one point of minima (D) none of these
03:28View SolutionLet f(x)={x^(2)+x;-1<=x<0 and lambda;x=0 and log_((1)/(2))(x+(1)/(2));0
04:16View SolutionDiscuss maxima/minima of f(x)=x1+xtanx,x∈(0,π2)
04:01View SolutionLet f(x)={6,x≤17−x,x>1 'then for f(x) at x=1 discuss maxima and minima.
01:45View SolutionDiscuss maxima/minima of f(x)=x1+xtanx,x∈(0,π2)
04:32View SolutionDiscuss the global maxima and global minima for f(x)=tan−1x−logex on [1√3,√3]
03:46View SolutionLet f(x)={6,x≤17−x,x>1 'then for f(x) at x=1 discuss maxima and minima.
02:25View SolutionFind the points of local maxima or minima for f(x)= sin 2x−x,x∈(0,π)
01:54View SolutionDiscuss the global maxima and global minima of f(x)=tan−1x−(log)ex in[1√3,√3]
03:21View SolutionLet f(x)=2x3−9x2+12x+6. Discuss the global maxima and minima of f(x)
04:14View Solution

