Question

Solve ${\displaystyle \sin x\succ \frac{1}{2}\text{or}}$
find the domain of ${\displaystyle f\left(x\right)=\frac{1}{\sqrt{1+2\sin x}}}$

Explanation

For f(x) to get defined, we must have 1 + 2 sin `x gt 0` or `sin x gt -(1)/(2)`
As the function sin x has fundamental period `2pi`, it is sufficient to solve the inequality first on the interval of length `2pi`, and then get the solution set by adding numbers of the form `2npi, n in Z`, to each of the solutions obtained on that interval.
Thus, let us solve this inequality on the interval `[-(pi)/(2), (3pi)/(2)]`.

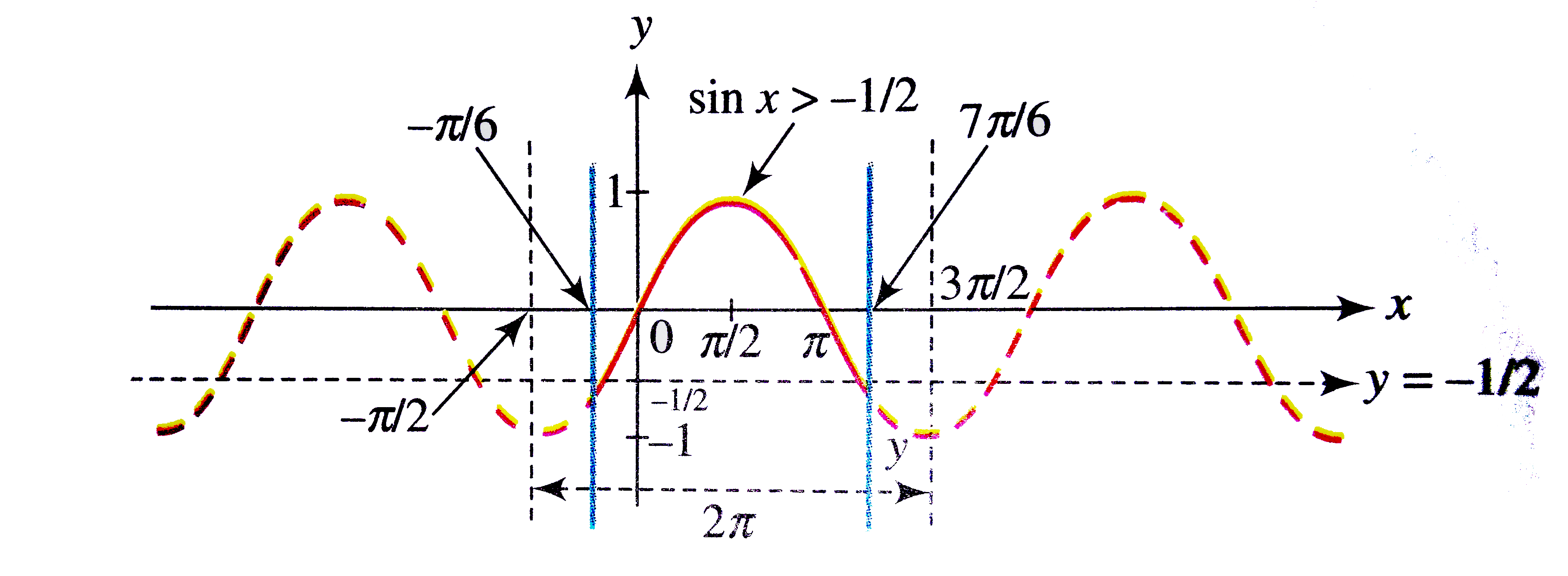
From the above figure, `sin x gt - (1)/(2)` when `- (pi)/(6) lt x lt (7pi)/(6)`
Thus, on generalizing, the above solution becomes `2npi - (pi)/(6) lt x lt 2npi + (7pi)/(6) , n in Z`