Draw the graph of y=3x−x31−3x2 and hence the graph of y=tan−1.3x−x31−3x2.Solution in Tamil

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

We have y=f(x)=3x−x31−3x2, which is an odd function.
Domain of the function is R−{±1√3}
1. y-intercept
f(0)=0
So the graph cuts the y-axis at (0,0).
2. x-intercept (zeros)
Put y=0 or 3x−x3=0∴x=0,±√3
So the graph meets the x-axis at (0,0) and (±√3,0).
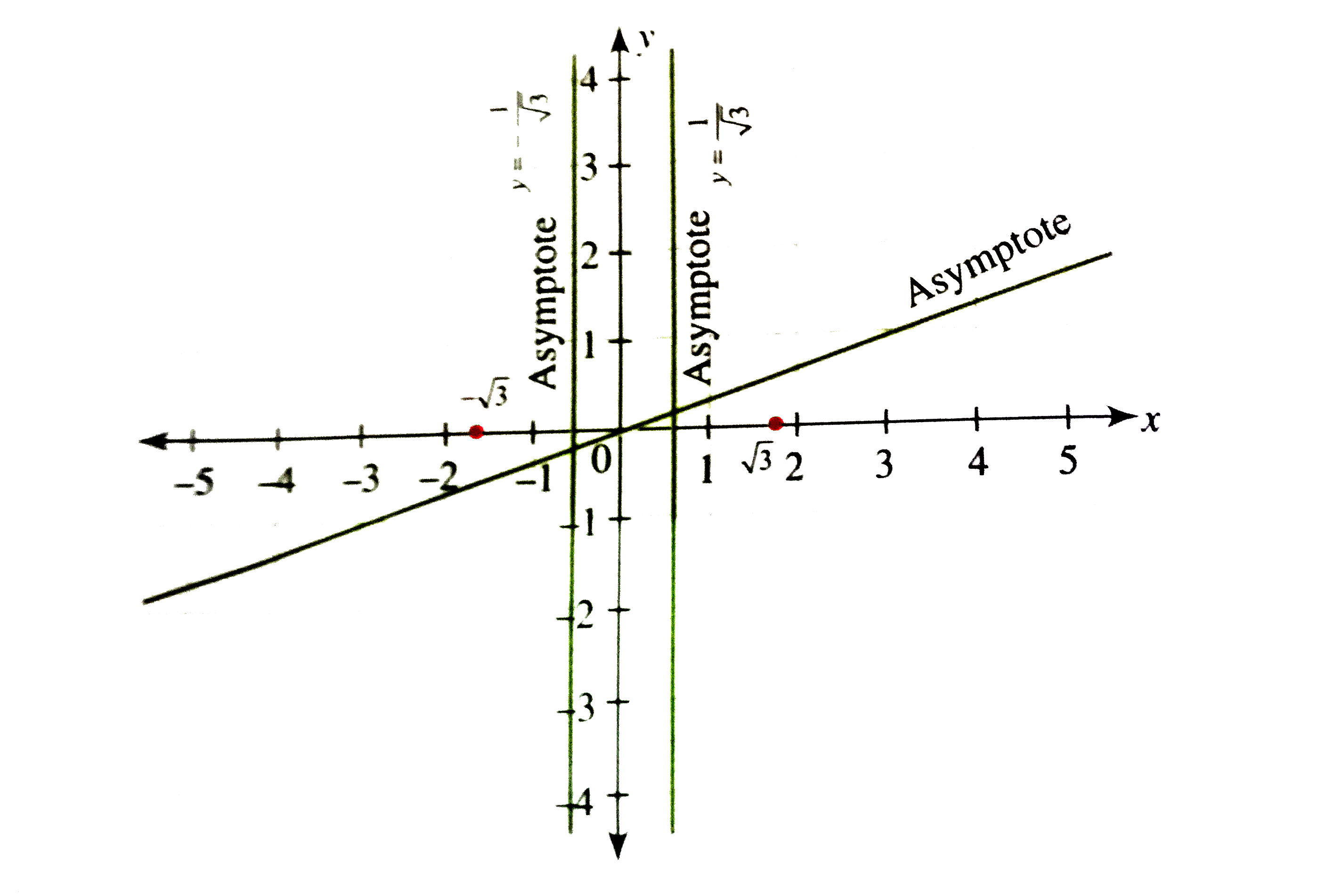
3. Asymptotes
Vertical asymptotes
Clearly , the graph has vertical asymptote x=±1√3, where the denominator becomes zero.
Horizontal asymptotes
Clearly, the graph has no horizontal asymptote as the degree of the numerator is higher than the degree of the numerator is higher than the degree of the denominator.
Oblique asymptotes ltbr> y=x3−3x3x2−1=13x−−89x3x2−1
Hence the oblique asymptote is y=13x.
Thus, important points ans lines are as shown in the following figure.
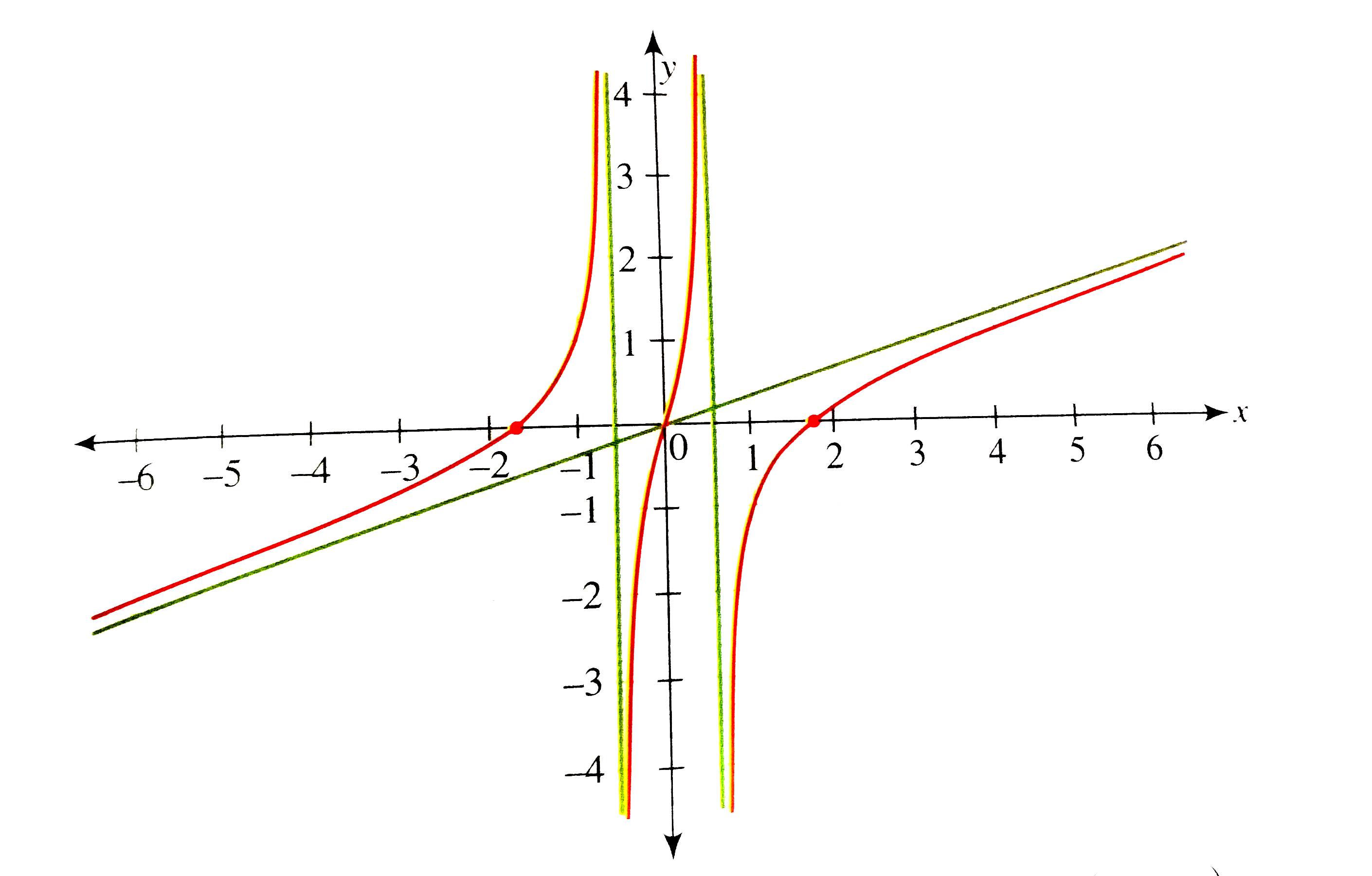
ocr_image
4. Monotinicity/Extremum
f'(x)=(3−3x2)(1−3x2)+6x(3x−x3)(1−3x2)2
=3(x2+1)2(1−3x2)2>0
Hence the function is increasing throughout.
limx→−1−√33x−x31−3x2=∞ and limx→−1+√33x−x31−3x2=−∞
f(x) approaches asymptote y=x/3 as x→−∞.
Thus, in (−∞,−1√3), f(x) increases from '−∞' to '∞' intersecting the x-axis at (−√3,0)
limx→−1+√33x−x31−3x2=−∞ and limx→√3−3x−x31−3x2=∞
Thus, in (−1√3,1√3), f(x) increases from '−∞' to '∞' intersecting the x-axis at (0,0).
limx→√3+3x−x31−3x2=−∞ and limx→∞3x−x31−3x2=∞
Thus, in (1√3,∞),f(x) increases from '−∞' to '∞' intersecting the x-axis at (√3,0).
f(x) approaches asymptote y=x/3 as x→∞.
From the above discussion, the graph of y=f(x) can be drawn as follows.
ocr_image
Now in each of the intervals (−∞,−1√3),(−1√3,1√3),(1√3,∞),f(x) takes values (−∞,∞).
So in each of the intervals, tan−1.3x−x31−3x2 takes values (−π2,π2).
So the graph of g(x)=tan−1.3x−x31−3x2 can be drawn as follows.
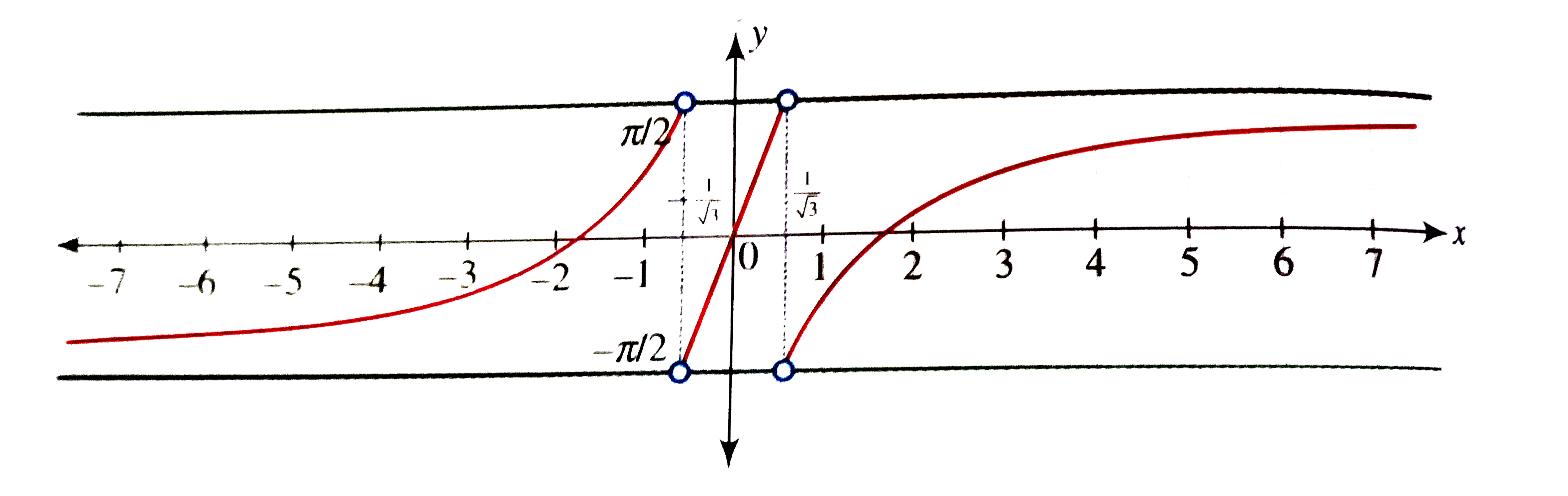
ocr_image
Here y=±π2 are asymptotes.

Updated on:21/07/2023
|
Class 12MATHSQUADRATIC EQUATIONS

Topper's Solved these Questions

Similar Questions