In ABC,C=600andB=450. Line joining vertex A of triangle and its circumcenter (O) meets the side BC∈D Find the ratio BD:DC Find the ratio AO:ODSolution in Tamil
In the figure, ∠AOC=2B
⇒∠DOC=π−2B
Similarly, ∠BOD=π−2C
Now, BDCD=12BD.OP12CD.OP
=Area of ΔBODArea of ΔCOD
=12OB.ODsin∠BOD12OC.ODsin∠COD
=sin(π−2C)sin(π−2B)=sin2Csin2B
=sin120∘sin90∘
=√32
Now in ΔOPB,OP=OBcosA=RcosA
Also ∠POD=∠POC−∠DOC
=A−(π−2B)
=π−B−C−π+2B
=B−C
Now in ΔOPD,OD=POcos(B−C)=RcosAcos(B−C)
⇒AOOD=RRcosAcos(B−C)
=cos(B−C)cosA=cos15∘cos75∘=cos15∘sin15∘=2√3

Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Exercise 5.112videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGECHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Exercise 5.28videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGECHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (MATRIX MATCH TYPE )1videos
PROGRESSION AND SERIES
- BOOK - CENGAGECHAPTER - PROPERTIES OF TRIANGLE, HEIGHT AND DISTANCEEXERCISE - Question Bank15videos
PROPERTIES OF TRIANGLE, HEIGHT AND DISTANCE
Similar Questions
Find the value of a2+b2+c2R2 in any right-angled triangle.
06:04View SolutionIf the sides of a triangle are a, b and √a2+ab+b2, then find the greatest angle
01:27View SolutionIt is given that in a group of 4 students, the probability of 2 students not having the same birthday is 0.893. What is the probability that the 2 students have the same birthday?
03:34View SolutionThe area of a right triangle is 6864 sq. units. If the ratio of its legs is 143:24, then the value of r is ______
05:42View SolutionIn ABC,C=600andB=450. Line joining vertex A of triangle and its circumcenter (O) meets the side BC∈D Find the ratio BD:DC Find the ratio AO:OD
02:10View SolutionLine segments AB and CD intersect at O such that AC∣∣DB. If ∠CAB=450and ∠CDB=550, then ∠BOD= 1000 (b) 800 (c) 900 (d) 1350
05:30View SolutionThe diagonals of a rectangle ABCD meet at O . If ∠BOC=44∘, find ∠OAD
08:19View SolutionA point O inside a rectangle ABCD is joined to the vertices. Prove that the sum of the areas of a pair of opposite triangles so formed is equal to the sum of the other pair of triangles. Given: A rectangle ABCDandO is a point inside it. OA,OB,OCandOD have been joined. To Prove: ar(AOD)+ar(BOC)=ar(AOB)+ar(COD)
07:31View SolutionABCD IS A PARALLELOGRAM AND O is any point in its interior. Prove that: ar (AOB)+ ar (COD)= ar (BOC)+ ar(AOD) ar (AOB)+ar (COD)=12 ar(^(gm)ABCD) Given: A parallelogram ABCD and O is a point in its interior. To Prove: ar ( AOB)+ ar(COD)=ar (BOC)+ar(AOD)
03:31View SolutionIn Figure, ABCD is a parallelogram in which ∠DAO=400, ∠BAO=350 and ∠COD=650. Find: ∠ABO (ii) ∠ODC (iii) ∠ACB (iv) ∠CBD
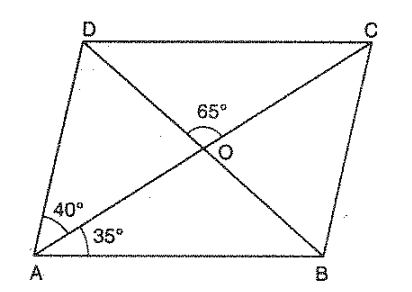 03:13View Solution
03:13View SolutionIn ABC,C=600andB=450. Line joining vertex A of triangle and its circumcenter (O) meets the side BC in D Find the ratio BD:DC and AO:OD
01:51View SolutionIn ABC,C=600andB=450. Line joining vertex A of triangle and its circumcenter (O) meets the side BC∈D Find the ratio BD:DC Find the ratio AO:OD
02:34View SolutionO is any point inside a triangle ABC . The bisector of ∠AOB,∠BOC and ∠COA meet the sides AB,BC and CA in point D,EandF respectively. Show that AD⋅BE⋅CF=DB⋅EC⋅FA
02:35View SolutionLine segments AB and CD intersect at O such that AC=DB. If ∠CAB=450and ∠CDB=550, then ∠BOD= (a)1000 (b) 800 (c) 900 (d) 1350
02:22View SolutionIn ABC, if ∠A=400and ∠B=600. Determine the longest and shortest sides of the triangle.
05:53View SolutionIn a parallelogram ABCD diagonals AC and BD intersect at O and AC=6.8 cm and BD=5.6cm . Find the measures of OC and OD .
04:46View Solution

