Given a right triangle ABC with ∠A=900. Let D be the mid-point of BC. If the inradii of the triangle ABD and ACD are r1andr2 then find the range of r1r2.Solution in Tamil
In the figure, D is mid-point of BC. From the geometry, AD=BD=CD=a/2
In ΔABD,r1=Δ1s1
⇒r1=12(a2)ha2+a2c2
⇒r1=ah2(a+c)

In ΔACD,r2=Δ2s2
⇒r2=12(a2)ha2+a2+b2
⇒r2=ah2(a+b)
⇒r1r2=a+ba+c
=2RsinA+2RsinB2RsinA+2RsinC
=1+sinB1+sinC (as A=90∘)
=1+sinB1+cosB (as C=90∘−B)
When B aproaches to 90∘,r1r2 approaches to 2
When B approaches to 0∘,r1r2 approaches to 12
∴r1r2∈(12,2)
Topper's Solved these Questions
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGECHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Exercise 5.1
12 Videos
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGECHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Exercise 5.2
8 Videos
- PROGRESSION AND SERIESBOOK - CENGAGECHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (MATRIX MATCH TYPE )
1 Videos
- PROPERTIES OF TRIANGLE, HEIGHT AND DISTANCEBOOK - CENGAGECHAPTER - PROPERTIES OF TRIANGLE, HEIGHT AND DISTANCEEXERCISE - Question Bank
15 Videos
Similar Questions
Find the value of a2+b2+c2R2 in any right-angled triangle.
View SolutionIf the sides of a triangle are a, b and √a2+ab+b2, then find the greatest angle
View SolutionIt is given that in a group of 4 students, the probability of 2 students not having the same birthday is 0.893. What is the probability that the 2 students have the same birthday?
View SolutionThe area of a right triangle is 6864 sq. units. If the ratio of its legs is 143:24, then the value of r is ______
View SolutionIn right angled triangle ABC,/_A is right angle.AD is perpendicular to the hypotenuse BC.Prove that (Delta ABC)/(Delta ACD)=(BC^(2))/(AC^(2))
View SolutionGiven a right triangle with /_A=90^(@). Let M be the mid-point of BC.If the inradii of the triangle ABM and ACM are r_(1) and r_(2) then find the range of (r_(1))/(r_(2))
View SolutionIn ΔABC,r1r+r2r3bc=
View SolutionIn ΔABC,r3+r1r2r1+r2=
View SolutionIn triangle ABC, if D is point on BC such that ∠ADC=∠ACD then AD=?
View SolutionΔABC में BC पर एक बिंदु D इस प्रकार है कि BD:DC=m:n है |
सिद्ध करें कि ar(ΔABD):ar(ΔACD)=m:n
View SolutionIf in a triangle (1−r1r2)(1−r1r3)=2 then the triangle is right angled (b) isosceles equilateral (d) none of these
View SolutionGiven a right triangle with ∠A=90∘. Let M be the mid-point of BC. If the radii of the triangle ABMandACM are r1andr2 then find the range of r1r2.
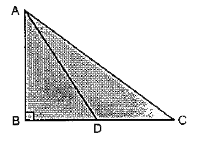
View SolutionIn the given figure, triangle ABC is a right triangle with ∠B=90∘andD is mid-point of side BC. Prove that :
AC2=AD2+3CD2
View SolutionABC is a right triangle angleBAC=90^@ And D, BC is a point on the hypotenuse, BD = AD. Prove that D, BC is the midpoint of the arm.
View SolutionRight triangle /_\ABC Of /_A= 90^@ . AD perpendicular to the hypotenuse BC. Prove that (/_\ABC)/(/_\ACD)=(BC^2)/(AC^2)
View SolutionIf in a triangle (1−r1r2)(1−r1r3)=2 then the triangle is right angled (b) isosceles equilateral (d) none of these
View Solution
