Question

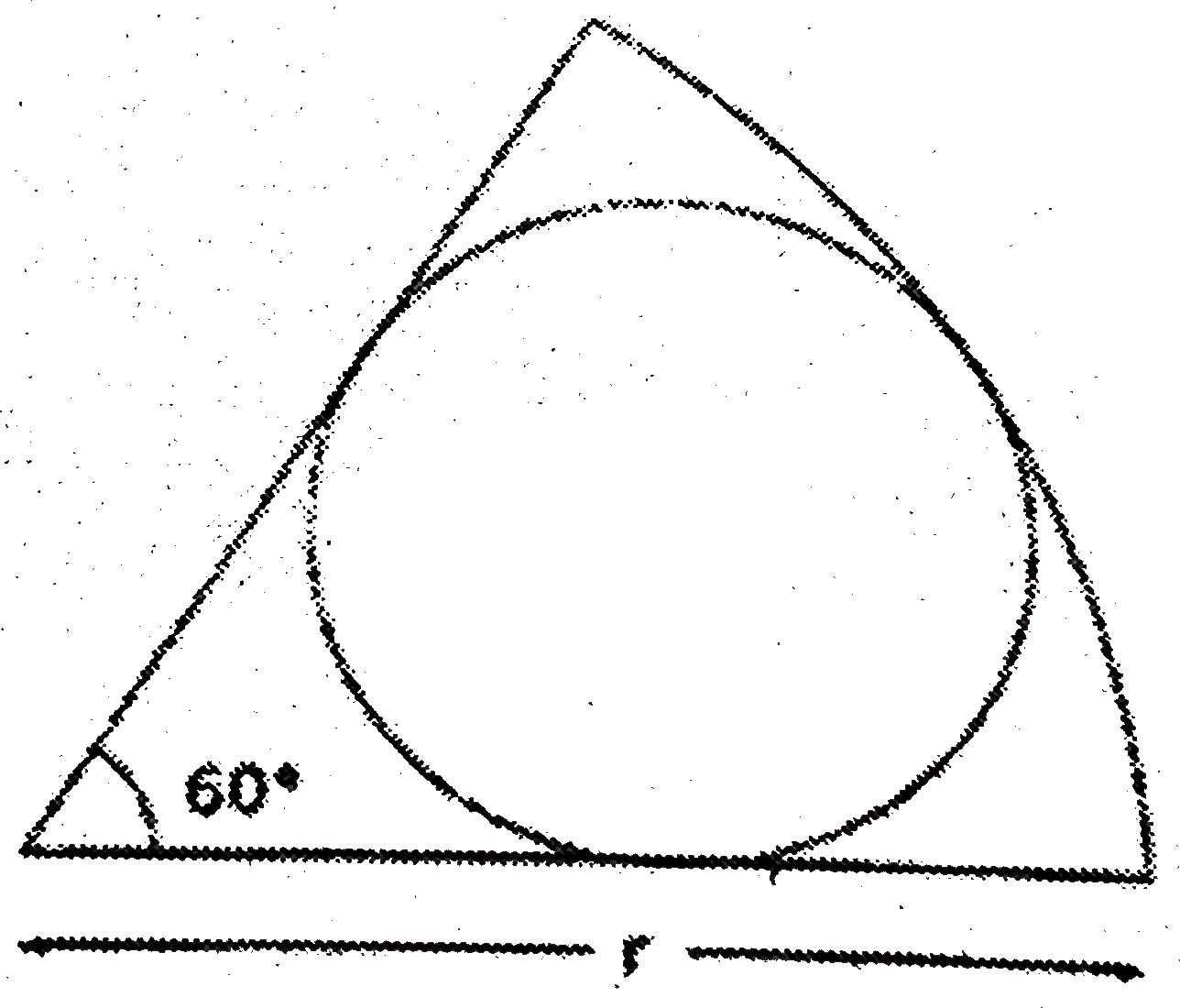
If inside a big circle exactly $n(n\le 3)$
small circles, each of radius $r,$
can be drawn in such a way that each small circle touches the big
circle and also touches both its adjacent small circles, then the radius of
big circle is
$r(1+\cos ec\frac{\pi }{n})$
(b) $\left(\frac{1+\frac{\tan \pi }{n}}{\frac{\cos \pi }{\pi }}\right)$

$r[1+\cos ec\frac{2\pi }{n}]$
(d) $\frac{r{[\frac{\sin \pi }{2n}+\frac{\cos \pi }{2n}]}^2}{\frac{\sin \pi }{n}}$

Options

A. `r(1 + cosec.(pi)/(n))`
B. `((1 + tan pi//n)/(cos pi//n))`
C. `r[1 + cosec.(2pi)/(n)]`
D. `(r[sin.(pi)/(2n) + cos.(2pi)/(n)]^(2))/(sin pi//n)`

Answer

A::D

Explanation

If R is radius of bigger circle, then `sin ((pi)/(n)) = (r)/(R-r)`
or `R = r (1 + cosec((pi)/(n))) =(r["sin"(pi)/(2n) + "cos"(pi)/(2n)]^(2))/("sin"(pi)/(n))`