Find the length of the chord which subtends an angle of $120^{\circ}$ at the centre of the circle of radius 6 cm.

Text Solution

Verified by Experts

let the chord be AB and O be the circle.

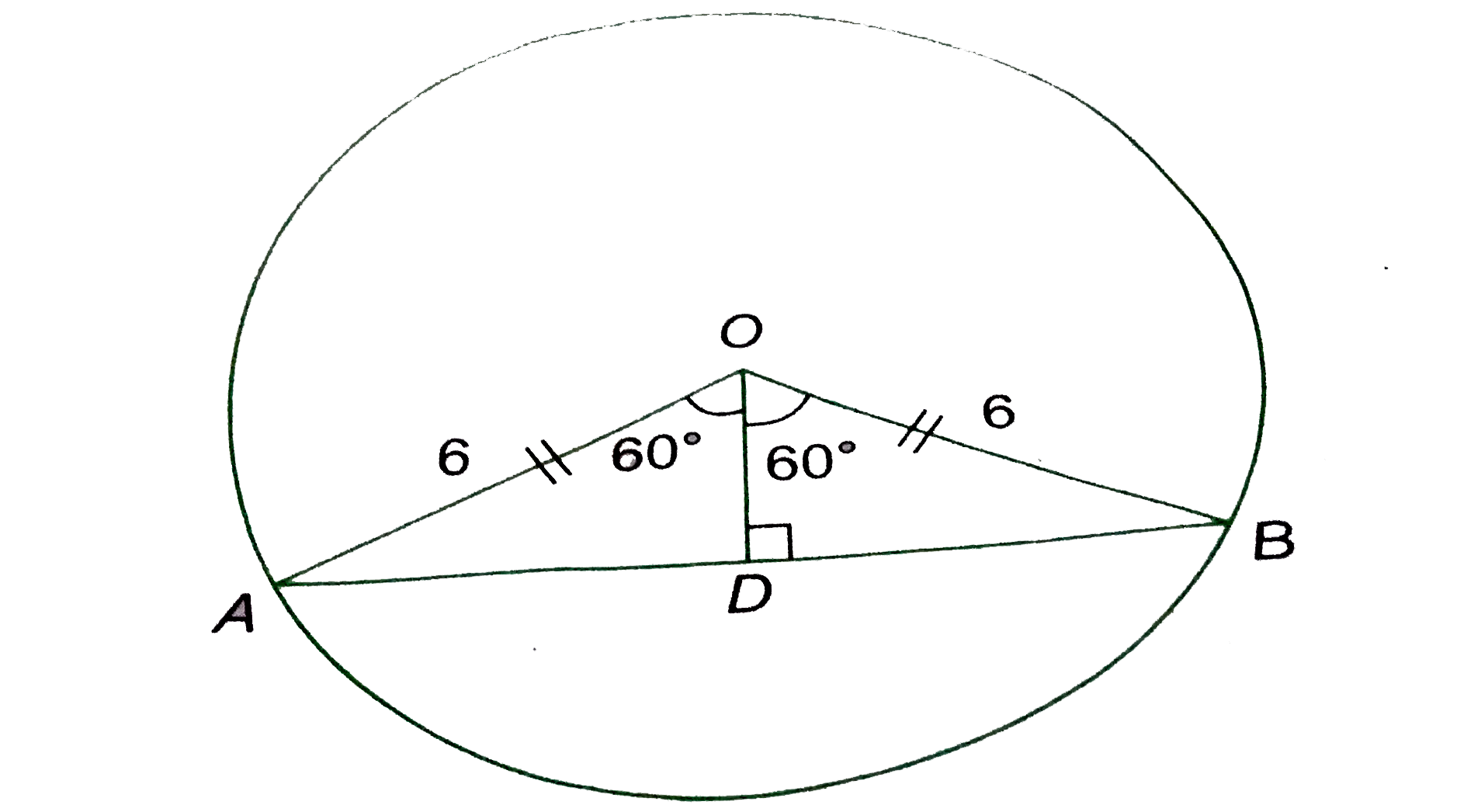
In the figure, D is the mid-point of AB and OD od perpendicular to AB.
$O A = O B = 6 c m, ∠ A O B = 120^{\circ}$ .
Clearly, triangles AOD and BOD are congruent.
$∴ ∠ A O D = ∠ B O D = \frac{1}{2} ∠ A O B = 60^{\circ}$
In $Δ A O D, {sin 60}^{\circ} = \frac{A D}{A O} = \frac{A D}{6}$
$∴ A D = 6 {sin 60}^{\circ} = 6 \times s q e r \frac{3}{2} = 3 \sqrt{3}$
$∴ A B = 2 \times A D = 6 \sqrt{3}$

|

Updated on:21/07/2023

Class 12 MATHS TRIGONOMETRIC FUNCTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
What is the length of the chord of a unit circle which subtends an angle at the centre ?
A $sin (\frac{θ}{2})$
B $cos (\frac{θ}{2})$
C $2 sin (\frac{θ}{2})$
D $2 cos (\frac{θ}{2})$

CENGAGE-TRIGONOMETRIC FUNCTIONS -SINGLE CORRECT ANSWER TYPE

Home
Ask Doubt
Profile