In a regular tetrahedron, let θ be angle between any edge and a face not containing the edge. Then the value of cos2θ is Solution in Tamil
In the figure we have regular pyramid.
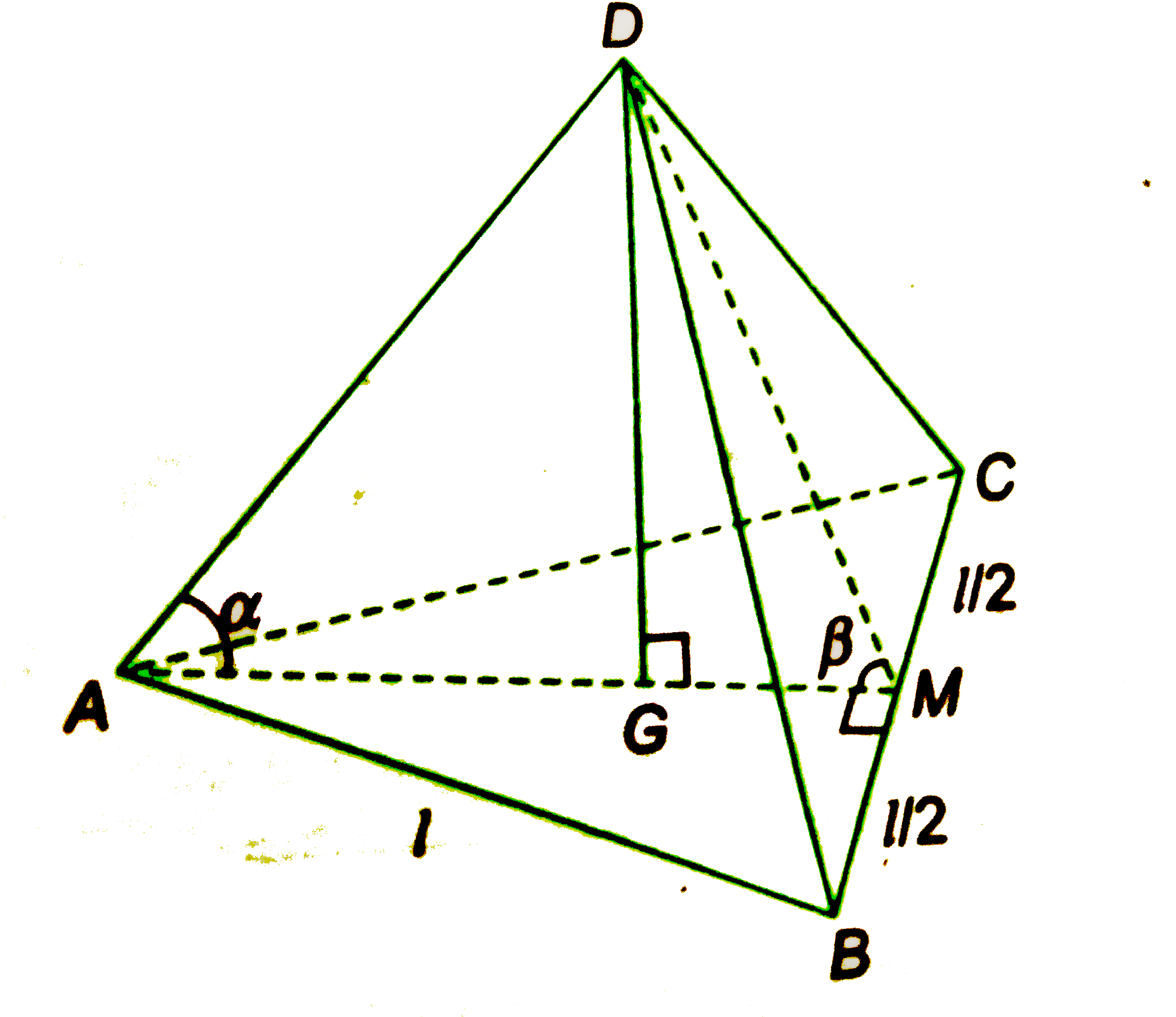
All the edges have length l cm.
So, each face of the pyramid is an equilateral triangle.
From vertex D drop perpendicular to meet opposite face ABC at point G, which is the centre of the triangle ABC.
Here, G is also centroid of triangle ABC as it an equailateral triangle.
Clearly, AG=23AM
In triangle AMB,AM=ABsin60∘=√32lcm
∴AG=23×AM=23×√32l=12√3lcm
In triangle AGD, using Pythagoras, we get
AD2=AG2+GD2
∴l2=13l2+GD2
∴GD=√231cm
For angle between any edge and face not containing that edge, consider edge AD and face ABC.
Angle between them is α,which is angle between AD and AM.
In triangle AGD, cosα=AGAD=1√3ll=1√3
Angle between two faces ABC and BCD is the angle between AM and DM, which is β.
In triangle DGM,cosβ=GMDM=13
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise 2.18videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise 2.28videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGECHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Matrix Match Type)1videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGECHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Matrix Match Type1videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
Similar Questions
Two sides of a parallelgram are 12 cm and 8 cm. If one of the interior angles is 135∘, then find area of the parallelogram.
14:06View SolutionProve that sinx−cosx+1sinx+cos−1=secx+tanx.
07:02View SolutionFind the derivative of y=tan−12x3
03:58View SolutionRefer to the following diagram :

Column IColumn IIa.Collinear vectorsp.→ab.Coinitial vectorsq.→bc.Equal vectorsr.→cd.Unlike vectors (same initial point)s.→d08:36View SolutionLet k be the length of any edge of a regular tetrahedron (a tetrahedron whose edges are equal in length is called a regular tetrahedron). Show that the angle between any edge and a face not containing the edge iscos−1(1/√3) .
07:32View SolutionIn a regular tetrahedron,prove that angle theta between any edge and the face not containing that edge is given by cos theta=(1)/(sqrt(3))
View SolutionIf volume of a regular tetrahedron of edge length k is V and shortest distance between any pair of opposite edges of same regular tetrahedron is d,then find the value of (d^(3))/(V)
04:01View SolutionComprehesion-I
Let k be the length of any edge of a regular tetrahedron (all edges are equal in length). The angle between a line and a plane is equal to the complement of the angle between the line and the normal to the plane whereas the angle between two plane is equal to the angle between the normals. Let O be the origin and A,B and C vertices with position vectors →a,→b and →c respectively of the regular tetrahedron.
The angle between any edge and a face not containing the edge is05:10View SolutionComprehesion-I
Let k be the length of any edge of a regular tetrahedron (all edges are equal in length). The angle between a line and a plane is equal to the complement of the angle between the line and the normal to the plane whereas the angle between two plane is equal to the angle between the normals. Let O be the origin and A,B and C vertices with position vectors →a,→b and →c respectively of the regular tetrahedron.
The angle between any two faces is05:10View SolutionLet OABC be a regular tetrahedron, then angle between edges OA and BC is:
05:47View SolutionIf K is the length of any edge of a regular tetrahedron, then the distance of any vertex from the opposite face is
05:23View SolutionLet k be the length of any edge of a regular tetrahedron (a tetrahedron whose edges are equal in length is called a regular tetrahedron). Show that the angel between any edge and a face not containing the edge iscos−1(1/√3) .
08:49View SolutionLet k be the length of any edge of a regular tetrahedron (a tetrahedron whose edges are equal in length is called a regular tetrahedron). Show that the angel between any edge and a face not containing the edge iscos−1(1/√3) .
07:39View SolutionThe angle between any two opposite pair of edges of a regular tetrahedron (i.e all faces are equilateral triangles) is
07:18View SolutionIn a regular tetrahedron, let θ be angle between any edge and a face not containing the edge. Then the value of cos2θ is
11:32View SolutionComprehesion-I
Let k be the length of any edge of a regular tetrahedron (all edges are equal in length). The angle between a line and a plane is equal to the complement of the angle between the line and the normal to the plane whereas the angle between two plane is equal to the angle between the normals. Let O be the origin and A,B and C vertices with position vectors →a,→b and →c respectively of the regular tetrahedron.
The angle between any edge and a face not containing the edge is04:31View Solution

