Solve tanx>cotx, where x∈[0,2π].
Text Solution
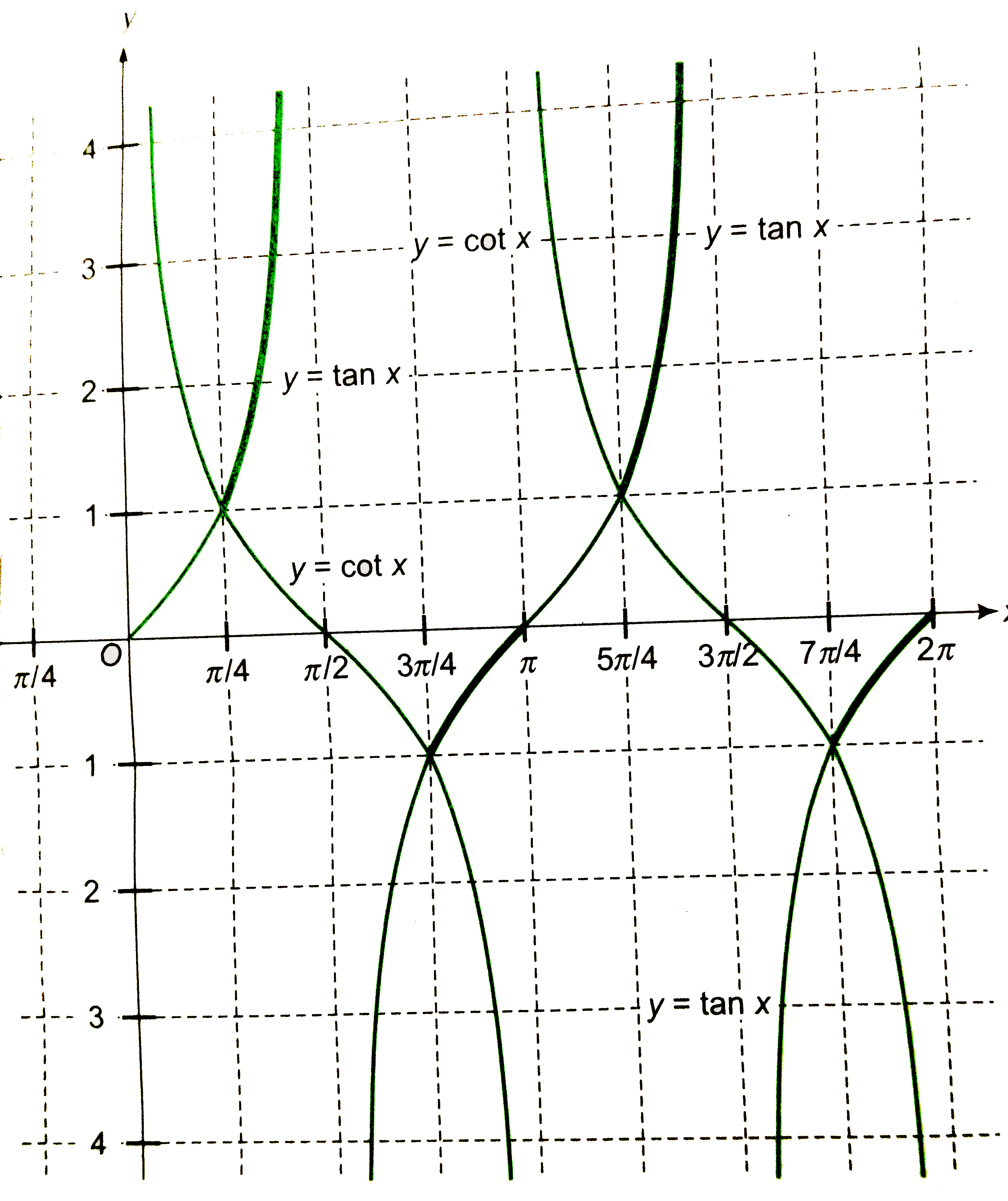
We find the tanx≥cotx.
Therefore, the value of tan x is more than the value of cot x.
That is, the values of x for which graph of y=tanx is above the graph of y=cotx.
From the graph, it is clear that
x∈(π/4,π/2)∪(3π/4,π)∪(5π/4,3π/2)∪(7π/4,2π).
|
Topper's Solved these Questions
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise 2.1
8 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise 2.2
8 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGECHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Matrix Match Type)
1 Videos
- TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASBOOK - CENGAGECHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Matrix Match Type
1 Videos
Similar Questions
Knowledge Check
If tanx=3cotx then x=?
A45∘B60∘C30∘D15∘Let f(x)=max{tanx,cotx}. Then the number of roots of the equation f(x)=12 in (0,2π) is
A0B1C2D4
Find the number of roots of the equation tan(x+π6)=2tanx,forx∈(0,3π).
View SolutionComprehension 2 (Q.No 4 to 6)
It is known that
√tanx+√cotx=⎧⎪ ⎪⎨⎪ ⎪⎩√sinx√cosx+√cosx√sinxif0<x<π2√−sinx√cosx+√−cosx√−sinxifπ<x<3π2
ddx(√tanx−√cotx)=12(√tanx+√cotx)(tanx+cotx),∀∈(0,π,2)∪(π,3π2) and ddx(√tanx+√cotx)=12(√tanx−√cotx)(tanx+cotx),∀x∈(0,π2)∪(π,3π2).
Value of the integral I=∫(√tanx+√cotx) dx, where x∈(0,π2), is
View SolutionUsing mean value theorem, prove that tanx>x for all x(0,π2).
View SolutionThe area bounded by the curve f(x)=||tanx+cotx|−|tanx−cotx∣∣ between the lines x=0,x=π2 and the X-axis is
View SolutionSolve tanx+tan2x+tan3x=tanxtan2xtan3x,x∈[0,π]
View SolutionThe number of solution of the equation 2(tanx−sinx)+3(cotx−cosx)+5=0xε[0,2π]
View Solution
