The number of solutions of the equation $[y + [y]] = 2 cos x,$ where $y = \frac{1}{3} [sin x + [sin x + [sin x]]]$ (where [.] denotes the greatest integer function) is

A

4

B

2

C

3

D

0

Text Solution

Verified by Experts

The correct Answer is:D

$[y + [y]] = 2 cos x$
$or [y] + [y] = 2 cos x (∵ [x + n] = [x] + n if n \in I)$
$or 2 [y] = 2 cos x or [y] = cos x (1)$
Also, $y = \frac{1}{3} [sin x + [sin x + [sin x]]]$
$= \frac{1}{3} (3 [sin x])$
$= [sin x] (2)$
From (1) and (2),
$[[sin x]] = cos x$
$or [sin x] = cos x$

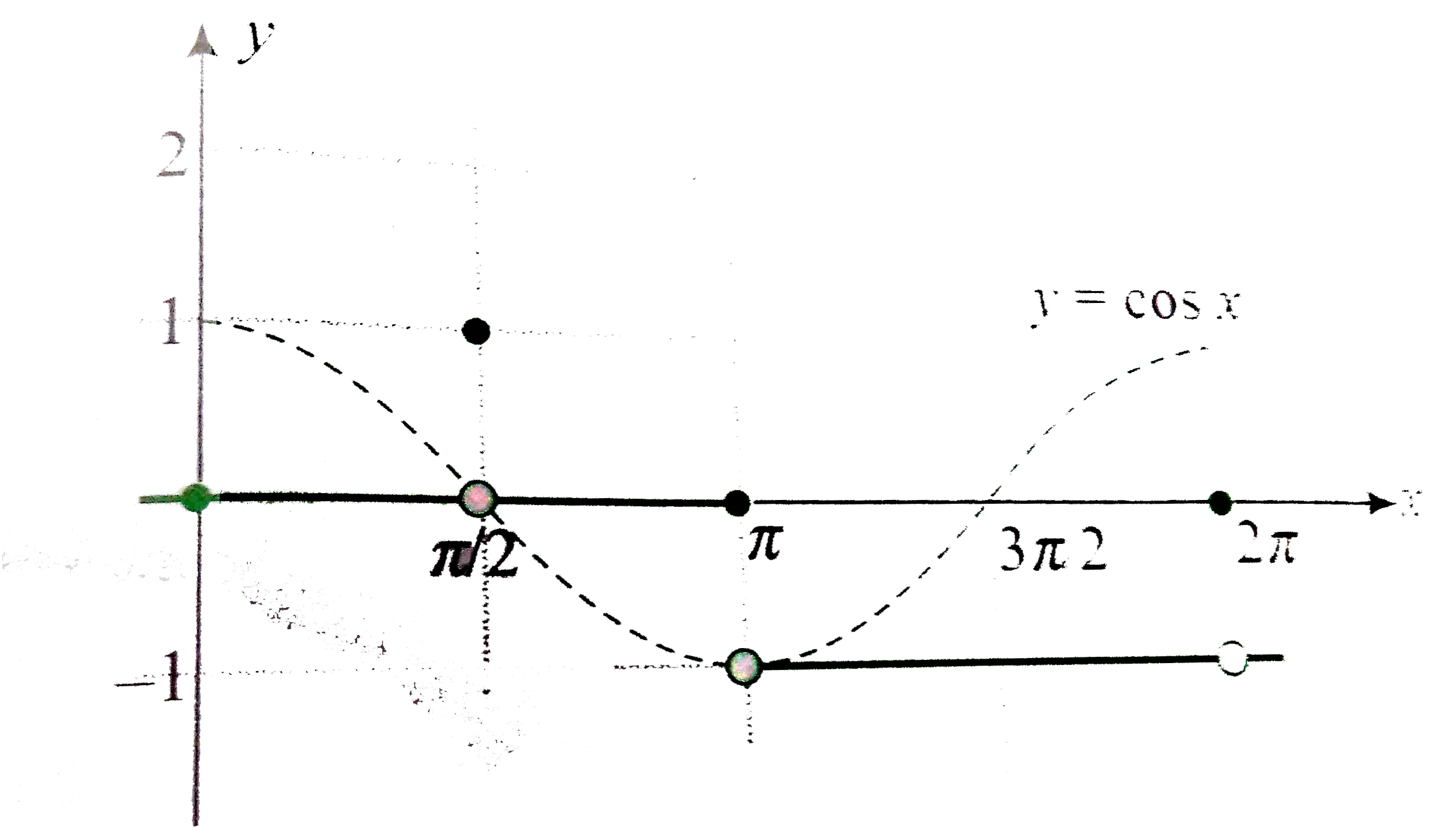
The number of solutions is 0.

|

Updated on:21/07/2023

Class 12 MATHS RELATIONS AND FUNCTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Number of solutions of the equations $y = \frac{1}{3} [sin x + [sin x + [sin x]]] and [y + [y]] = 2 cos x$ , where [.] denotes the greatest integer function is:
A0
B1
C2
Dinfinite
Question 2 - Select One
Domain of the function $f (x) = \frac{1}{[sin x - 1]}$ (where [.] denotes the greatest integer function) is
A $R ~ {2 n π + \frac{π}{2}, n \in l}$
B $R ~ {n π + \frac{π}{2}, n \in l)}$
C $[2 n π, 2 n π + \frac{π}{2}]$
Dnone of these
Question 3 - Select One
The range of the function $f (x) = [sin x + cos x]$ (where $[x]$ denotes the greatest integer function) is $f (x) \in$ :
A[-2,1]
B{-2,-1,0,1}
C{-1,1}
D{-2,0,-1}

CENGAGE-RELATIONS AND FUNCTIONS-Exercise (Single)

Home
Ask Doubt
Profile