Let f:R→R be a function satisfying f(2−x)=f(2+x)andf(20−x)=f(x)∀x∈R. For this function f, answer the following.
If f(2)≠f(6), then the Solution in Tamil
fundamental period of f(x) is 1
fundamental period of f(x) may be 1
period of f(x) cannot be 1
fundamental period of f(x) is 8
The correct Answer is:C
f(2−x)=f(2+x) (1)
Replace x by 2−x. Then f(x)=f(4−x) (2)
Also, given f(20−x)=f(x) (3)
From (1) and (2), f(4−x)=f(20−x).
Replace x by 4−x. Then f(x)=f(x+16).
Hence, the period of f(x) is 16.
If 1 is a period, then f(x)=f(x+1)∀x∈R
orf(2)=f(3)=f(4)=f(5)=f(6)
which contradicts the given hypotheses that f(2)≠f(6).
Therefore, 1 cannot be period of f(x).
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise (Matrix)9videos
RELATIONS AND FUNCTIONS
- BOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise (Numerical)31videos
RELATIONS AND FUNCTIONS
- BOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise (Multiple)27videos
RELATIONS AND FUNCTIONS
- BOOK - CENGAGECHAPTER - Quadratic Equations, Inequalities, Modulus and LogarithmsEXERCISE - Question Bank28videos
Quadratic Equations, Inequalities, Modulus and Logarithms
- BOOK - CENGAGECHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.311videos
SCALER TRIPLE PRODUCTS
Similar Questions
The function f(x) is defined on the interval [0, 1]. Now, match the following lists:
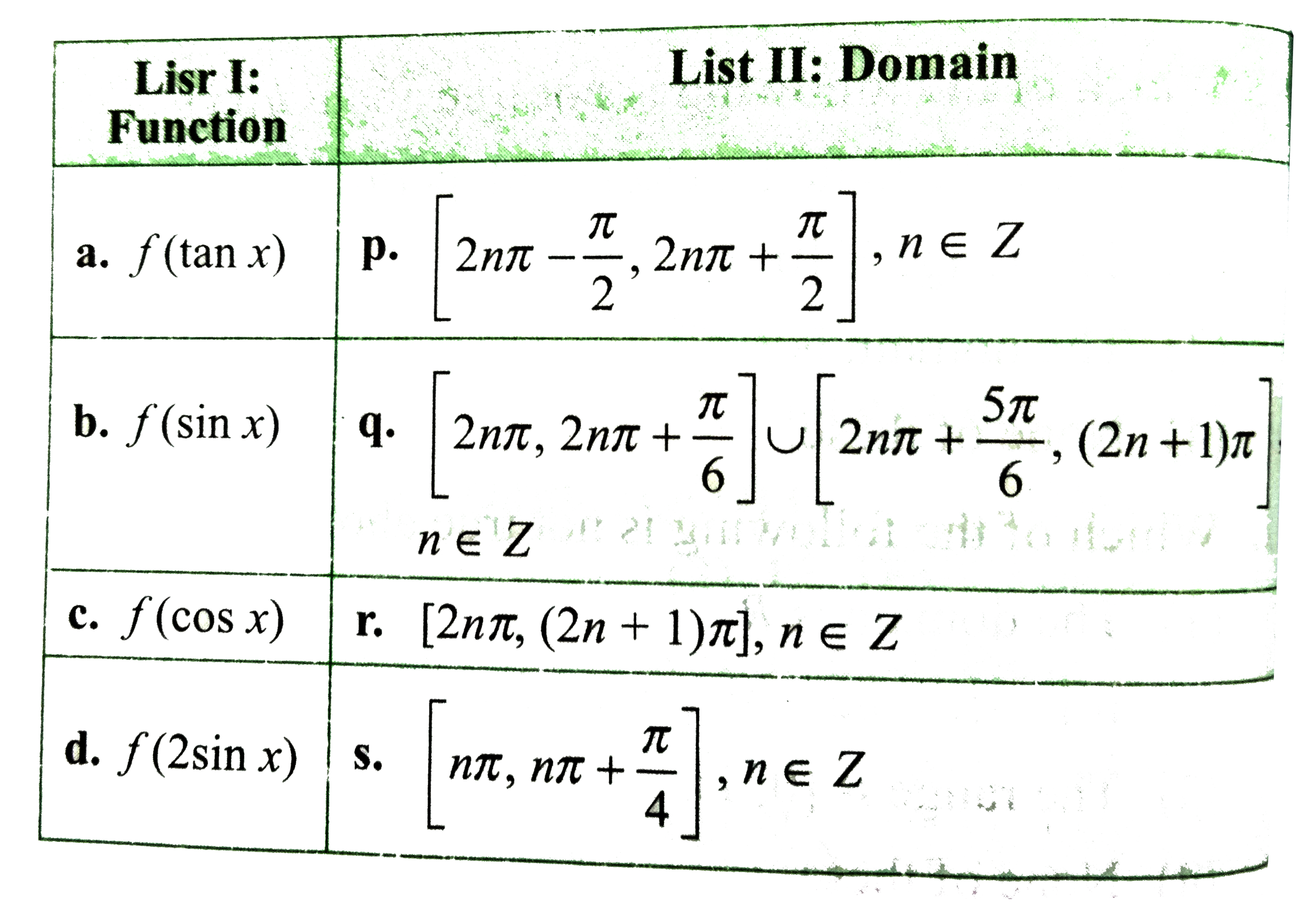 02:51View Solution
02:51View SolutionLet f be a real-valued invertible function such that f(−32xx−2)=5x−2,x≠2. Then value of f−1(13) is________
05:00View SolutionLet f(x)=sec−1[1+cos2x], where [.] denotes the greatest integer function. Then the range of f(x) is
01:50View SolutionLet a,b,c,d be positive integers such that (log)ab=32and(log)cd=54. If (a−c)=9, then find the value of (b−d).
View SolutionNumber of integral value(s) of λ for which vectors x2ˆi−ˆj+xˆk,(λ−1)ˆi−2λˆj+ˆk and ˆi−ˆj+ˆk, in the order from right-handed system ∀x ∈R, is
03:33View SolutionLet f:R rarr R be a function such that f(x+y)=f(x)+f(y),AA x,y in R
02:47View SolutionA function f:R rarr R^(+) satisfies f(x+y)=f(x)f(y)AA x in R If f'(0)=2 then f'(x)=
02:13View SolutionLet f:R rarr R be a differential function satisfy f(x)=f(x-y)f(y)AA x,y in R and f'(0)=a,f'(2)=b then f'(-2)=
01:44View SolutionLet f(x) be a function satisfying f(x)f(x+2)=10∀x∈R, then
01:58View SolutionLet f:R→R be a function satisfying f(2−x)=f(2+x)andf(20−x)=f(x)∀x∈R. For this function f, answer the following.
The graph of y=f(x) is not symmetrial about04:14View SolutionLet f:R→R be a function satisfying f(2−x)=f(2+x)andf(20−x)=f(x)∀x∈R. For this function f, answer the following.
If f(2)≠f(6), then the04:14View SolutionA function f : R→R Satisfies the following conditions
(i) f(x)≠0∀x∈R
(ii) f(x+y)=f(x)f(y)∀x,y,∈R
(iii) f(x) is differentiable
(iv ) f'(0) =2
The derivative of f(x) satisfies the equation07:44View SolutionLet f:R→R be a function satisfying f(2−x)=f(2+x)andf(20−x)=f(x)∀x∈R. For this function f, answer the following.
The graph of y=f(x) is not symmetrial about08:57View SolutionLet f:R→R be a function satisfying f(2−x)=f(2+x)andf(20−x)=f(x)∀x∈R. For this function f, answer the following.
If f(2)≠f(6), then the03:33View SolutionLet f:R→R be a function satisfying f(2−x)=f(2+x)andf(20−x)=f(x)∀x∈R.For this functions f. answer the following
The graph of y = f(x) is not symmetrical about01:21View Solution

