The maximum distance of the centre of the ellipse from the chord of contact of mutually perpendicular tangents of the ellipse is Solution in Tamil
The correct Answer is:C
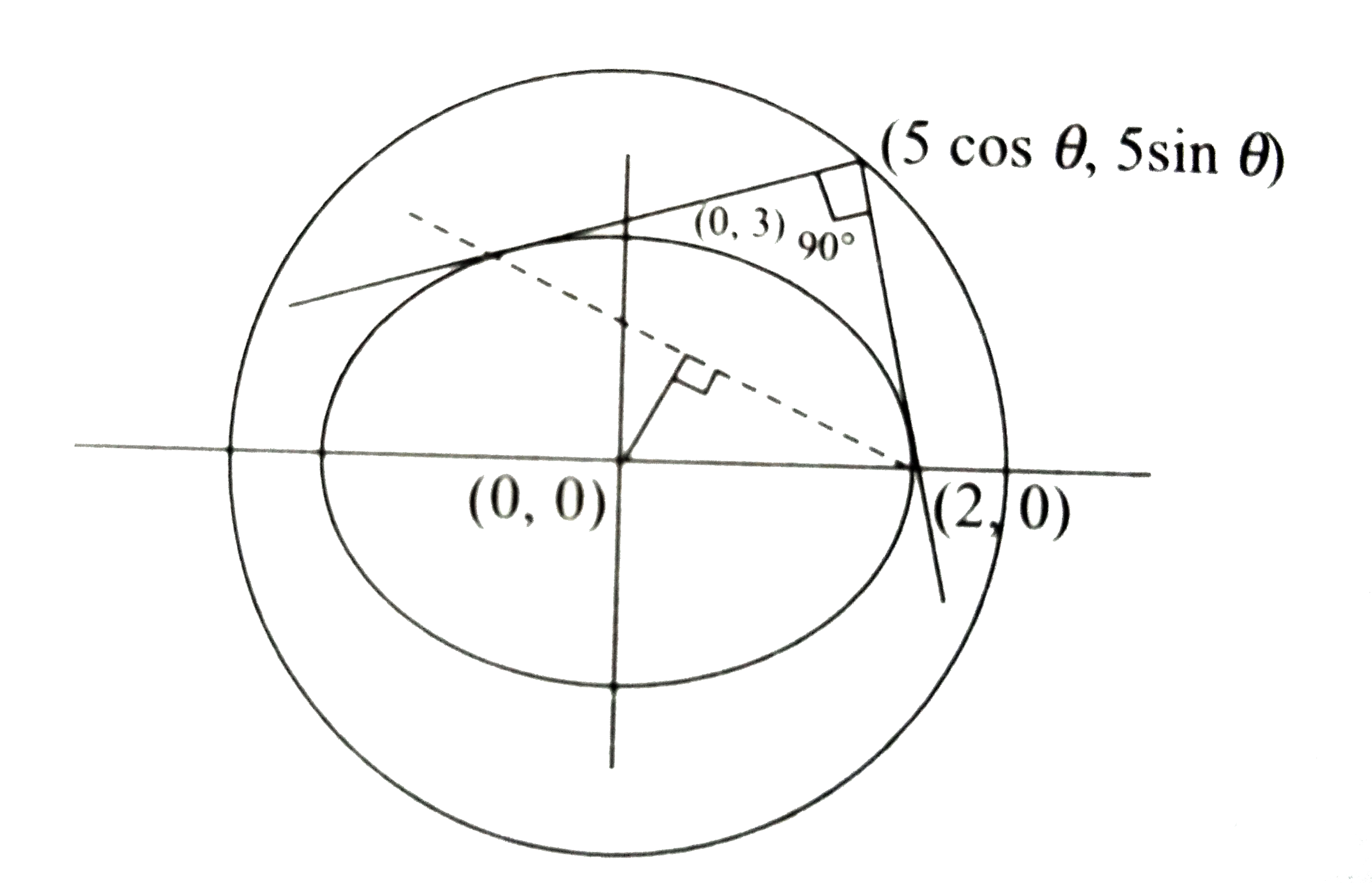
Perpendicular tangents meet on director circle , Any point on this circle is
Equation of chord of contact of ellipse w.r.t this point is
Distance from center
For maximum value and
Maximum distance
Topper's Solved these Questions
Similar Questions
In triangle ABC,a=4 and b=c=2√2. A point P moves within the triangle such that the square of its distance from BC is half the area of rectangle contained by its distance from the other two sides. If D be the centre of locus of P, then
View SolutionLet a,b>0 and →α=(→ia+4ˆjb+bˆk) and →β=bˆi+aˆj+1bˆk, then the maximum value of 305+→α.→β is
View SolutionLet any double ordinate PNP' of the hyperbola x225−y216=1 be produced on both sides to meet the asymptotes in QandQ' . Then PQ.P′Q is equal to 25 (b) 16 (c) 41 (d) none of these
View SolutionFrom the point A(4,3), tangent are drawn to the ellipse x216+y29=1 to touch the ellipse at B and C.EF is a tangent to the ellipse parallel to line BC and towards point A. Then find the distance of A from EF.
View SolutionThe maximum distance of the centre of the ellipse (x^(2))/(81)+(y^(2))/(25)=1 from a normal to the ellipse is
View SolutionNumber of points on the ellipse x225+y216=1 from which pair of perpendicular tangents are drawn to the ellipse x216+y29=1 is
View SolutionTwo perpendicular tangents to the ellipse x216+y29=1 are such that the auxiliary circle intercepts chord of length l1 and l2 on these tangents, then :
View SolutionThe locus of the point of intersection of perpendicular tangents to the ellipse (x−1)216+(y−2)29=1 is
View SolutionIf two points are taken on the mirror axis of the ellipse x225+y29=1 at the same distance from the centre as the foci, then the sum of the sequares of the perpendicular distance from these points on any tangent to the ellipse is
View SolutionThe locus of point intersection of perpendicular tangents of ellipse ((x-1)^(2))/(16)+((y-1)^(2))/(9)=1 is
View SolutionFor the ellipse x216+y29=1 .If The maximum distance from the centre of the ellipse to the chord of contact of mutually perpendicular tangents is λ then 5λ−10=
View SolutionThe locus of the foot of the perpendicular drawn from the centre on any tangent to the ellipse x225+y216=1 is:
View SolutionProve that the chords of contact of pairs of perpendicular tangents to the ellipse x2a2+y2b2=1 touch another fixed ellipse.
View SolutionProve that the chords of contact of pairs of perpendicular tangents to the ellipse x2a2+y2b2=1 touch another fixed ellipse.
View SolutionThe product of the perpendiculars from the foci of the ellipse x216+y24=1 onto any tangent to the ellipse is
View SolutionNumber of points on the ellipse x225+y216=1 from which pair of perpendicular tangents are drawn to the ellipse x216+y29=1 is
View Solution
