The greatest integral value of a such that √9−a2+2x−x2≥√16−x2 for at least one positive value of x is (a) 3 (b) 4 (c) 6 (d) 7Solution in Tamil
8
7
6
4
The correct Answer is:2
y=√9−a2+2ax−x2
y=√16−x2
⇒y2+(x−a)2=9
⇒y2+x2=16
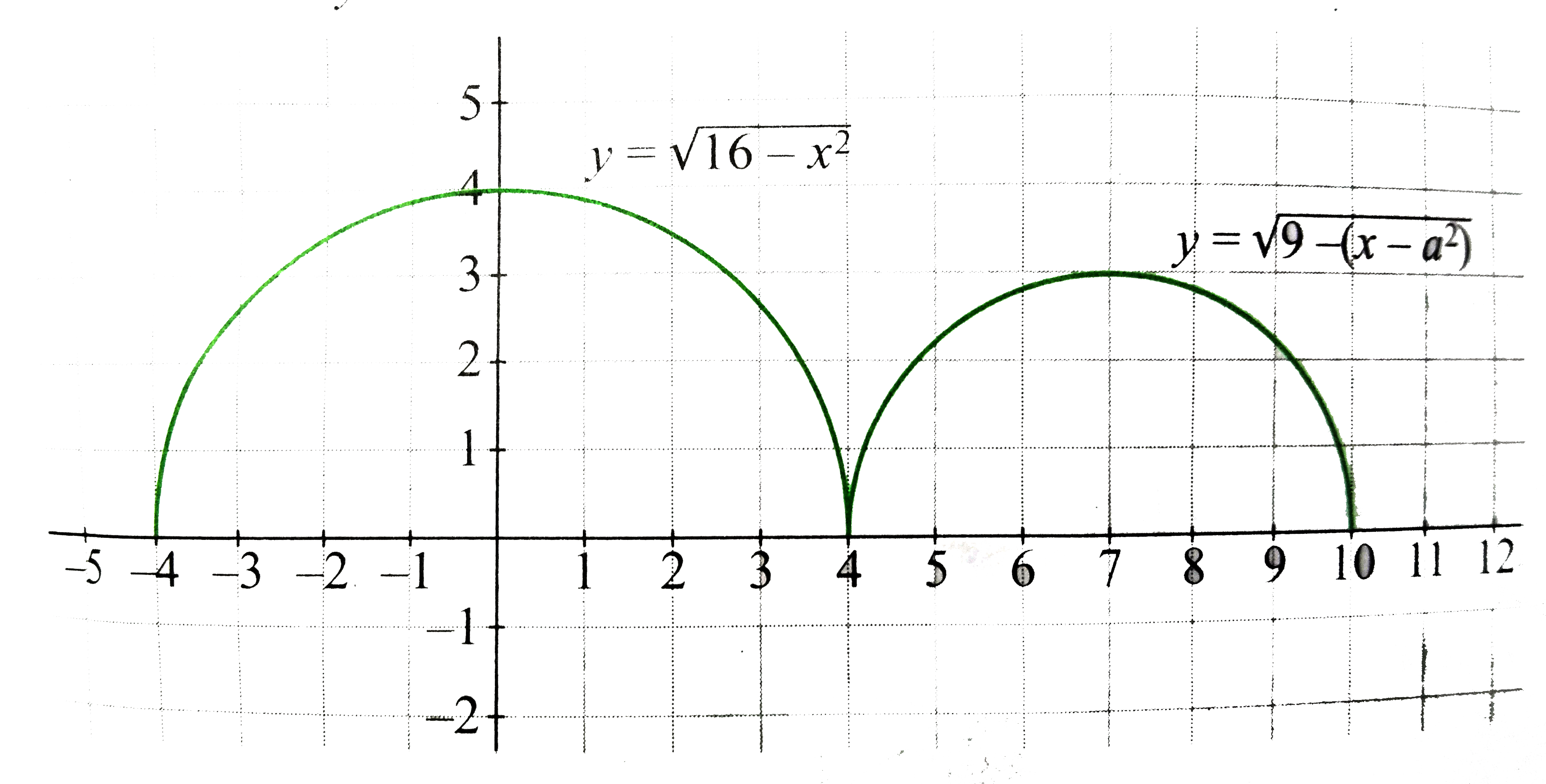
If a>7 , no solution exist, So, a≤7
Hence, maximum value of a is 7.
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Multiple)
25 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Comprehension)
46 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.6
5 Videos
- BINOMIAL THEOREMBOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
16 Videos
Similar Questions
about to only mathematics
View SolutionEach side of a square is of length 6 units and the centre of the square Is ( - 1, 2). One of its diagonals is parallel to x+y=0. Find the co-ordinates of the vertices of the square.
View SolutionIf the circle x2+y2+2x+3y+1=0 cuts x2+y2+4x+3y+2=0 at AandB , then find the equation of the circle on AB as diameter.
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View Solution(3+sqrt(8))^(x^(2)-2x+1)+(3-sqrt(8))^(x^(2)-2x-1)=((2)/(3-sqrt(8))) then the difference between the greatest value and the least possible value of x is:
View SolutionIf 1+2x+3x2+4x3+∞≥4 , then least value of ξs1/2 greatest value of ξs43 least value of ξs2/3 greatest value of x does not exists
View SolutionThe greatest integral value of a such that sqrt(9-a^(2)+2x-x^(2))>=sqrt(16-x^(2)) for at least one positive value of x is (a) 3 (b) 4 (c) 6 (d) 7
View SolutionNumber of possible integral values of sqrt(x-2)+sqrt(6-x) is
View SolutionThe value of the integral ∫320[x2]dx, where [] denotes the greatest integer function, is (A) 2+√2 (B) 2−√2 (C) 4+2√2 (D) 4−2√2
View SolutionThe greatest, the least values of the function ,f(x)=2−√1+2x+x2,x∈[−2,1] are respectively
View SolutionThe greatest and the least value of the function, f(x)=√1−2x+x2−√1+2x+x2,x∈(−∞,∞) are
View SolutionThe value of the definite integral ∫20(√1+x3+3√x2+2x) dx is :
View SolutionThe least integral value of a such that √9−a2+2ax−x2>√16−x2 for at least one positive x
View Solutionयदि limx→∞[√x4+ax3+3x2+bx+2−√x4+2x3−cx2+3x−d]=4 हो, तो a, b, c तथा d के मान है
View SolutionDo for the value of x: sqrt(2x^2+x+3)+sqrt(2x^2+x-6)=3
View Solution
