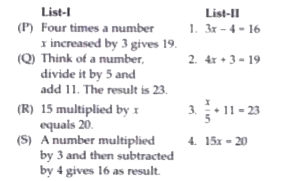Match the equation in List I with the number of solutions in List II.

A
abcdqpsr
B
abcdqprs
C
abcdsrqp
D
abcdpqrs
Text Solution
The correct Answer is:A
a. cosax+cosbx=2,(a≠0,b≠0)
⇒ax=2nπandbx=2mπ,m,n∈Z
⇒ab=nm (here ab must be rational)
or LCM of a and b must exist. Also, x=0 is one solution So, equation has at least one solution.
b. cosx+cos(√2)x=2
⇒x=2nπand√2x=2mπ,m,n∈Z
⇒√2n=m, which is satisfied by m=n=0 only.
So, equation has only one solution x=0.
LCM of (1,√2) does not exist or 1√2 is irrational number.
c. cosax+cosbx=1,(a≠0,b≠0)
Clearly, equation has infinite solutions.
d. cosx+cosπx=−2
⇒cosx=−1andcosπx=−1
⇒x=(2k+1)πandπx=(2m+1)π,m,n∈Z
⇒(2k+1)π=(2m+1), which does not hold.
So, equation has no solution.
|
Topper's Solved these Questions
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGECHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Exercise (Numerical)
21 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGECHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - JEE Main Previous Year
2 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGECHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Exercise (Comprehension)
20 Videos
- TRIGNOMETRIC RATIOS IDENTITIES AND TRIGNOMETRIC EQUATIONSBOOK - CENGAGECHAPTER - TRIGNOMETRIC RATIOS IDENTITIES AND TRIGNOMETRIC EQUATIONSEXERCISE - Question Bank
4 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - SINGLE CORRECT ANSWER TYPE
38 Videos
Similar Questions
Knowledge Check
Match the salls in List-I with their use in List-II.
 AP- 2, Q-2,3 R-2,4 S-1,3BP-1,2 Q-2,3 R-1,4 S-1,3CP-1,4 Q-1,3 R-2,3 S-2,4DP-2, Q-1,3, R-1, S-4
AP- 2, Q-2,3 R-2,4 S-1,3BP-1,2 Q-2,3 R-1,4 S-1,3CP-1,4 Q-1,3 R-2,3 S-2,4DP-2, Q-1,3, R-1, S-4Match the List-I and List-II:
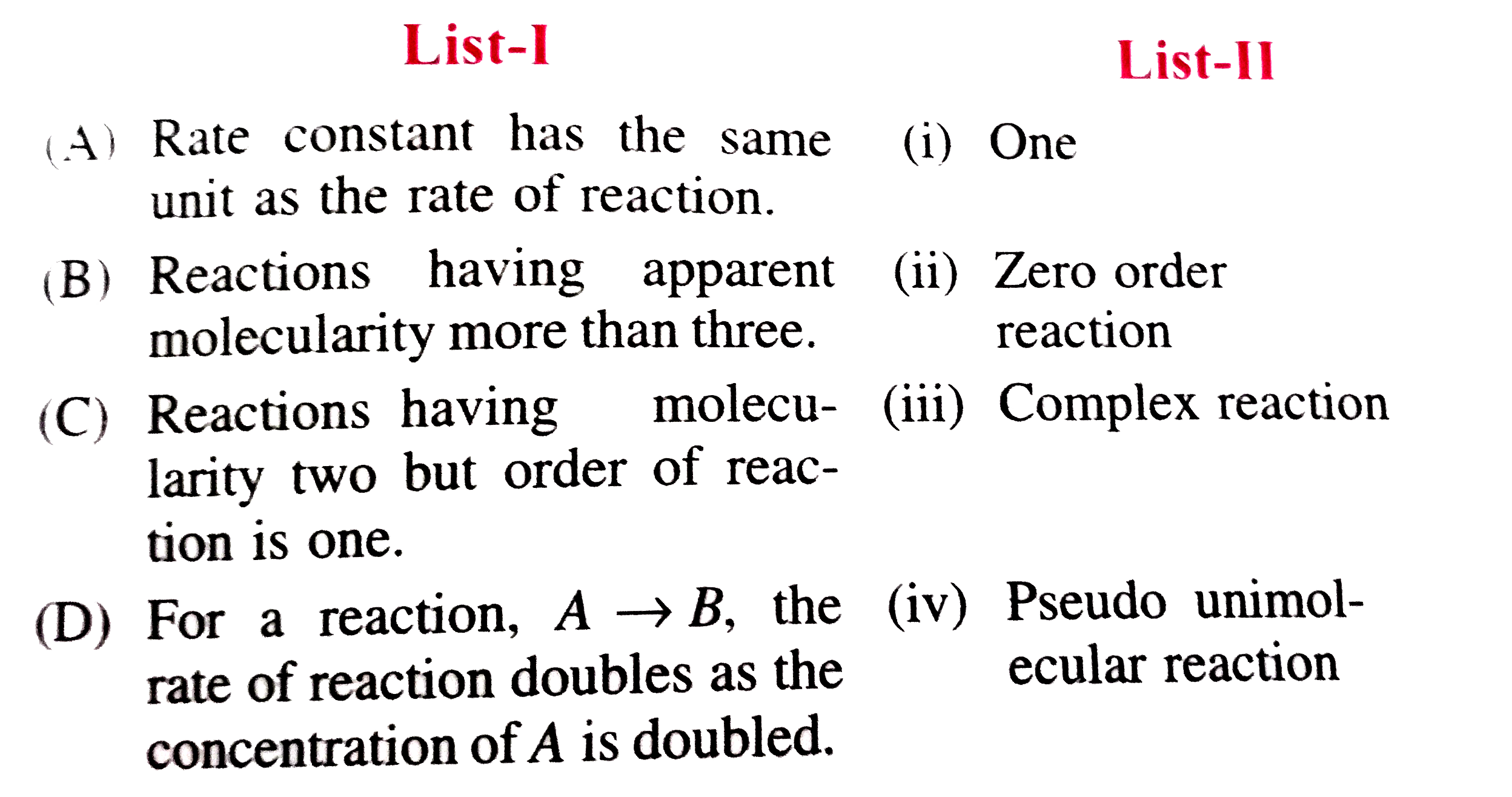 A(A)(B)(C)(D)iiiviiiiB(A)(B)(C)(D)iiiiiiviC(A)(B)(C)(D)iiiiiiviD(A)(B)(C)(D)iiiviiii
A(A)(B)(C)(D)iiiviiiiB(A)(B)(C)(D)iiiiiiviC(A)(B)(C)(D)iiiiiiviD(A)(B)(C)(D)iiiviiiiMatch the List-I and List-II:
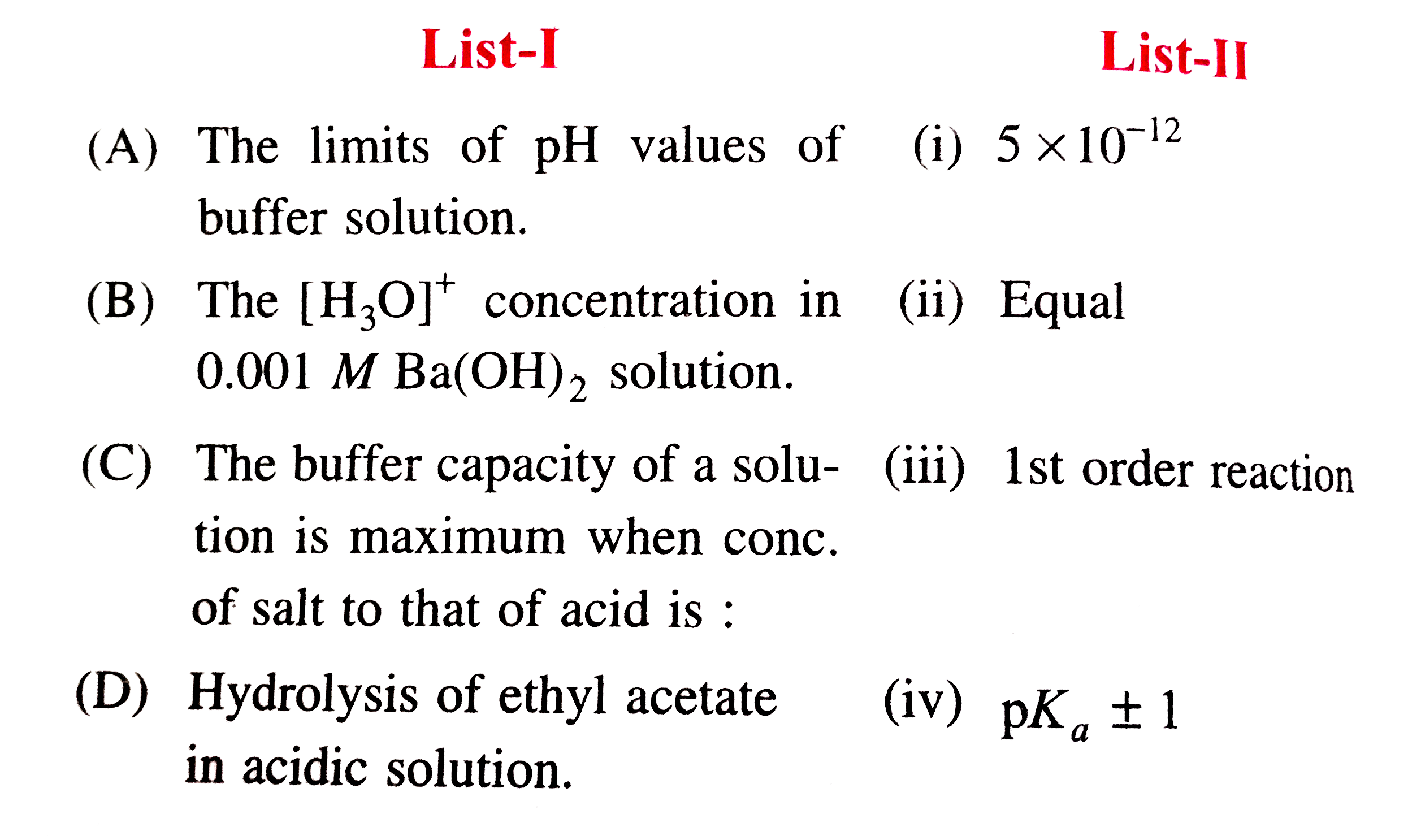 A(A)(B)(C)(D)iviiiiiiB(A)(B)(C)(D)iviiiiiiC(A)(B)(C)(D)iiviiiiiD(A)(B)(C)(D)iviiiiii
A(A)(B)(C)(D)iviiiiiiB(A)(B)(C)(D)iviiiiiiC(A)(B)(C)(D)iiviiiiiD(A)(B)(C)(D)iviiiiii