Doubtnut Promotions Sticky

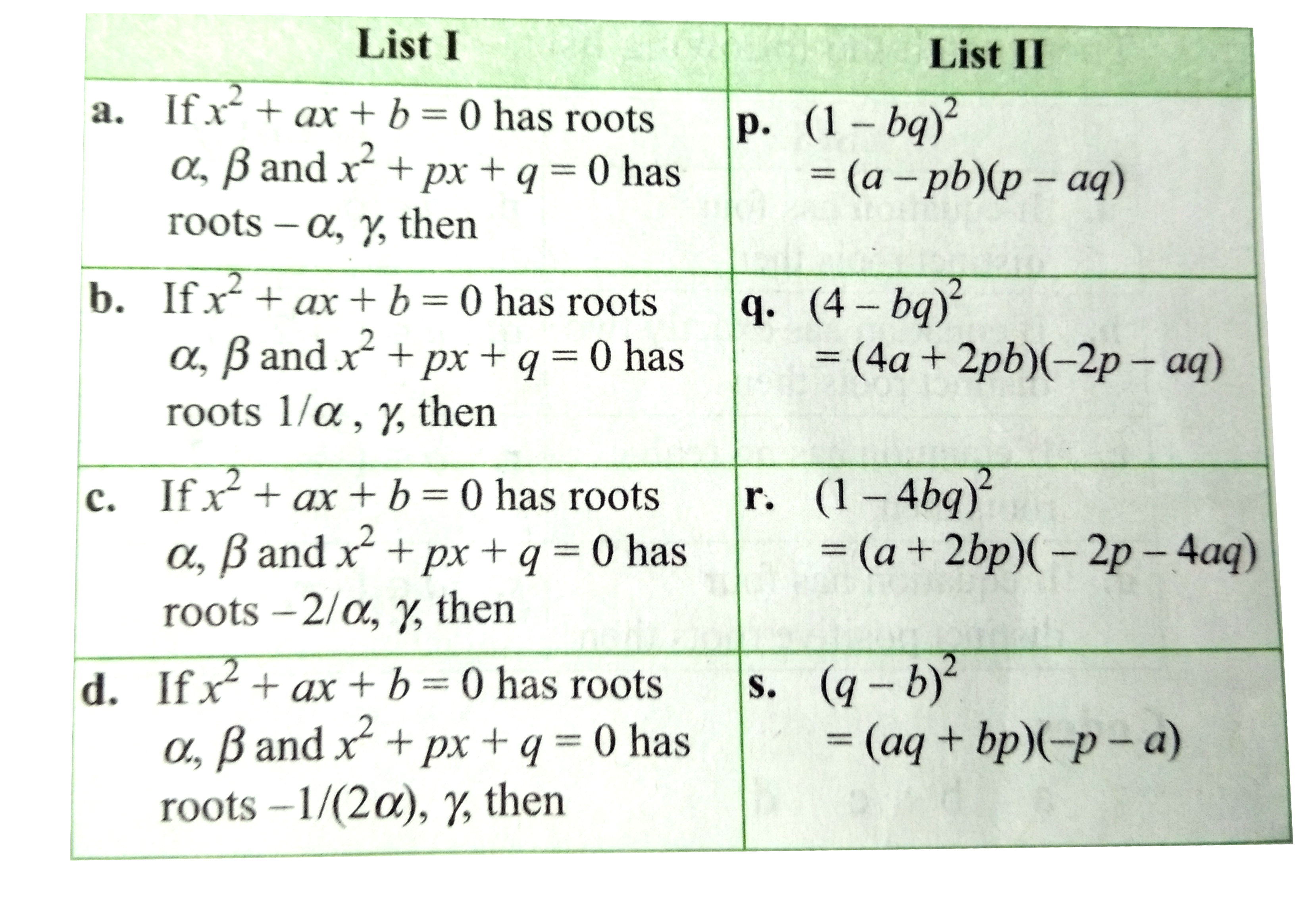
Match the following lists:
Match the following lists:

Text Solution
Verified by Experts

The correct Answer is:a→s;b→p;c→q;d→r

a→s,b→p,c→q,d→r
(a) x2ax+b=0 has root lapha. Hence.
α2+aα+b=0 (1)
x2+px+q=0 has root −α. Hence,
α2−pα+q= (2)
Eliminating α from (1) and (2), we get
(q−b)2=(aq+bp)(−p−a)
or (q−b)2=−(aq+pb)(p+a)
(b) x2ax+b=0 has root α. Hence,
α2+aα+b=0 (1)
x2+px+q=0 has root 1/α. Hence,
qα2+pα+1=0 (2)
Eliminating α from (1) and (2), we get
(1−bq)2=(a−pb)(p−aq)
(c) x2+ax+b=0 has roots, α,β. Hence,
α2+aα+b=0 (1)
x2+px+q=0 has roots −2/α,γ. Hence,
qα2−2pα+4=0 (2)
Eliminating α from (1) and (2), we et
(4−bq)2=(4a+2pb)(−2p−aq)
(d) x2+ax+b=0 has roots α,β. Hence,
α2+aα+b=0 (1)
x2+px+q=0 has roots −1/2α,γ. Hence,
4qα2−2pα+1=0 (2)
Elimintaing α from (1) and (2), we get
(1−4bq)2=(a+2bp)(−2p−4aq)

Doubtnut Promotions Banner
|