if tangents are drawn to the ellipse $x^{2} + 2 y^{2} = 2$ all points on the ellipse other its four vertices then the mid-points of the tangents intercepted between the coorinate axs lie on the curve Solution in Tamil

A

$\frac{x^{2}}{4} + \frac{y^{2}}{2} = 1$

B

$\frac{x^{2}}{4 x^{2}} + \frac{y^{2}}{2 y^{2}} = 1$

C

$\frac{x^{2}}{2} + \frac{y^{2}}{4} = 1$

D

$\frac{x^{2}}{2 x^{2}} + \frac{y^{2}}{4 y^{2}} = 1$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Given equation of ellipse is $x^{2} + 2 y^{2} = 2$ which can be written as $\frac{x^{2}}{2} + \frac{y^{2}}{1} = 1$
Let P be point on the ellipse , other than its four vertices , then the parametric coordinates of P be $(\sqrt{2} cos θ, sin θ)$

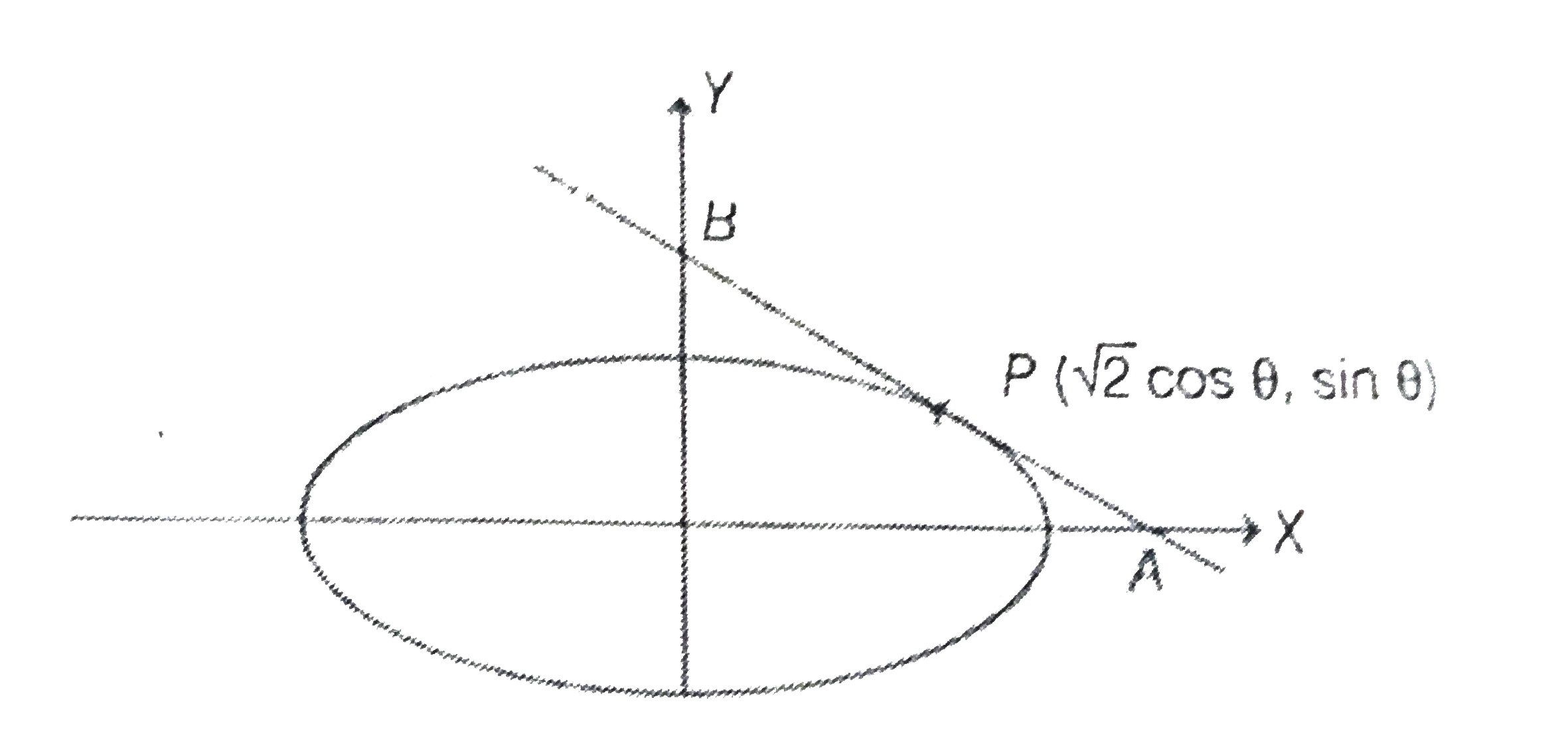
Now , the equation of tangent at P is
$\frac{x \sqrt{2} cos θ}{2} + \frac{y sin θ}{1} = 1$
$[∵$ equation of tangent at $(x_{1}, y_{1}_)$ is given by T=0 $\Rightarrow \frac{x x_{1}}{a^{2}} + \frac{y y_{1}}{b^{2}} = 1$ ]
$\Rightarrow \frac{x}{\sqrt{2} sec θ} + \frac{y}{cos e c θ} = 1$
$∴ A (\sqrt{2} s e x θ, 0) and B (0, cos sec θ)$
$L e t mid-point of A B b e R (h, k),$ then
$h = \frac{\sqrt{2} sec θ}{2} and k = \frac{cos e c θ}{2}$
$2 h = \sqrt{2} sec θ and 2 K = cos e c θ$
$\Rightarrow cos θ = \frac{1}{\sqrt{2 h}} and sin θ$
we know that , ${cos}^{2} θ + {sin}^{2} {sin}^{2} θ = 1$
$∴ \frac{1}{2 h^{2}} + \frac{1}{4 k^{2}} 1$
= so locus of $(h . k)$ is $\frac{1}{2 x^{2}} + \frac{1}{4 y^{2}} = 1$

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile