Two consecutive irreversible first order reactions can be represented by
Ak1−−→Bk2−−→C
The rate equation for A is readily interated to obtain
[A]t=[A]0.e−k1t , and [B]t=k1[A]0k2−k1[e−k1(t)−e−k2(t)]
When k1=1s−1 and k2=500s−1 , select most appropriate graph
a) =[A](0).e^(-k(1^(t))) , and [B](t)=(k(1)[A](0))/(k(2)-k(1))[e^(-k(1)(t))-e^(-k(2)(t))] When k(1)=1s^(-1) and k(2)=500s^(-1) , select most appropriate graph
a)
b)
c)
d)](https://d10lpgp6xz60nq.cloudfront.net/physics_images/NRA_PHY_CHM_JMA_C07_E01_187_O01.png) b)
b) 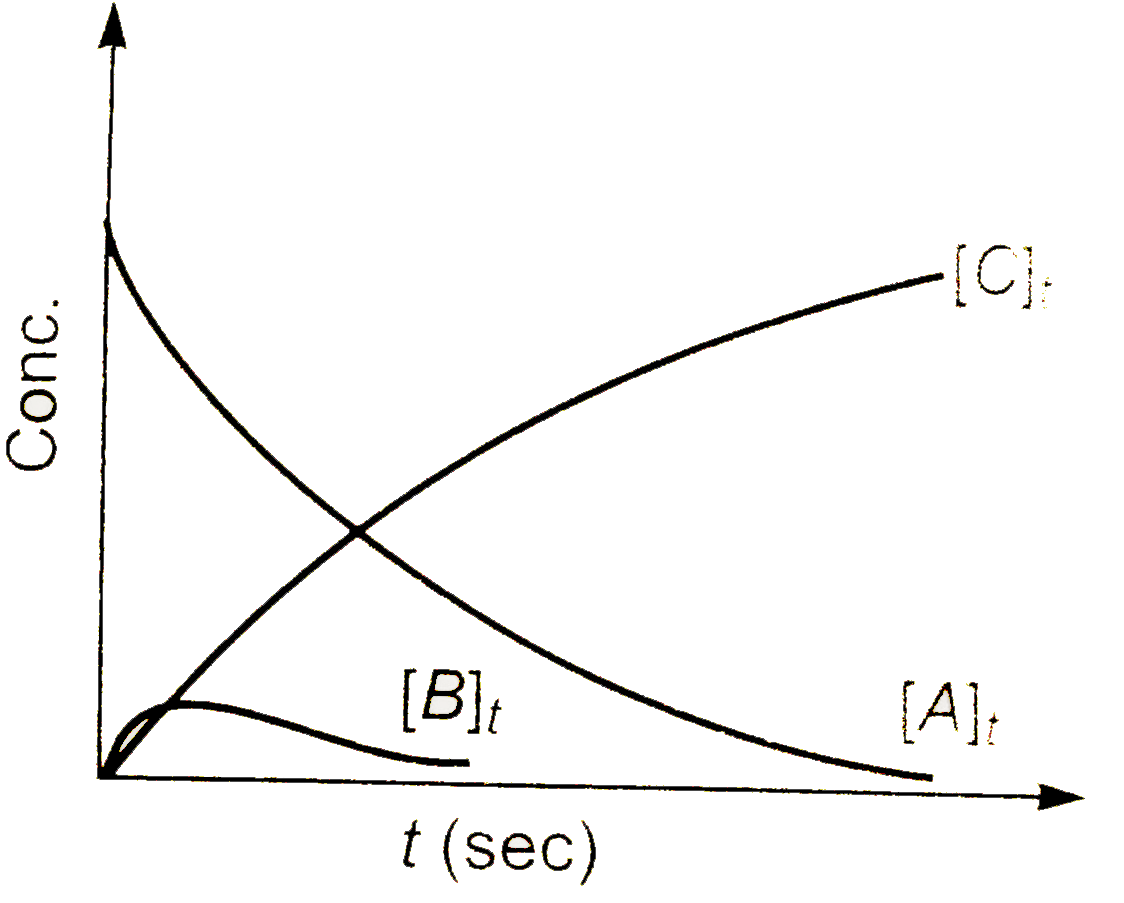
c) 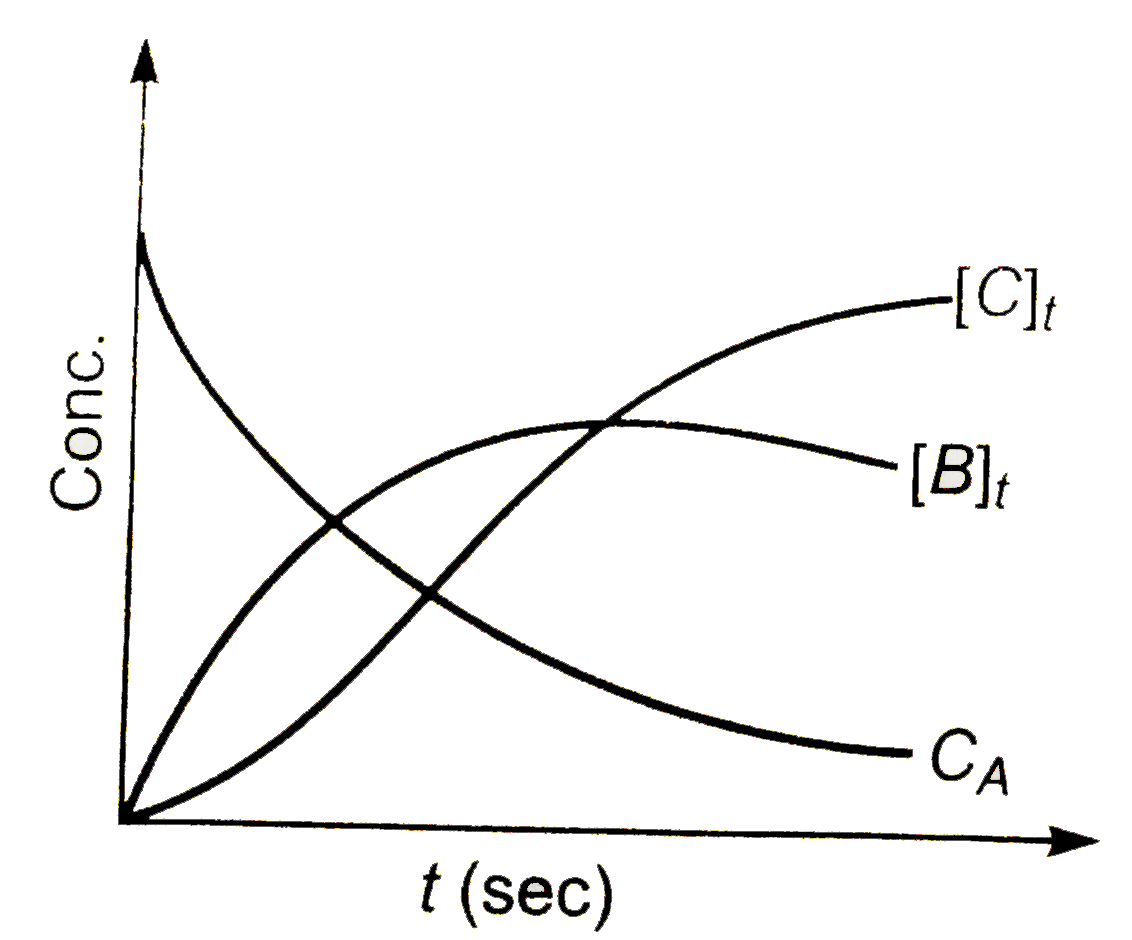 d)
d) 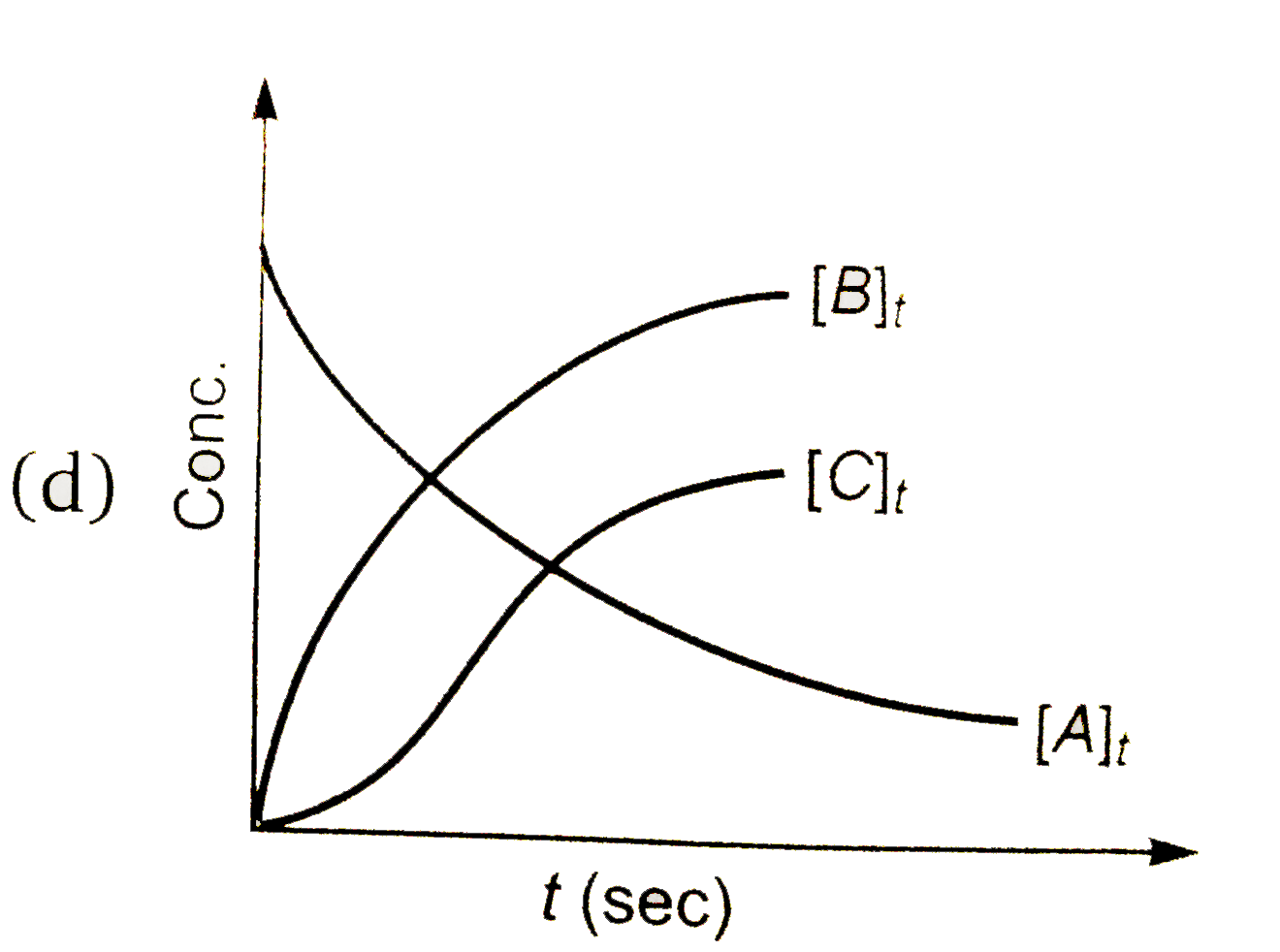 Solution in Punjabi
Solution in Punjabi
graph A
graph B
graph C
graph D
The correct Answer is:A
Step by step video & image solution for Two consecutive irreversible first order reactions can be represented by Aoverset(k_(1))(rarr)Boverset(k_(2))(rarr)C The rate equation for A is readily interated to obtain [A]_(t)=[A]_(0).e^(-k_(1^(t))) , and [B]_(t)=(k_(1)[A]_(0))/(k_(2)-k_(1))[e^(-k_(1)(t))-e^(-k_(2)(t))] When k_(1)=1s^(-1) and k_(2)=500s^(-1) , select most appropriate graph a) b) c) d) by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CHEMICAL KINETIC & NUCLEAR CHEMISTRYBOOK - NARENDRA AWASTHICHAPTER - CHEMICAL KINETIC & NUCLEAR CHEMISTRYEXERCISE - Level 3 - One Or More Answers Are Correct
11 Videos
- CHEMICAL KINETIC & NUCLEAR CHEMISTRYBOOK - NARENDRA AWASTHICHAPTER - CHEMICAL KINETIC & NUCLEAR CHEMISTRYEXERCISE - Level 3 - Assertion - Reason Type Questions
3 Videos
- CHEMICAL KINETIC & NUCLEAR CHEMISTRYBOOK - NARENDRA AWASTHICHAPTER - CHEMICAL KINETIC & NUCLEAR CHEMISTRYEXERCISE - Level 2 (Q.33 To Q.35)
2 Videos
- SURFACE CHEMISTRYBOOK - NARENDRA AWASTHICHAPTER - SURFACE CHEMISTRYEXERCISE - Level -2
1 Videos
Similar Questions
Select the correct statement(s) :
a) When T→∞ or Ea→O then K=A
b) A positive catalyst can change ΔH of the reaction
c) A mixture catalyst may be thermodynamically unstable by kinetically stable
d) A negative catalyst increases the activation energy of the reaction
View SolutionEach question contains STATEMENTS-1 (Assertion) and STATEMENT -2 (Reason).
Examine the statements carefully and mark the correct answer according to the instructions given below:
STATEMENTS-1: Acid catalysed hydrolysis of esters is pseudo first order reaction.
STATEMETNT-2:Water is present in excess in given reaction.
a) If both the statements are TRUE and STATEMENT-2 is the correct explanation of STATEMENT-1
b) If both the statement are TRUE but STATEMENT-2 is NOT the correct explanation of STATEMENT-1
c) If STATEMENT-1 is TRUE and STATEMENT-2 is FALSE
d) If STATEMENT-1 is FALSE and STATEMENT-2 is TRUE
View SolutionIn a sample of wood, the reading of a counter is 32 dpm and in a fresh sample of tree it is 122dpm . Due to error counter gives the reading 2 dpm in absence of .14C . Half life of .14C is 5770 years .
The approximate age (in years) of wood sample is :
View Solutionfor the graph below, select correct order of temperture ?
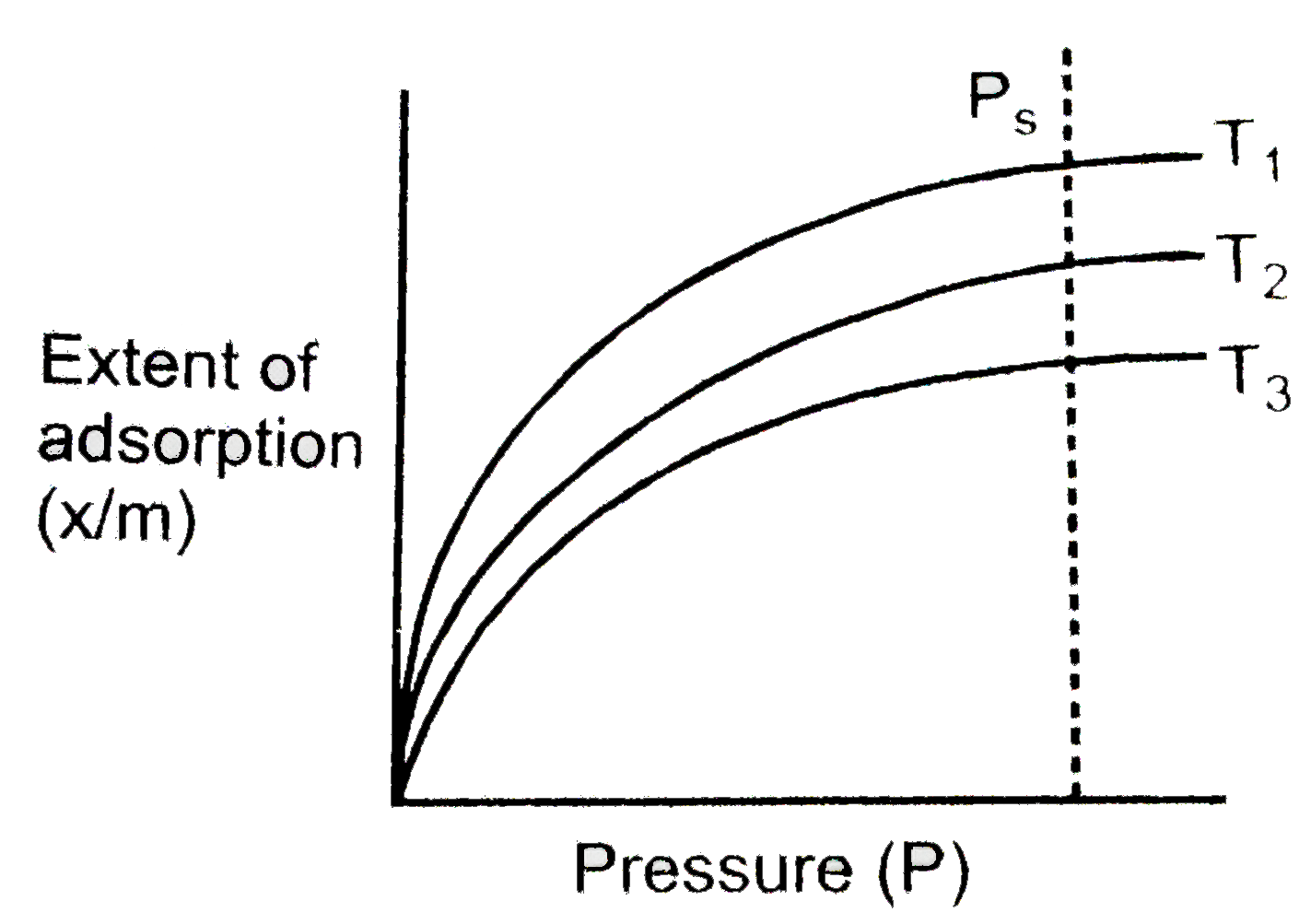
View SolutionFor a gaseous reaction, following data is given:
A→B,k1=1015e−2000/T
C→D,k2=1014e−1000/T
The temperature at which k1=k2 is
View SolutionFor the consecutive unimolecular-type first-order reaction Ak1−−→Rk2−−→S, the concentration of component R, CR at any time t is given by -
CR=COAK1[e−k1t(k2−k1)+e−k2t(k1−k2)]
if CA=CAO,CR=CRO=0 at t=0
The time at which the maximum concentration of R occurs is -
View SolutionThe rate constant k1 and k2 for two different reactions are 1016e−2000/T and 1015e−1000/T, respectively. The temperature at which k1=k2 is
View SolutionTwo consecutive irreversible fierst order reactions can be represented by Aoverset(k_(1))(rarr)Boverset(k_(2))(rarr)C The rate equation for A is readily interated to obtain [A]_(t)=[A]_(0).e^(-k_(1^(t))) , and [B]_(t)=(k_(1)[A]_(0))/(k_(2)-k_(1))[e^(-k_(1)^(t))-e^(-k_(2)^(t))] At what time will B be present in maximum concentration ?
View SolutionTwo consecutive irreversible fierst order reactions can be represented by
Ak1−−→Bk2−−→C
The rate equation for A is readily interated to obtain
[A]t=[A]0.e−k1t , and [B]t=k1[A]0k2−k1[e−kt1−e−kt2]
When k1=1s−1 and k2=500s−1 , select most appropriate graph
View SolutionTwo consecutive irreversible fierst order reactions can be represented by Aoverset(k_(1))(rarr)Boverset(k_(2))(rarr)C The rate equation for A is readily interated to obtain [A]_(t)=[A]_(0).e^(-k_(1^(t))) , and [B]_(t)=(k_(1)[A]_(0))/(k_(2)-k_(1))[e^(-k_(1)^(t))-e^(-k_(2)^(t))] If k_(1) and k_(2) both are almost same then which graph is most suitable ?
View SolutionTwo consecutive irreversible fierst order reactions can be represented by
Ak1−−→Bk2−−→C
The rate equation for A is readily interated to obtain
[A]t=[A]0.e−k1t , and [B]t=k1[A]0k2−k1[e−kt1−e−kt2]
Select the corret statement for given reaction:
View SolutionThe rate constants k_(1) and k_(2) for two different reactions are 10^(16) * e^(-2000//T) and 10^(15) * e^(-1000//T) , respectively . The temperature at which k_(1) = k_(2) is
View SolutionThe rate constants k1 and k2 for two different reactions are 1025e−4000/T and 1025e−1000/T, respectively. The temperature at which k2=k2 is
View SolutionThe rate constant k1 and k2 for two different reactions are 1016e−2000/T and 1015e−1000/T, respectively. The temperature at which k1=k2 is
View Solution{:(A rarr B, k_(A)=10^(15)e^(-2000//T),),(C rarr D, k_(C )=10^(14)e^(-1000//T)):} Temperature T K at which (k_(A)=k_(C )) is :
View SolutionFor the two gaseous reactions, following data are given A→B,k1=1010e−20,000/T,C→D,k2=1012e−24,606/T the temperature at which k1 becomes equal to k2 is :
View Solution
