A capacitor of 10 μF is connected to a source of 50 V and then disconnected. When the space between the plates is filled with teflon of dielectric constant 2.5. The new potential difference is -
20 V
20.8 V
23.8 V
25 V
The correct Answer is:A
To solve the problem step by step, we will follow the principles of capacitors and dielectrics.
Step 1: Understand the initial conditions
We have a capacitor with a capacitance C=10μF connected to a voltage source of V=50V. When connected, the charge Q on the capacitor can be calculated using the formula:
Q=C⋅V
Step 2: Calculate the initial charge
Substituting the values into the formula, we get:
Q=10×10−6F⋅50V=5×10−4C
So, the initial charge Q on the capacitor is 5×10−4C.
Step 3: Disconnect the battery
After disconnecting the battery, the charge Q on the capacitor remains constant because there is no external circuit to change it.
Step 4: Introduce the dielectric
Now, we fill the space between the capacitor plates with a dielectric material (Teflon) that has a dielectric constant K=2.5. The new capacitance C′ of the capacitor with the dielectric is given by:
C′=K⋅C=2.5⋅10μF=25μF
Step 5: Relate charge, capacitance, and voltage
The relationship between charge, capacitance, and voltage is given by:
Q=C′⋅V′
Since Q remains constant, we can set up the equation:
5×10−4C=25×10−6F⋅V′
Step 6: Solve for the new voltage V′
Rearranging the equation to solve for V′:
V′=QC′=5×10−4C25×10−6F
Calculating V′:
V′=5×10−425×10−6=525×102=0.2×100=20V
Conclusion
The new potential difference V′ after filling the capacitor with Teflon is 20V.
---
We have a capacitor with a capacitance C=10μF connected to a voltage source of V=50V. When connected, the charge Q on the capacitor can be calculated using the formula:
Q=C⋅V
Substituting the values into the formula, we get:
Q=10×10−6F⋅50V=5×10−4C
So, the initial charge Q on the capacitor is 5×10−4C.
After disconnecting the battery, the charge Q on the capacitor remains constant because there is no external circuit to change it.
Now, we fill the space between the capacitor plates with a dielectric material (Teflon) that has a dielectric constant K=2.5. The new capacitance C′ of the capacitor with the dielectric is given by:
C′=K⋅C=2.5⋅10μF=25μF
The relationship between charge, capacitance, and voltage is given by:
Q=C′⋅V′
Since Q remains constant, we can set up the equation:
5×10−4C=25×10−6F⋅V′
Rearranging the equation to solve for V′:
V′=QC′=5×10−4C25×10−6F
Calculating V′:
V′=5×10−425×10−6=525×102=0.2×100=20V
The new potential difference V′ after filling the capacitor with Teflon is 20V.
Similar Questions
Two parallel plate capacitors of capacitances C and 2C are connected in parallel and charged to a potential difference V. The battery is then disconnected and the region between the plates of the capacitor C is completely filled with a material of dielectric constant K. The potential differences across the capacitors now becomes...........
View SolutionA parallel plate capacitor is charged completely and then disconnected from the battery. IF the separation between the plates is reduced by 50% and the space between the plates if filled with a dielectric slab of dielectric constant 10, then the potential difference between the plates
View Solution
Knowledge Check
A capacitor with air as the dielectric is charged to a potential of 100 volts. If the space between the plates is now filled with a dielectric of dielectric constant 10, the potential difference between the plates will be
A1000 voltsB100 voltsC10 voltsDZeroAn air capacitor is charged to a potential of 150 V and then the charging battery is disconnected. If the space between the plates is then completely filled with a material of dielectric constant 10 , then the potential between the plates will become
A5 VB10 VC15 VD20 VThe capacitance of a parallel plate air condenser is 10μF. When the space between the plates is filled with a liquid of dielectric constant K, the potential difference between the plates reduces to 1/5 of the original value. The value of the dielectric constant of the liquid is
A2B5C10D8
Two parallel plate capacitos of capacitences C and 2C are connected in parallel and charged to a potential difference V0 . The battery is then disconnected and the region between the plates of the capacitor C completely filled with a material of dielectric constant 2. The potential difference across the capacitors now becomes-
View SolutionConsider a parallel plate capacitor of 10μF with air filled in the gap between the plates. Now one half of the space between the plates is filled with a dielectric of dielectric constant 4, as shown in the figure. The capacity of the capacitor charges to
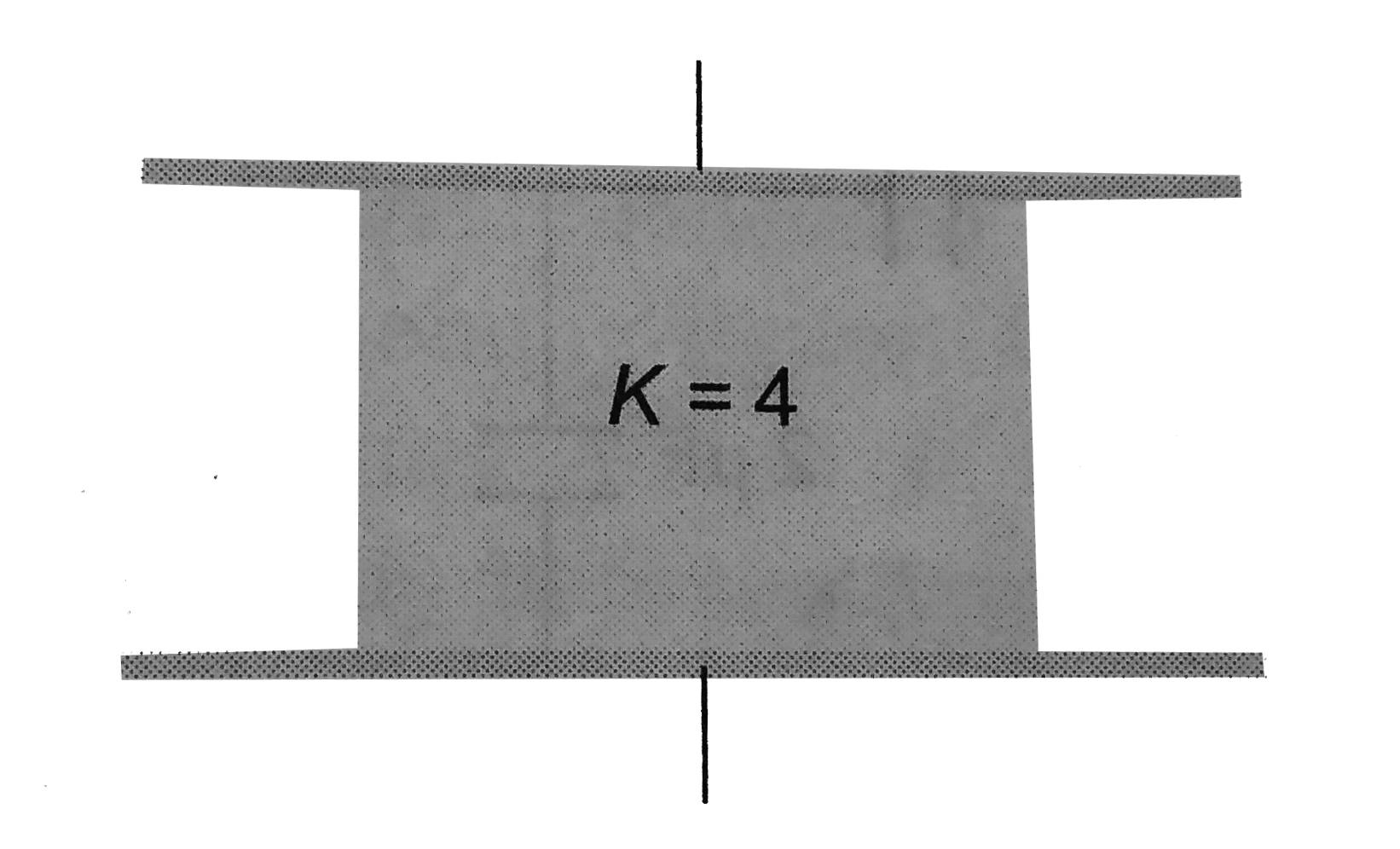
View SolutionA parallel plate capacitor of capacitance 10μF is connected across a battery of emf 5 mV. Now, the space between the plates of the capacitors is filled with a deielectric material of dielectric constant K=5. Then, the charge that will flow through the battery till equilibrium is reached is
View SolutionThe area of the plates of a capacitor is 50cm2 each and the separation between them is 1mm. The space between the plates is filled with a material of dielectric constant 4. the capacitor, initially uncharged, is connected to a battery of EMF 50 V. the work done by the battery until the capacitor gets completely charged is (in μJ).
View SolutionSTATEMENT-1: A parallel plate capacitor is charged by a battery of voltage V. The battery is then disconnected. If the space between the plates is filled with a dielectric, the enegry stroed in the capacitor will decrease
STATEMENT-2: The capacitance of a capacitor increases due to the introduciton of a dielectric between the plates.
View Solution
