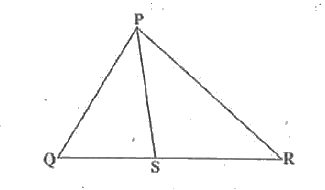
Prove that PR = 2 QS.'(##VPU_HSS_MAT_IX_C07_E01_008_Q01##)'Solution in Malayalam
Step by step video & image solution for Prove that PR = 2 QS.'(##VPU_HSS_MAT_IX_C07_E01_008_Q01##)' by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Find the distance between the two points on the number line, denoted by each pair of numbers given below:
i) 1,−5.
ii) 12,23
iii) −12,−13.
iy) −12,34
View SolutionThe weight of 6 players in a volley ball team are all different and the average weight is 60 kilograms
i) Prove that the team has at least one player weighing more than 60 kilograms.
ii) Prove that the team has at least one player weighing less than 60 kilograms.
View SolutionPQRS is a parallelogram in which PR is perpendicular to QS. If PR=8cm and QS=6 cm, then find PS
View Solutionआकृति में PS कोण QPR का समद्विभाजक है । सिद्ध कीजिए कि QSSR=PQPR है ।
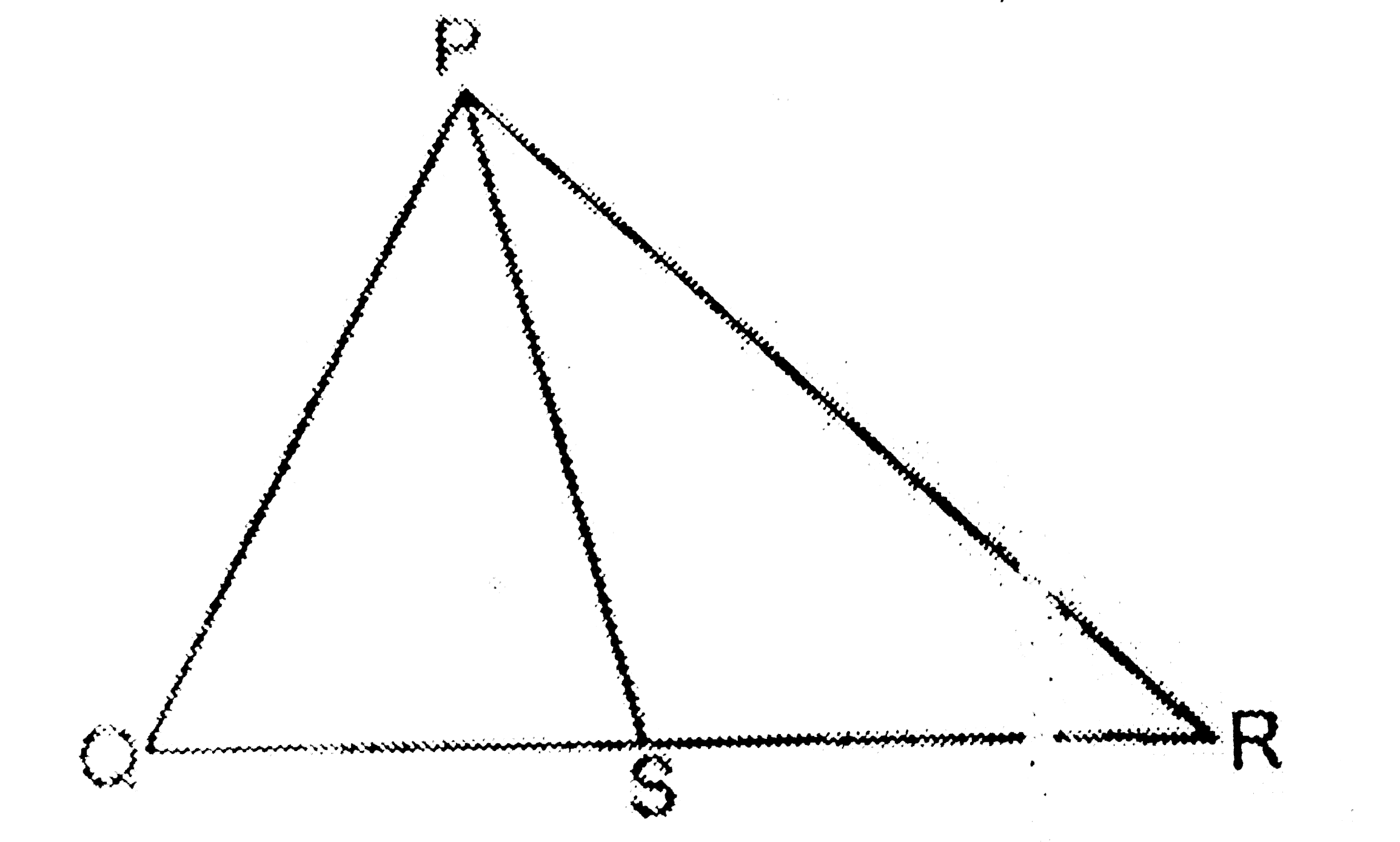
View Solutionबगल के चित्र में RS = QT और QS = RT. साबित करें कि PQ = PR.
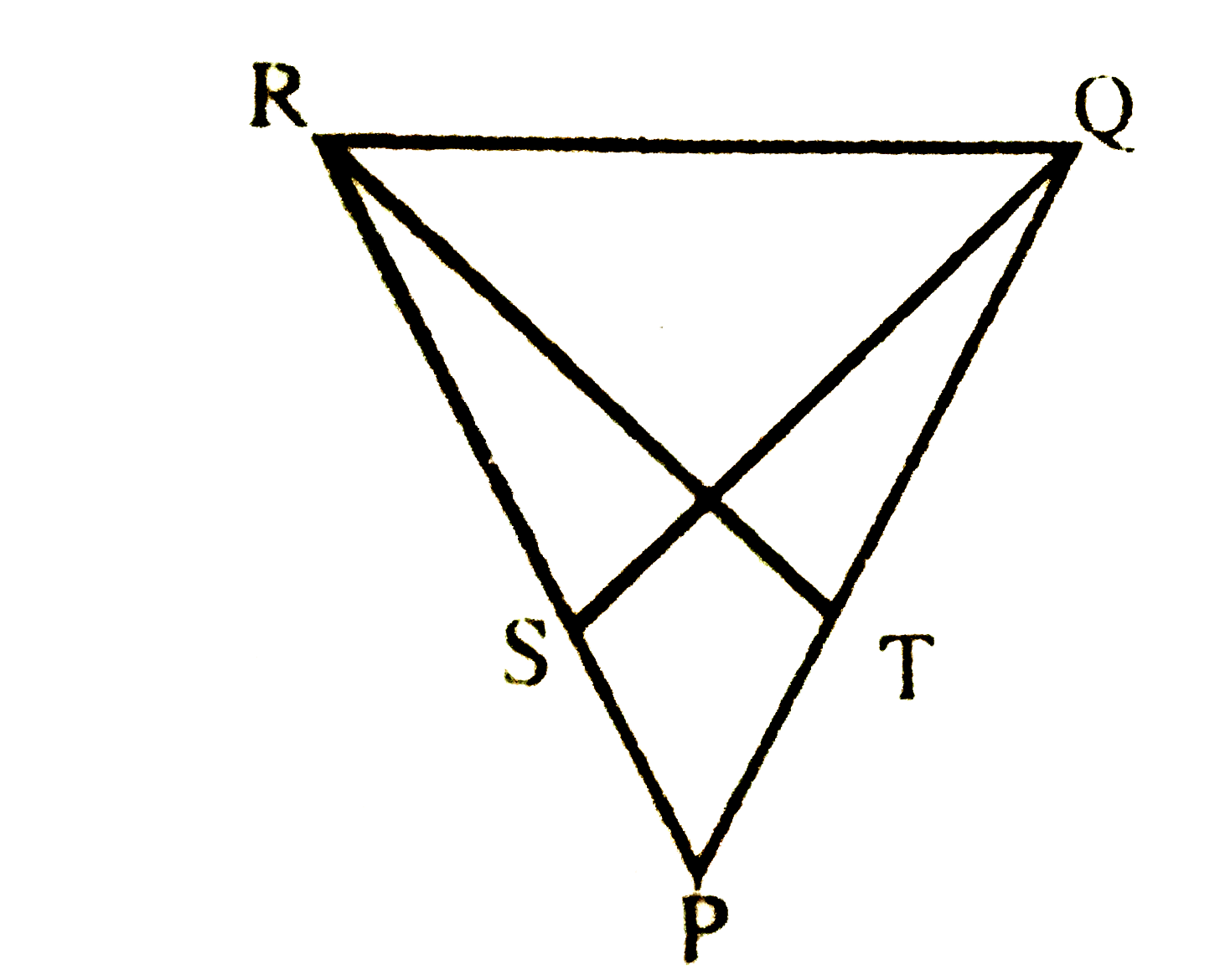
View Solutionबगल की आकृति में PQ = PR और PR पर S कोई
बिन्दु है साबित करें की RS<QS.
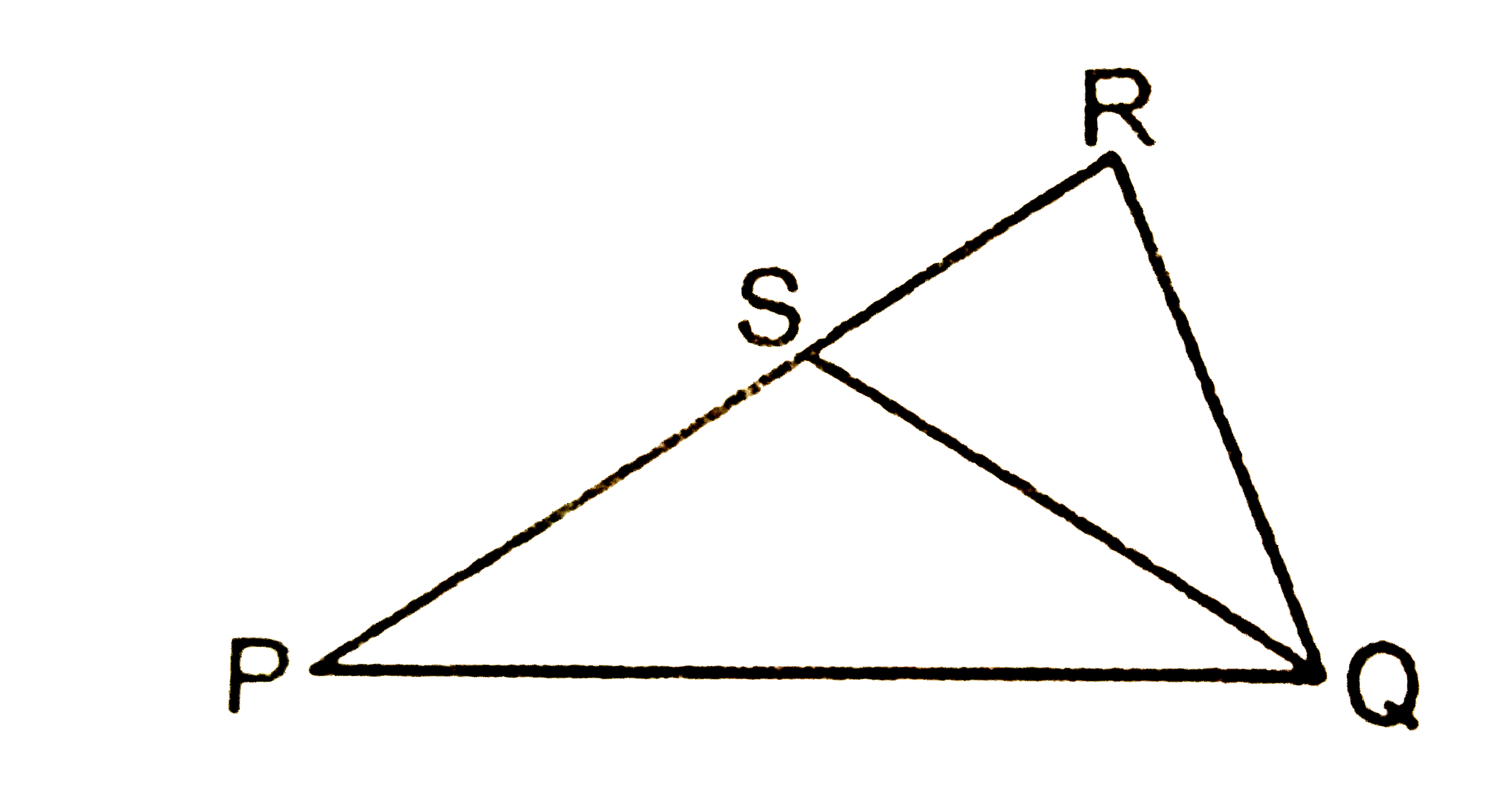
View Solutionचित्र में , PS = QR तथा ∠SPQ=∠RQP है तो सिद्ध कीजिए कि PR = QS तथा ∠QPR=∠PQS
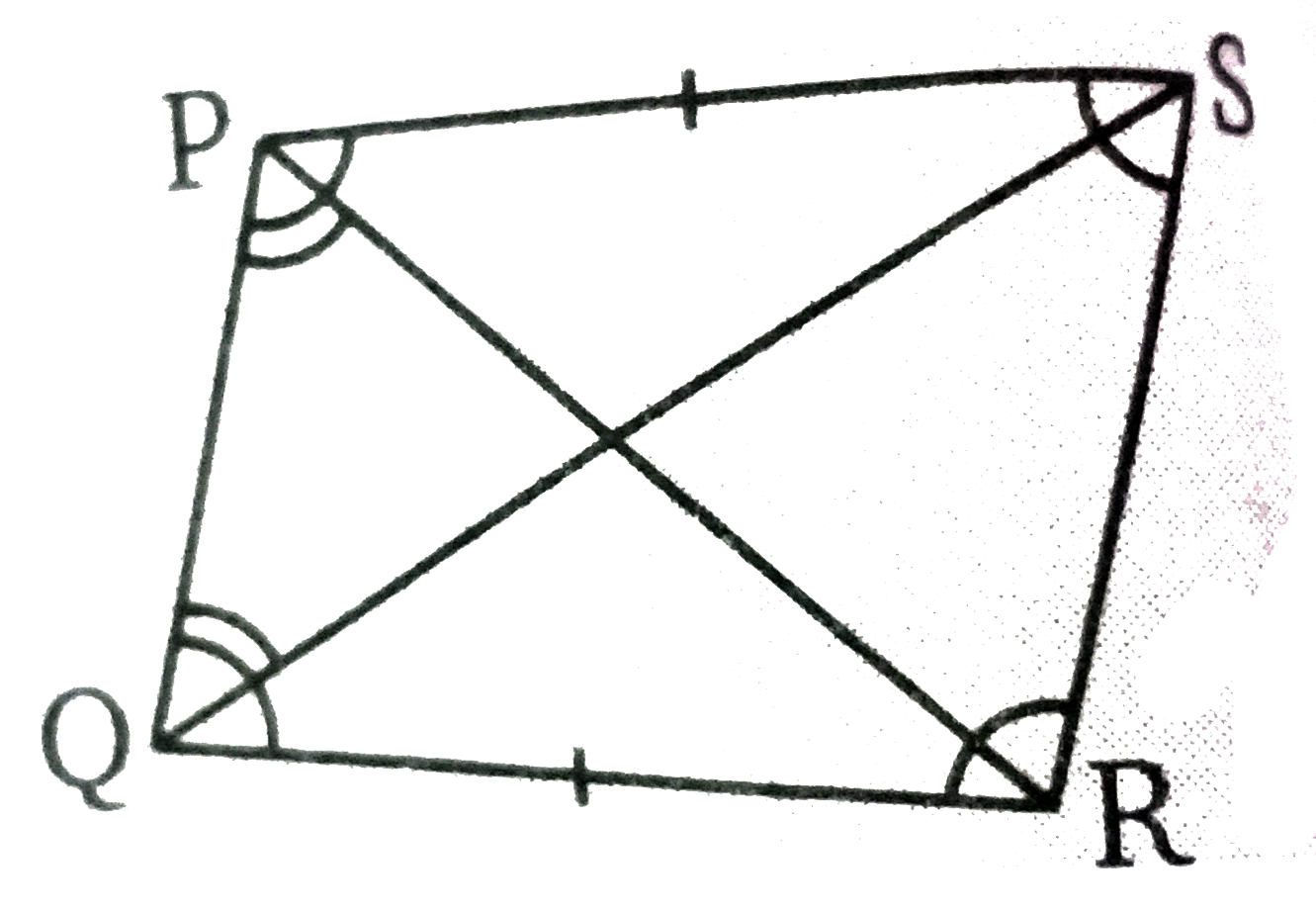
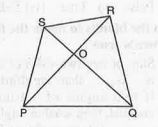
View SolutionPQRS एक चतुर्भुज है |जिसके विकर्ण PR तथा QS बिन्दु O पर प्रतिच्छेद करते हैं | सिध्द करो कि
(i) PQ+QR+RS+SP>PR+QS
(ii)PQ+QR+RS+SP<2(PR+QS)
View SolutionPQRS एक चतुर्भुज है जिसके विकर्ण PR और QS, बिंदु O पर प्रतिच्छेद करते है। सिद्ध करें कि -(१)-PQ+QR+RS+SP>PR+QS,(2)-PQ+QR+RS+SP<2(PR+QS)
View SolutionIn Fig PS is the bisector of ∠QPR of ΔPQR . Prove that QSSR=PQPR
View SolutionProve that, in a parallelogram PQRS,−−→PR−−−→QS=−−−→2PQ
View Solutionএকটি সামান্তরিক PQRS-এ প্রমাণ করো যে,−−→PR−−−→QS=2−−→PQ
View SolutionIn the given figure PR=QS Prove that PQ=RS Will happen
View SolutionDiagonals PR and QS of a quadrilateral PQRS intersect each other at O. Prove that PR+QR+RS+SP<2(PR+QS).
View Solutionआकृति में, PS=QR और ∠SPQ=∠RQP तो सिद्ध करें कि PR=QS और ∠QPR=∠PQS
View SolutionIn △PQR,m∠PQR=90∘, segQS⊥hypPR, then: a)PS2=QS×PR b)QS2=PS×RS c)PR2=QS×PS d)PR2=QS2×PS2
View Solution
