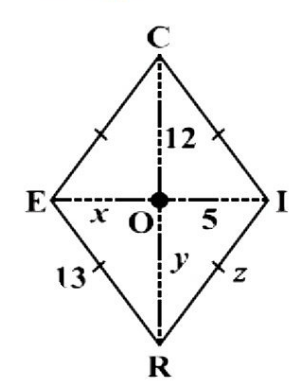
If RICE is a rhombus with EC = 20 cm and OC = 12 cm, can you find x, y, z ?
To solve the problem, we need to find the values of x, y, and z in the rhombus RICE, given that EC = 20 cm and OC = 12 cm.
1. Understanding the Rhombus Properties:
In a rhombus, the diagonals bisect each other at right angles. Let's denote the diagonals as EI and RC, where E and R are opposite vertices and I and C are the midpoints of the diagonals.
2. Given Information:
- EC (one diagonal) = 20 cm
- OC (half of diagonal EC) = 12 cm
3. Finding the Length of the Other Diagonal:
Since OC is half of EC, we can denote the full length of diagonal EI as 2y. Therefore, we have:
OC=y=12 cm
Hence, the full length of diagonal EI is:
EI=2y=2×12=24 cm
4. Using the Right Triangle Formed by the Diagonals:
In the right triangle formed by the diagonals, we can apply the Pythagorean theorem. The sides of the triangle are:
- Half of EC = x (which we need to find)
- Half of EI = y = 12 cm
- The side of the rhombus (which we denote as z)
According to the properties of the rhombus:
z2=x2+y2
5. Finding x:
We know that EC = 20 cm, so:
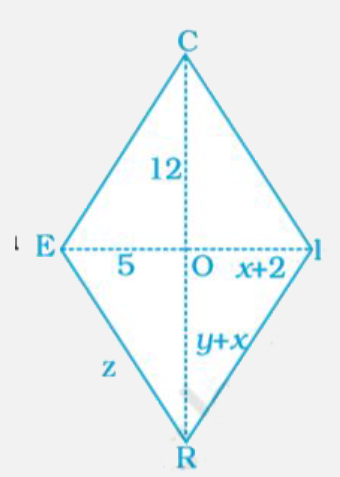
x+2=5⟹x=5−2=3 cm
6. Finding y:
We already established that y = 12 cm.
7. Finding z:
Since all sides of a rhombus are equal, we can say:
z=EC=20 cm
Summary of Values:
- x=3 cm
- y=12 cm
- z=20 cm
In a rhombus, the diagonals bisect each other at right angles. Let's denote the diagonals as EI and RC, where E and R are opposite vertices and I and C are the midpoints of the diagonals.
- EC (one diagonal) = 20 cm
- OC (half of diagonal EC) = 12 cm
Since OC is half of EC, we can denote the full length of diagonal EI as 2y. Therefore, we have:
OC=y=12 cm
Hence, the full length of diagonal EI is:
EI=2y=2×12=24 cm
In the right triangle formed by the diagonals, we can apply the Pythagorean theorem. The sides of the triangle are:
- Half of EC = x (which we need to find)
- Half of EI = y = 12 cm
- The side of the rhombus (which we denote as z)
z2=x2+y2
We know that EC = 20 cm, so:
x+2=5⟹x=5−2=3 cm
We already established that y = 12 cm.
Since all sides of a rhombus are equal, we can say:
z=EC=20 cm
- x=3 cm
- y=12 cm
- z=20 cm
Topper's Solved these Questions
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - APPLICATIONS, GAMES AND PUZZLES
3 Videos
- SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTBOOK - NCERT EXEMPLARCHAPTER - SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTEXERCISE - Exercise
52 Videos
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - EXERCISE
91 Videos
Similar Questions
Knowledge Check
In the figure AD=12cm,AB=20cmandAE=10cm. Find EC :
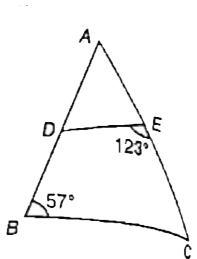 A14 cmB10 cmC8 cmD15 cm
A14 cmB10 cmC8 cmD15 cmIf diagonals of a rhombus are 12 cm and 16 cm, then what is the perimeter (in cm) of the rhombus?
A20B40C60D80
The diagonals of a rhombus are 8 cm and 15 cm. Find its side.
View Solution
RICE is a rhombus. Find x, y, z.
View SolutionIf the side of a rhombus is 10 cm and the longer diagonal is 16 cm, find the length of the other diagonal and hence the area of the rhombus.
View SolutionIf the diagonals of a rhombus are 12cm and 16cm, find the length of each side.
View SolutionThe diagonals of a rhombus are 7.5 cm and 12 cm. Find its area.
View SolutionThe area of a rhombus whose diagonals are 10 cm and 12 cm is x cm2. The value of x is
View Solution