If the diagonal d of a quadrilateral is doubled and the heights h1 and h2 falling on d are halved, then the area of quadrilateral is __________.
To solve the problem, we need to understand how the area of a quadrilateral changes when the diagonal is doubled and the heights are halved. Let's denote the diagonal of the quadrilateral as d and the heights from the opposite vertices to this diagonal as h1 and h2.
1. Understanding the Area of a Quadrilateral:
The area A of a quadrilateral can be calculated using the formula:
A=12×d×(h1+h2)
where d is the length of the diagonal and h1 and h2 are the heights from the opposite vertices to the diagonal.
2. Doubling the Diagonal:
If the diagonal d is doubled, then the new diagonal becomes:
d′=2d
3. Halving the Heights:
If the heights h1 and h2 are halved, then the new heights become:
h′1=h12andh′2=h22
4. Calculating the New Area:
Substituting the new values into the area formula:
A′=12×d′×(h′1+h′2)
Replacing d′, h′1, and h′2:
A′=12×(2d)×(h12+h22)
Simplifying the expression:
A′=12×2d×(h1+h22)
A′=d×(h1+h2)2
A′=12×d×(h1+h2)
5. Final Result:
We see that the new area A′ is equal to the original area A:
A′=A
Therefore, the area of the quadrilateral remains unchanged.
Conclusion:
The area of the quadrilateral is the same after doubling the diagonal and halving the heights.
The area A of a quadrilateral can be calculated using the formula:
A=12×d×(h1+h2)
where d is the length of the diagonal and h1 and h2 are the heights from the opposite vertices to the diagonal.
If the diagonal d is doubled, then the new diagonal becomes:
d′=2d
If the heights h1 and h2 are halved, then the new heights become:
h′1=h12andh′2=h22
Substituting the new values into the area formula:
A′=12×d′×(h′1+h′2)
Replacing d′, h′1, and h′2:
A′=12×(2d)×(h12+h22)
Simplifying the expression:
A′=12×2d×(h1+h22)
A′=d×(h1+h2)2
A′=12×d×(h1+h2)
We see that the new area A′ is equal to the original area A:
A′=A
Therefore, the area of the quadrilateral remains unchanged.
The area of the quadrilateral is the same after doubling the diagonal and halving the heights.
Topper's Solved these Questions
- MENSURATIONBOOK - NCERT EXEMPLARCHAPTER - MENSURATIONEXERCISE - APPLICATIONS, GAMES AND PUZZLES
13 Videos
- MENSURATIONBOOK - NCERT EXEMPLARCHAPTER - MENSURATIONEXERCISE - THINK AND DISCUSS
2 Videos
- MENSURATIONBOOK - NCERT EXEMPLARCHAPTER - MENSURATIONEXERCISE - THINK AND DISCUSS
2 Videos
- LINEAR EQUATIONS IN ONE VARIABLEBOOK - NCERT EXEMPLARCHAPTER - LINEAR EQUATIONS IN ONE VARIABLEEXERCISE - THINK AND DISCUSS
3 Videos
- PLAYING WITH NUMBERS BOOK - NCERT EXEMPLARCHAPTER - PLAYING WITH NUMBERS EXERCISE - Think and Discuss
1 Videos
Similar Questions
If both the diagonals of a quadrilateral divides it into four triangles of equal area, then the quadrilateral is a rhombus.
View SolutionIf each diagonals of a quadrilateral separates it into two triangles of equal area then show that the quadrilateral is a parallelogram.
View SolutionIn the given figure, compute the area of quadilateral
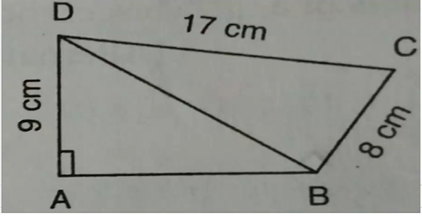
View SolutionIf the diagonals of a quadrilateral bisect each other at right angle, then the quadrilateral is a parallelogram (b) rectangle (c) rhombus (d) kite
View SolutionProve that If each diagonal of a quadrilateral separates it into two triangles of equal area then show that the quadrilateral is a parallelogram.
View SolutionIf →p and →q are two diagonals of a quadrilateral such that |→p−→q|=→p.→q,|→p|=1,|→q|=√2, then the area of quadrilateral is equal to
View SolutionIf the diagonals AC, BD of a quadrilateral ABCD, intersect at O, and separate the quadrilateral into four triangles of equal area, show that quadrilateral ABCD is a parallelogram.
View SolutionIf the diagonal BD of a quadrilateral ABCD bisects both ∠B and ∠D , show that ABBC=ADCD .
View SolutionThe position vectors of the vertices of a quadrilateral with A as origin are B(→b),D(→d)andC(l→b+m→d). Prove that the area of the quadrialateral is 12(l+m)∣∣→b×→d∣∣.
View SolutionRadius of a cylinder is r and the height is h. Find the change in the volume if the
height is doubled and the radius is halved.
View Solution
