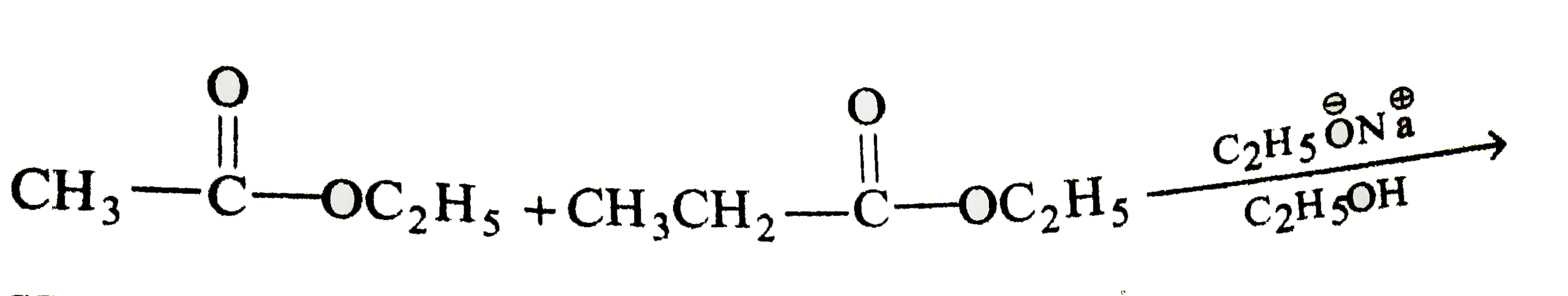Tell how many different angles would be formed by a transversal intersecting three parallel lines. How many different angle measures would there be?
To solve the problem of how many different angles are formed by a transversal intersecting three parallel lines and how many different angle measures there would be, we can follow these steps:
1. Identify the Lines and Transversal:
- We have three parallel lines, which we will label as L1, L2, and L3.
- A transversal line, which we will label as T, intersects these three parallel lines.
2. Count the Total Angles Formed:
- When a transversal intersects parallel lines, it creates angles at each intersection.
- Since there are three parallel lines and the transversal intersects each of them, a total of 12 angles are formed (4 angles at each intersection).
3. Identify Corresponding Angles:
- Corresponding angles are angles that are in the same position at each intersection.
- For example, angle 1 (formed at L1) is corresponding to angle 5 (formed at L2) and angle 9 (formed at L3). Therefore, angle 1 = angle 5 = angle 9.
4. Identify Vertically Opposite Angles:
- Vertically opposite angles are equal when two lines intersect.
- For instance, angle 1 is vertically opposite to angle 3, so angle 1 = angle 3.
5. Group the Angles:
- From the above relationships, we can group the angles:
- Group 1: Angle 1, Angle 5, Angle 9 (corresponding angles)
- Group 2: Angle 2, Angle 6, Angle 10 (corresponding angles)
- Angle 2 is also vertically opposite to angle 4, angle 6 is vertically opposite to angle 8, and angle 10 is vertically opposite to angle 12.
6. Determine Unique Angle Measures:
- From the groups, we can see that:
- All angles in Group 1 are equal (1 unique angle measure).
- All angles in Group 2 are equal (1 unique angle measure).
- Therefore, we have only two unique angle measures.
7. Conclusion:
- Total angles formed = 12
- Different angle measures = 2
Final Answer:
- The total number of different angles formed by a transversal intersecting three parallel lines is 12.
- The number of different angle measures is 2.
- We have three parallel lines, which we will label as L1, L2, and L3.
- A transversal line, which we will label as T, intersects these three parallel lines.
- When a transversal intersects parallel lines, it creates angles at each intersection.
- Since there are three parallel lines and the transversal intersects each of them, a total of 12 angles are formed (4 angles at each intersection).
- Corresponding angles are angles that are in the same position at each intersection.
- For example, angle 1 (formed at L1) is corresponding to angle 5 (formed at L2) and angle 9 (formed at L3). Therefore, angle 1 = angle 5 = angle 9.
- Vertically opposite angles are equal when two lines intersect.
- For instance, angle 1 is vertically opposite to angle 3, so angle 1 = angle 3.
- From the above relationships, we can group the angles:
- Group 1: Angle 1, Angle 5, Angle 9 (corresponding angles)
- Group 2: Angle 2, Angle 6, Angle 10 (corresponding angles)
- Angle 2 is also vertically opposite to angle 4, angle 6 is vertically opposite to angle 8, and angle 10 is vertically opposite to angle 12.
- From the groups, we can see that:
- All angles in Group 1 are equal (1 unique angle measure).
- All angles in Group 2 are equal (1 unique angle measure).
- Therefore, we have only two unique angle measures.
- Total angles formed = 12
- Different angle measures = 2
- The total number of different angles formed by a transversal intersecting three parallel lines is 12.
- The number of different angle measures is 2.
Topper's Solved these Questions
- LINES AND ANGLES BOOK - NCERT EXEMPLARCHAPTER - LINES AND ANGLES EXERCISE - Exercise
105 Videos
- LINES AND ANGLES BOOK - NCERT EXEMPLARCHAPTER - LINES AND ANGLES EXERCISE - Exercise (fill in the blanks)
3 Videos
- LINES AND ANGLES BOOK - NCERT EXEMPLARCHAPTER - LINES AND ANGLES EXERCISE - Solved Examples (fill in the blanks)
3 Videos
- INTEGERSBOOK - NCERT EXEMPLARCHAPTER - INTEGERSEXERCISE - VOCOBULARY
5 Videos
- PERIMETER AND AREABOOK - NCERT EXEMPLARCHAPTER - PERIMETER AND AREAEXERCISE - Think and Discuss
7 Videos
Similar Questions
Knowledge Check
How many different codons are possible?
A16B32C48D64If a transversal line cuts two parallel lines then bisector of internal angle formed a
ArectangleBsquareCrhombusDparallelogram
If a transversal intersects two parallel lines; then each of alternate interior angles are equAL.
View SolutionHow many pairs of adjacent angles are formed when two lines intersect at a point?
View SolutionHow many pairs of adjacent angles are formed when two lines intersect in a point?
View SolutionIf a transversal intersects two parallel lines, then
corresponding angles are on the ______ side of the transversal
View SolutionIf a transversal intersects two parallel lines, then
alternate interior angles are on the _______ side of the transversal.
View SolutionIf a transversal intersects two parallel lines, then
sum of interior angles on the same side of a transversal is _______
View Solution