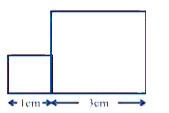
If p squares of each side 1mm makes a square of side 1cm, then p is
10
100
1000
10000
The correct Answer is:B
To solve the problem of finding the value of p when p squares of each side 1 mm make a square of side 1 cm, we can follow these steps:
Step 1: Understand the dimensions
We know that:
- The side of the larger square is 1 cm.
- The side of each smaller square is 1 mm.
Step 2: Convert units
Since we want to compare the areas, we need to convert the side of the larger square from centimeters to millimeters.
- 1 cm = 10 mm.
Step 3: Calculate the area of the larger square
The area A of the larger square can be calculated using the formula for the area of a square, which is:
A=side×side
So,
A=10 mm×10 mm=100 mm2
Step 4: Calculate the area of one smaller square
The area a of one smaller square (with side 1 mm) is:
a=1 mm×1 mm=1 mm2
Step 5: Relate the areas
Since p smaller squares make up the area of the larger square, we can express this relationship as:
Area of larger square=p×Area of one smaller square
This gives us the equation:
100 mm2=p×1 mm2
Step 6: Solve for p
To find p, we can rearrange the equation:
p=100 mm21 mm2=100
Conclusion
Thus, the value of p is 100.
We know that:
- The side of the larger square is 1 cm.
- The side of each smaller square is 1 mm.
Since we want to compare the areas, we need to convert the side of the larger square from centimeters to millimeters.
- 1 cm = 10 mm.
The area A of the larger square can be calculated using the formula for the area of a square, which is:
A=side×side
So,
A=10 mm×10 mm=100 mm2
The area a of one smaller square (with side 1 mm) is:
a=1 mm×1 mm=1 mm2
Since p smaller squares make up the area of the larger square, we can express this relationship as:
Area of larger square=p×Area of one smaller square
This gives us the equation:
100 mm2=p×1 mm2
To find p, we can rearrange the equation:
p=100 mm21 mm2=100
Thus, the value of p is 100.
Topper's Solved these Questions
- PERIMETER AND AREABOOK - NCERT EXEMPLARCHAPTER - PERIMETER AND AREAEXERCISE - Exercise (Fill in the blanks)
19 Videos
- PERIMETER AND AREABOOK - NCERT EXEMPLARCHAPTER - PERIMETER AND AREAEXERCISE - Exercise (True or false)
7 Videos
- PERIMETER AND AREABOOK - NCERT EXEMPLARCHAPTER - PERIMETER AND AREAEXERCISE - Think and Discuss
7 Videos
- LINES AND ANGLES BOOK - NCERT EXEMPLARCHAPTER - LINES AND ANGLES EXERCISE - Exercise (fill in the blanks)
3 Videos
- PRACTICAL GEOMETRY SYMMETRY AND VISUALISING SOLID SHAPESBOOK - NCERT EXEMPLARCHAPTER - PRACTICAL GEOMETRY SYMMETRY AND VISUALISING SOLID SHAPESEXERCISE - EXERCISE
108 Videos
Similar Questions
Knowledge Check
The side of an equilateral triangle is equal to the diagonal of the square. If the side of the square is 10 cm, then what is the area of the equilateral triangle?
Aa. 50√2Bb. 50√3Cc. 100√2Dd. 100√3The side of an equilateral triangle is equal to the diagonal of the square. If the side of the square is 12 cm, then what is the area of the equilateral triangle?
A50√2B50√3C72√3D100√3