Cost of fencing around a square field is Rs. 12000. If the cost of fencing per metre is Rs. 30, find the area of the square field.
The correct Answer is:10000sq m
To solve the problem step by step, let's break it down:
Step 1: Understand the given information
We know the total cost of fencing around the square field is Rs. 12,000 and the cost of fencing per meter is Rs. 30.
Step 2: Calculate the perimeter of the square field
To find the perimeter, we can use the formula:
Perimeter=Total CostCost per meter
Substituting the values:
Perimeter=1200030
Step 3: Perform the division
Now, we calculate:
Perimeter=400 meters
Step 4: Relate perimeter to the side of the square
The perimeter of a square is given by the formula:
Perimeter=4×Side
Let’s denote the side of the square as s. Therefore, we can write:
4s=400
Step 5: Solve for the side of the square
To find the side, we divide both sides of the equation by 4:
s=4004
Calculating this gives:
s=100 meters
Step 6: Calculate the area of the square field
The area A of a square is given by:
A=Side×Side=s×s
Substituting the value of s:
A=100×100
Step 7: Perform the multiplication
Calculating this gives:
A=10000 square meters
Final Answer
The area of the square field is 10,000 square meters.
---
We know the total cost of fencing around the square field is Rs. 12,000 and the cost of fencing per meter is Rs. 30.
To find the perimeter, we can use the formula:
Perimeter=Total CostCost per meter
Perimeter=1200030
Now, we calculate:
Perimeter=400 meters
The perimeter of a square is given by the formula:
Perimeter=4×Side
4s=400
To find the side, we divide both sides of the equation by 4:
s=4004
s=100 meters
The area A of a square is given by:
A=Side×Side=s×s
A=100×100
Calculating this gives:
A=10000 square meters
The area of the square field is 10,000 square meters.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLARCHAPTER - MENSURATIONEXERCISE - Exercise (Multiple Choice Questions)6videos
MENSURATION
- BOOK - NCERT EXEMPLARCHAPTER - MENSURATIONEXERCISE - Exercise42videos
MENSURATION
- BOOK - NCERT EXEMPLARCHAPTER - INTEGERS EXERCISE - Exercise42videos
INTEGERS
- BOOK - NCERT EXEMPLARCHAPTER - NUMBER SYSTEMEXERCISE - Exercise49videos
NUMBER SYSTEM
Similar Questions
Knowledge Check
- Question 1 - Select One
Cost of erecting a fence round a square field of 625 hectares at 15 paise per metre is
ARs. 500BRs. 750CRs. 100DRs. 1500 - Question 2 - Select One
The cost of making a fence around a square field is Rs. 392 at the rate of Rs. 14 per metre. What is the length of each side of the field ?
A7mB8mC8 cmD7 cm - Question 3 - Select One
The cost of fencing a square field is Rs. 1976 at the rate of Rs. 26 per metre. What is the length of each side of the field?
A18 mB19 mC20 mD13 m
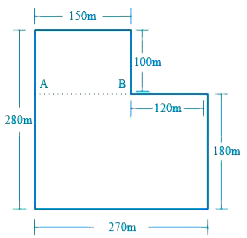
Total cost of fencing the park shown in Fig. 6.17 is Rs 55000. Find the cost of fencing per metre.
 View Solution
View SolutionA rectangular plot of land is 240 m by 200 m. The cost of fencing per meter is Rs 30. what is the cost of fencing the entire field?
View SolutionThe cost of fencing a square field at 60 paise per metre is Rs. 1200. Find the cost of reaping the field at the rate of 50 paise per 100 sq. metres.
View SolutionThe cost of fencing a circular field at the rate of Rs.24 per metre is Rs. 5280. Find the radius of the field.
View SolutionThe area of a square field isd 1444m2. Find the cost of fencing the field at the rate of Rs.5 per metre.
View Solution

