Divide the park shown in figure into two rectangles. Find the total area of this park. If one packet of fertilizer is used for 300sq m, how many packets of fertilizer are required for the whole park?
To solve the problem step by step, we will first divide the park into two rectangles, calculate the area of each rectangle, and then find the total area of the park. Finally, we will determine how many packets of fertilizer are needed for the entire area.
Step 1: Identify the dimensions of the first rectangle (A-B-C-D)
- Length (A-B): 150 meters
- Breadth (B-C): 100 meters
Step 2: Calculate the area of the first rectangle (A-B-C-D)
- Area = Length × Breadth
- Area = 150 m × 100 m = 15,000 m²
Step 3: Identify the dimensions of the second rectangle (A-E-F-G)
- Length (A-E): 270 meters (150 + 120)
- Breadth (A-F): 180 meters (280 - 100)
Step 4: Calculate the area of the second rectangle (A-E-F-G)
- Area = Length × Breadth
- Area = 270 m × 180 m = 48,600 m²
Step 5: Calculate the total area of the park
- Total Area = Area of rectangle A-B-C-D + Area of rectangle A-E-F-G
- Total Area = 15,000 m² + 48,600 m² = 63,600 m²
Step 6: Determine the number of packets of fertilizer required
- Given that one packet of fertilizer covers 300 m², we can find the number of packets needed for the total area.
- Number of packets = Total Area / Area covered by one packet
- Number of packets = 63,600 m² / 300 m² = 212 packets
Final Answer:
The total area of the park is 63,600 m², and 212 packets of fertilizer are required for the whole park.
---
- Length (A-B): 150 meters
- Breadth (B-C): 100 meters
- Area = Length × Breadth
- Area = 150 m × 100 m = 15,000 m²
- Length (A-E): 270 meters (150 + 120)
- Breadth (A-F): 180 meters (280 - 100)
- Area = Length × Breadth
- Area = 270 m × 180 m = 48,600 m²
- Total Area = Area of rectangle A-B-C-D + Area of rectangle A-E-F-G
- Total Area = 15,000 m² + 48,600 m² = 63,600 m²
- Given that one packet of fertilizer covers 300 m², we can find the number of packets needed for the total area.
- Number of packets = Total Area / Area covered by one packet
- Number of packets = 63,600 m² / 300 m² = 212 packets
The total area of the park is 63,600 m², and 212 packets of fertilizer are required for the whole park.
Topper's Solved these Questions
- MENSURATIONBOOK - NCERT EXEMPLARCHAPTER - MENSURATIONEXERCISE - Exercise (Fill in the blanks)
5 Videos
- MENSURATIONBOOK - NCERT EXEMPLARCHAPTER - MENSURATIONEXERCISE - Exercise (True or False)
7 Videos
- MENSURATIONBOOK - NCERT EXEMPLARCHAPTER - MENSURATIONEXERCISE - Exercise (Multiple Choice Questions)
6 Videos
- INTEGERS BOOK - NCERT EXEMPLARCHAPTER - INTEGERS EXERCISE - Exercise
42 Videos
- NUMBER SYSTEMBOOK - NCERT EXEMPLARCHAPTER - NUMBER SYSTEMEXERCISE - Exercise
49 Videos
Similar Questions
Knowledge Check
A railing of 288 m is required for fencing a semi-circular park. Find the area of the park.
A4928m2B9865m2C8956m2D9856m2A word and number arrangement machine when given an input line of words and numbers rearranges them following a particular rule in each step. The following is an illustration of mput and rearrangement (All the numbers given in the arrangement are two digit numbers).
Input : gone over 35 69 test 72 park 27
Step I : 27 gone over 35 69 test 72 park
Step II : 27 test gone over 35 69 72 park
Step III : 27 test 35 gone over 69 72 park
Step IV : 27 test 35 park gone over 69 72
Step V : 27 test 35 park 69 gone over 72
Step VI : 27 test 35 park 69 over gone 72
Step VI : 27 test 35 park 69 over 72 gone
and Step VII is the last step of the rearrangement of the above input as the desired arrangement is obtained.
As per the rules followed in the above steps, find out in each of the following questions the appropriate step for the given input.
Input : 86 open shut door 31 49 always 45 How many steps will be required to complete the rearrangement ?AFiveBSixCSevenDFourThere are three floors in a given building such that floors 2 is above floors 1 and floors 3 is above floors 2. in the building there are two flats in each floors such Flat M is in the west of Flat N. In the building each flat has an area of 576 ft and each flat has same number of store.
The area of each store of one of the flat on the even number floor is 64 ft. total store on floor number third is seven. the flat which has store of area of 72 ft is on odd number floor. area of each store stay in the flat which is exactly below 9 store in flat is 288 ft. there is only one floor between the flat who's each room area is 192 ft and the flat whose area is 72 ft. there is no man on the west of the flat having 6 childrens.
What is the total number of store on floor 2nd ?Aa) 6Bb) 4Cc) 3Dd) 8
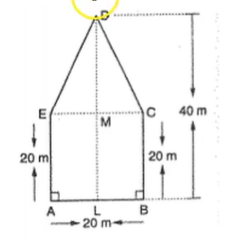
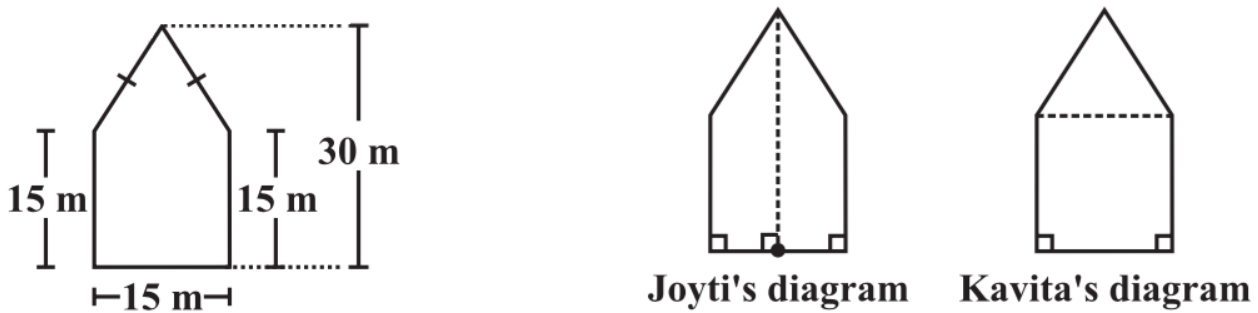
There is a pentagonal shaped park as shown in the figure. For finding its area Jyoti and Kavita divided it in two different ways. Find the area of this park using both ways. Can you suggest some other way of finding its area ?
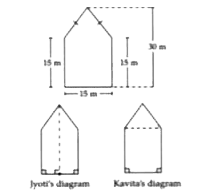
View SolutionThere is a pentagonal shaped park as shown in the figure. For finding its area Jyoti and Kavita divided it in two different ways.Find the area of this park using both ways. Can you suggest some other way of finding its area?
View SolutionA gardener has a supply of fertilizer of type I which consists of 10% nitrogen and 6% phosphoric acid and type II fertilizer which consists of 5% nitrogen and 10% phosphoric acid. After testing the soil conditions, he finds that he needs at least 14 kg of nitrogen and 14 kg of phosphoric acid for his crop. If the type I fertilizer costs 60 paise per kg and type II fertilizer costs 40 paise per kg, determine how many kilograms of each fertilizer should be used, so that nutrient requirements are met at a minimum cost. What is the minimum cost?
View SolutionThe length of a chain used as the boundary of a semicircular park is 108m. Find the area of the park.
View SolutionTwo opposite sides of a rectangle are each divided into n congruent segments, and the endpoints of segment are joined to the center to form triangle A. The other sides are each divided into m consegments, and the endpoints of one of these segments are joined to the center to form triangle B figure for n = 5, m= 7.) What is the ratio of the area of triangle A to the area of triangle B?
View Solution