57 sweets were distributed among 10 children such that each girl got 6 sweets and each boy got 5 sweets. What is the number of boys ?
10 बच्चों में कुल 57 मिठाइयाँ इस प्रकार बांटी गई कि प्रत्येक लड़की को 6 मिठाइयाँ और प्रत्येक लड़के को 5 मिठाइयाँ मिली | लड़कों कि संख्या है :
3
6
4
5
To solve the problem, we need to determine the number of boys among the 10 children given that each girl receives 6 sweets and each boy receives 5 sweets, with a total of 57 sweets distributed.
1. Define Variables:
Let the number of girls be g and the number of boys be b.
We know that:
g+b=10(1)
2. Set Up the Sweets Equation:
Each girl receives 6 sweets, and each boy receives 5 sweets. The total number of sweets distributed is 57. Therefore, we can write:
6g+5b=57(2)
3. Substitute from Equation (1):
From equation (1), we can express g in terms of b:
g=10−b
Now substitute g in equation (2):
6(10−b)+5b=57
4. Simplify the Equation:
Expanding the equation gives:
60−6b+5b=57
Combine like terms:
60−b=57
5. Solve for b:
Rearranging the equation gives:
−b=57−60
−b=−3
b=3
6. Find the Number of Girls:
Substitute b back into equation (1) to find g:
g+3=10⟹g=10−3=7
7. Conclusion:
The number of boys is 3.
Let the number of girls be g and the number of boys be b.
We know that:
g+b=10(1)
Each girl receives 6 sweets, and each boy receives 5 sweets. The total number of sweets distributed is 57. Therefore, we can write:
6g+5b=57(2)
From equation (1), we can express g in terms of b:
g=10−b
Now substitute g in equation (2):
6(10−b)+5b=57
Expanding the equation gives:
60−6b+5b=57
Combine like terms:
60−b=57
Rearranging the equation gives:
−b=57−60
−b=−3
b=3
Substitute b back into equation (1) to find g:
g+3=10⟹g=10−3=7
The number of boys is 3.
Topper's Solved these Questions
Similar Questions
The ratio of boys and girls in a college was 4 : 5. New students got admitted and the number of boys went up by 50% and the number of girls went up by 60%. What is the new ratio of boys and girls in the college?
एक कॉलेज में लड़कों तथा लड़कियों की संख्या में 4 : 5 का अनुपात है | नए छात्रों का नामांकन हुआ और लड़कों की संख्या 50% तथा लड़कियों की संख्या 60% बढ़ गयी | इस कॉलेज में लड़कों और लड़कियों का नया अनुपात क्या है ?
View SolutionRs. 10,000 has to be distributed among 3 craftsmen, 5 helpers and 6 labourers such that each helper receives the amount twice as much as a labourer receives and each craftsman receives the amount thrice as much as a labourer receives. What is the amount received by the three craftsmen ?
10000 रुपये का वितरण 3 शिल्पकारों, 5 सहायकों तथा 6 श्रमिकों के बीच इस प्रकार करना है कि प्रत्येक सहायक को मिलने वाली राशि एक श्रमिक को मिलने वाली राशि से दोगुनी हो तथा प्रत्येक शिल्पकार को मिलने वाली राशि एक श्रमिक को मिलने वाली राशि से तिगुनी हो | तीनों शिल्पकारों द्वारा प्राप्त की गयी राशि ज्ञात करें |
View Solution
Knowledge Check
A total of 57 sweets were distributed among 10 children such that each girl gets 6 sweets and each boy gets 5 sweets. The number of boys is :
A6B4C3D5
Rs 820 is divided among 6 men, 8 women and 12 boys in such a way that every woman gets an amount equal to that received by one man and one boy combined and that every man gets one and a half times the amount received by a boy. What is the total amount received by 8 women?
820 रुपये को 6 पुरुषों, 8 महिलाओं तथा 12 लड़कों के बीच इस प्रकार विभाजित किया जाता है कि प्रत्येक महिला को एक पुरुष तथा एक लड़के को मिली संयुक्त राशि के बराबर राशि मिलती है तथा प्रत्येक पुरुष को एक लड़के को मिली राशि से डेढ़ गुना राशि मिलती है| 8 महिलाओं को मिली कुल राशि ज्ञात करें |
View Solutionfirst graph shows the number of students (boys and girls in thousands) in Grade 1 to Grade 5.
पहला आरेख ग्रेड 1 से लेकर ग्रेड 5 तक छात्रों की संख्या (लड़के और लड़कियाँ, हज़ार में) को दर्शाता है।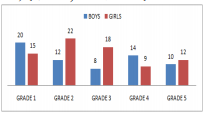 And the bar graph below shows the percentage share of five schools in the total students studying in that class.
And the bar graph below shows the percentage share of five schools in the total students studying in that class.
और नीचे दिया गया दंड आरेख उस कक्षा में पढ़ रहे कुल छात्रों में पाँच विद्यालयों की प्रतिशत हिस्सेदारी को दर्शाता है।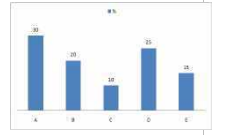 Based on the information, if the boys to girls ratio in school D is 3:1, then the number of boys studying in school D is:
Based on the information, if the boys to girls ratio in school D is 3:1, then the number of boys studying in school D is:
दी गयी जानकारी के आधार पर, यदि विद्यालय D में लड़कों और लड़कियों की संख्या का अनुपात 3:1 है, तो विद्यालय D में पढ़ने वाले लड़कों की | संख्या कितनी है?
View SolutionA total of 60 students are travelling in a bus. The ratio of the number of boys to that of girls is 2 : 1. Then, 15 boys get down and 5 girls get in the bus at the first stop, and 5 boys get in and 10 girls get down from the bus at the second stop. What is the ratio of the number of boys to that of girls in the bus after the second stop?
एक बस में कुल 60 छात्र यात्रा कर रहे हैं। लड़कों तथा लड़कियों की संख्या का अनुपात 2:1 है। फिर, पहले बस स्टॉप पर 15 लड़के उतर जाते हैं तथा 5 लडकियाँ चढ़ती हैं। अगले बस स्टॉप पर 5 लड़के चढ़ते हैं और 10 लड़कियाँ उतर जाती हैं। दूसरे स्टॉप के बाद बस में लड़कों तथा लड़कियों की संख्या का क्या अनुपात है?
View SolutionThe average of some numbers is 54.6. If 75% of the numbers are increased by 5.6 each and the rest are decreased by 8.4 each, then what is the average of the numbers so obtained?
कुछ संख्याओं का औसत 54.6 है | यदि इन संख्याओं के 75% में से प्रत्येक को 5.6 से बढ़ा दिया जाए तथा शेष में से प्रत्येक को 8.4 से कम कर दिया जाए, तो इस प्रकार प्राप्त होने वाली संख्याओं का औसत क्या होगा ?
View SolutionThe ratio of boys and girls in a group is 7:6. If 4 more boys join the group and 3 girls leave the group, then the ratio of boys to girls becomes 4:3. What is the total number of boys and girls initially in the group?
एक समूह में लड़कों तथा लड़कियों का अनुपात 7 : 6 है | यदि 4 और लड़के समूह में आ जाते हैं तथा 3 लड़कियाँ समूह से चली जाती हैं, तो लड़कों तथा लड़कियों का अनुपात 4: 3 हो जाता है | आरंभ में इस समूह में लड़कों तथा लड़कियों की कुल संख्या कितनी थी ?
View SolutionThe average age of 120 students in a group is 13.56 years, 35% of the number of students are girls and the rest are boys. If the ratio of the average age of boys and girls is 6:5, then what is the average age (in years) of the girls?
एक समूह के 120 छात्रों की औसत उम्र 13.56 वर्ष है | छात्रों में 35% लड़कियाँ हैं तथा शेष लड़के हैं | यदि लड़कों और लड़कियों की औसत उम्र का अनुपात 6 : 5 है, तो लड़कियों की औसत उम्र ( वर्ष में ) कितनी है ?
View SolutionIn a school there are 550 students. The ratio of the boys to that of the girls is 6 : 5. How many more girls should join the school so that the ratio becomes 5 : 6?
एक विद्यालय में, 550 छात्र हैं | लड़कों और लड़कियों की संख्या में 6:5 का जनुपात है | इस अनुपात को 5 : 6 करने के लिए कितनी अतिरिक्त लड़कियों को विद्यालय में दाखिला लेना चाहिए ?
View Solution
