A rectangular lawn whose length is twice of its breadth is extended by having four semi-circular portions on its sides. What is the total area (in m2) of the lawn if the smaller side
of the rectangle is 12m? (Takeπ = 3.14)
एक आयताकार लॉन जिसकी लंबाई इसकी चौड़ाई से दोगुनी है, उसके किनारों पर चार अर्ध-वत्ताकार हिस्से होते हैं। यदि आयत की चौड़ाई 12 मीटर है तो लॉन का कुल क्षेत्रफल (m2 ) में क्या है? (मानिए π= 3.14)
444
548.32
308.64
853.2
To find the total area of the rectangular lawn with semi-circular portions, we will follow these steps:
Step 1: Determine the dimensions of the rectangle
Given that the breadth of the rectangle is 12 m, and the length is twice the breadth:
- Breadth (b) = 12 m
- Length (l) = 2 × breadth = 2 × 12 = 24 m
Step 2: Calculate the area of the rectangle
The area A of a rectangle is calculated using the formula:
A=Length×Breadth
Substituting the values we found:
A=24m×12m=288m2
Step 3: Determine the radii of the semi-circles
The rectangle has four semi-circular portions:
- The smaller semi-circles are on the shorter sides (breadth) of the rectangle.
- The diameter of each smaller semi-circle is equal to the breadth of the rectangle, which is 12 m.
- Therefore, the radius r of the smaller semi-circle is:
r=122=6m
- The larger semi-circles are on the longer sides (length) of the rectangle.
- The diameter of each larger semi-circle is equal to the length of the rectangle, which is 24 m.
- Therefore, the radius R of the larger semi-circle is:
R=242=12m
Step 4: Calculate the area of the semi-circles
The area of a semi-circle is given by the formula:
Area of semi-circle=12πr2
- Area of the two smaller semi-circles:
Areasmall=2×12π(6)2=π(62)=π×36
Substituting π=3.14:
Areasmall=3.14×36=113.04m2
- Area of the two larger semi-circles:
Arealarge=2×12π(12)2=π(122)=π×144
Substituting π=3.14:
Arealarge=3.14×144=452.16m2
Step 5: Calculate the total area
Now, we can find the total area of the lawn by adding the area of the rectangle and the areas of the semi-circles:
Total Area=Area of Rectangle+Area of Semi-circles
Total Area=288m2+113.04m2+452.16m2
Total Area=288+113.04+452.16=853.2m2
Final Answer
The total area of the lawn is 853.2 m².
---
Given that the breadth of the rectangle is 12 m, and the length is twice the breadth:
- Breadth (b) = 12 m
- Length (l) = 2 × breadth = 2 × 12 = 24 m
The area A of a rectangle is calculated using the formula:
A=Length×Breadth
Substituting the values we found:
A=24m×12m=288m2
The rectangle has four semi-circular portions:
- The smaller semi-circles are on the shorter sides (breadth) of the rectangle.
- The diameter of each smaller semi-circle is equal to the breadth of the rectangle, which is 12 m.
- Therefore, the radius r of the smaller semi-circle is:
r=122=6m
- The diameter of each larger semi-circle is equal to the length of the rectangle, which is 24 m.
- Therefore, the radius R of the larger semi-circle is:
R=242=12m
The area of a semi-circle is given by the formula:
Area of semi-circle=12πr2
Areasmall=2×12π(6)2=π(62)=π×36
Substituting π=3.14:
Areasmall=3.14×36=113.04m2
Arealarge=2×12π(12)2=π(122)=π×144
Substituting π=3.14:
Arealarge=3.14×144=452.16m2
Now, we can find the total area of the lawn by adding the area of the rectangle and the areas of the semi-circles:
Total Area=Area of Rectangle+Area of Semi-circles
Total Area=288m2+113.04m2+452.16m2
Total Area=288+113.04+452.16=853.2m2
The total area of the lawn is 853.2 m².
Topper's Solved these Questions
Similar Questions
A rectangular lawn whose length is twice of its breadth is extended by having four semi-circular portions on its sides. What is the total area (in m2) of the lawn if the smaller side of the rectangle is 12m? (Takeπ = 3.14)
एक आयताकार लॉन जिसकी लंबाई इसकी चौड़ाई से दोगुनी है, उसके किनारों पर चार अर्ध-वत्ताकार हिस्से होते हैं। यदि आयत की चौड़ाई 12 मीटर है तो लॉन का कुल क्षेत्रफल (m2 ) में क्या है? (मानिए π= 3.14)
View SolutionThe area of a rectangle is 126m2 and its length is 12m. The breadth of the rectangle is
View Solution
Knowledge Check
A rectangular lawn whose length is one-half times of its breadth. The area of the law is 23 hectares. The length of the lawn is:
A100 metresB3313 metresC6623 metresD(100√3) metresA playground is in the form of a rectangle having semicircle on the shorter sides. Find its area when the length of the rectangular portion is 80 m and the breadth is 42 m.
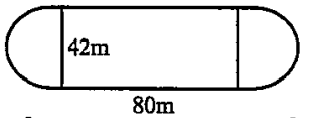 A4746m2B4748m2C4950m2D5049m2
A4746m2B4748m2C4950m2D5049m2
The length of a rectangle plot is five times of its breadth. If the area of the rectangular plot is 2000 m2 ,then what is the breadth of the rectangular plot?
एक आयताकार भूखंड की लंबाई इसकी चौड़ाई का पांच गुना है। यदि आयताकार भूखंड का क्षेत्रफल 2000 m2, है, तो आयताकार भूखंड की चौड़ाई क्या है?
View SolutionA rectangular grassy lawn is 18 m by 12 m. It has a gravel path 1.5 m wide all around it on the outside. What is the area of the path .
View SolutionThe breadth of a rectangle is four times of its length. If the area of the rectangle is 1764, then what is the breadth of the rectangle?
एक आयत की चौड़ाई इसकी लंबाई का चार गुना है। यदि आयत का क्षेत्रफल 1764 है, तो आयत की चौड़ाई क्या है?
View SolutionLength and breadth of rectangular field are in the ratio 5 : 2. If the perimeter of the field is 238 m. Find the length of the field.
एक आयताकार मैदान की लंबाई और चौड़ाई 5 : 2 के अनुपात में है। यदि मैदान का परिमाप 238 मीटर है, तो मैदान की लंबाई ज्ञात कीजिए।
View SolutionThe length of a rectangular park is 20m more than its breadth. If the cost of fencing the park at Rs. 53 per metre is Rs. 21,200, then what is the area (In square metres) of the park?
एक आयताकार पार्क की लंबाई इसकी चौड़ाई से 20 मीटर अधिक है। यदि 53 रु प्रति मीटर की दर से पार्क में बाड़ लगाने की लागत 21,200 है। तो पार्क का क्षेत्रफल (वर्ग मीटर में) क्या है?
View SolutionA lawn is in the form of a rectangle having its sides in the ratio 2:3. The area of the lawn is 16 hectares. Find the length and breadth of the lawn.
View Solution