Two particles of masses m, and m, separated by a distance dare at rest initially. If they move towards each other under mutual interaction (say electric, gravitational or elastic), where will they meet?
At the centre of the joining the two particles
Anywhere in between two masses
At the centre of mass of the system of two particles
None of the above
The correct Answer is:C
Initially, the particles are at fest, so the velocity of the centre of mass,
→vCM=m1×0+m2×0m1+m2=0, As here →Fext=0 so , →vCM = constant
ie, m1→v1+m2→v2m1+m2=0 (at all instants) or m1+→m1→v1+m2→v2=0
or mtΔ→r1Δt+m2Δ→r2Δt=0
m1Δ→r1+m2Δ→r2=0 (asΔt≠∞)
m1→d1+m2→d2=0 (with Δ→r=→d)
m1d1−m2d2=0 (as direction →d2 is opposite to d1 ) or m1d1=m2d2
But given that d1+d2=d so that d1=m2dm1+m2andd2=m1dm1+m2
Now, as d1andd2 represent the position of the centre of the mass relative to m1andm2 respectively the particles will collide at the centre of mass of the system,
Similar Questions
Consider a system of two particles of masses m1 and m2 separated by a distance r. Suppose they start to move towards each other due to their mutual attraction (attractive force may be electrical, gravitational, etc.).
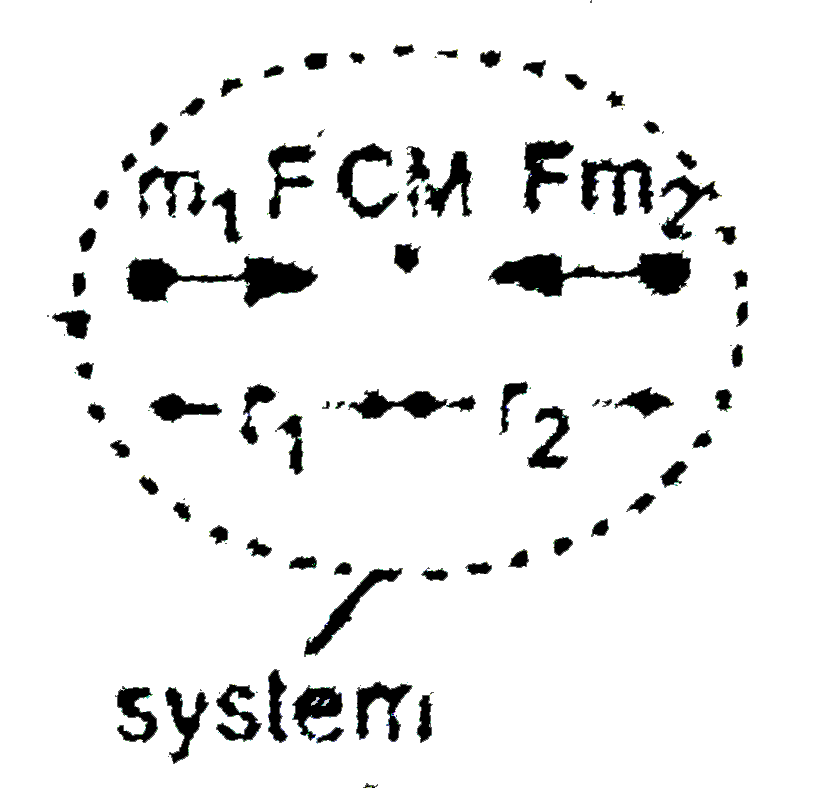
View SolutionWhen two blocks connected by a spring move towards each other under mutual interaction
View Solution
Knowledge Check
When two blocks connected by a spring move towards each other under mutual interaction
A(a) their velocities are equalB(b) their accelerations are equalC(c) the force acting on them are equal and oppositeD(d) All of the aboveWhen two blocks connected by a spring move towards each other under mutual interaction,
A(a) Their velocities are equal and oppositeB(b) Their accelerations are equal and oppositeC(c) The forces acting on them are equal and oppositeD(d) Their momenta are equal and oppositeTwo particles of masses 4kg and 6kgareseparatedbyadistanceof20cm and are moving towards each other under mutual force of attraction, the position of the point where they meet is
A12mfrom 4kg bodyB12m from 6kg bodyC8m from 4kg bodyD10m from 4kg body
When two blocks connected by a spring move towards each other under mutual interaction,
View SolutionTwo particles of masses 2 kg and 3 kg are at rest separated by 10 m. If they move towards each other under mutual force of attraction, the position of the point where they meet is
View SolutionTwo particles of masses 4 kg and 6 kg are at rest separated by 20 m. If they move towards each other under mutual force of attraction, the position of the point where they meet is
View SolutionTwo objects of masses m and 4m are at rest at an infinite separation. They move towards each other under mutual gravitational attraction. If G is the universal gravitaitonal constant, then at separation r
View SolutionTwo objects of masses m and 4m are at rest at an infinite separation. They move towards each other under mutual gravitational attraction. If G is the universal gravitaitonal constant, then at separation r
View Solution
